Journal of Oceanography and Marine Research
Open Access
ISSN: 2572-3103
+44 1300 500008
ISSN: 2572-3103
+44 1300 500008
Research Article - (2024)Volume 12, Issue 2
An intensive field study on near-shore infra-gravity wave motions was carried out to ascertain its oscillation patterns and effects on shoreline morphodynamics at the downdrift shoreline, east of Qua-Iboe River estuary, South-East coast of Nigeria. Time-series measurements of wave run-up swash periods over a neap-spring tidal cycle and daily beach profile surveys were made at the shoreline. Detailed analysis of the result revealed a dominance of gravity waves over infra-gravity wave oscillations in the wave spectra with average percentage frequencies of occurrence of 52.28 and 47.72 respectively. The infra-gravity wave spectrum is further filtered into first harmonic group (121 s-150 s), second harmonic group (91 s-120 s), third Harmonic group (61 s-90 s) and fourth harmonic group (31-60s) of oscillation periods. The first harmonic group of infra-gravity waves were noted as the principal agents of erosion and sedimentation on the beach. In addition, the first harmonic group scour sediment and trigger-off erosion on the shoreline while the short period harmonics of infra-gravity waves transport, spread, winnow and sort sediments accordingly. The gravity waves are responsible for compaction of sediment. Moreover, the oscillation pattern of the infra-gravity waves in the dissipative surf-zone shows regular and harmonic motions for which a full wavelength is propagated at every 2-3 minutes interval as the beat, and independent of tidal stage (flood or ebb). It also plays significant role in the self-cleansing potential of the surf-zone. An understanding of the energy radiation and transmission by the fourth harmonic group of infra-gravity wave oscillations is considered essential in the engineering design and management of shoreline protection, marinas and sea ports.
Infra-gravity waves; Oscillation patterns; Shoreline; Erosion and sedimentation
Infra-gravity surface waves are generated by nonlinear interactions of gravity /wind wave or swells and have periods which ranged from 20 s-200 s. Also, it can be tidally-modulated as observed in the inner shelf and in the regional seismic record which could contribute to the earth’s background free oscillations. Posited infra-gravity waves as waves types with periods ranging from 25 s-30 s to 300 s-600 s, frequencies between 0.003 Hz-0.004 Hz and wavelengths between 100 m and 10 km. They occur both in the deep and coastal oceans and are observed to be strongest near the shoreline where tidal variations of surf-zone width, beach slope and profile might affect the generation, energy dissipation, reflection or refraction.
Infra-gravity waves induce cells circulations through generation of longshore and cross-shore currents, and transport sediment on the shoreline and surf-zone.
The formation of crescentic sand bars on a beach can be attributed to standing infra-gravity edge waves in which infragravity energy must be in the form of a narrow spectral peak with one edge wave mode. Several field studies in the past such as Short and Wright, et al. had attempted to establish the relationship between infra-gravity waves and beach morphology but unable to identify the forms of infra-gravity energy. However, Guza and Inman, delineated beach morphodynamic states, based on surf-scaling parameter (€) as the breaker zone traverses different bed gradients, into reflective, intermediate and dissipative; thus:
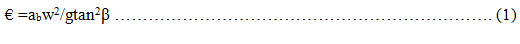
Where ab is the breaker amplitude, w=radian frequency (2π/T) and β=local gradient, g=acceleration due to gravity.
Guza and Inman posited that low values of € (€=1.0-2.5) define reflective conditions favourable for the occurrence of standing wave frequency at sub-harmonic frequencies (one half of incident wave frequency) together with sub-harmonic edge waves. On the other hand, fully dissipative beaches are characterised by € values ranged 102-103. Equation (1) is applied to this study as a basis for determination of the energy state of the study area.
When infra-gravity waves break at the shorelines; varying degrees of sediment perturbations and transport by cross-shore and longshore currents initiated by the breaking waves occur. These cause sediment perturbation and transport on the beach which result in erosion and accretion with morphological adjustment of the beach into beach cusps, log-spiral crenulate, embayment and intertidal bars, etc.
Shoreline erosion is a global problem attributable to impact of waves on the shoreline. The dynamics of shoreline erosion reveals that accretion processes occur simultaneously with erosion but the net volumetric change of sediment transport into and out of littoral cells on the shoreline defines the morpho-dynamic status of the shoreline. The net product of shoreline morphodynamics in recent past along Nigerian coastline is shoreline erosion which resulted in shoreline retreat. This can be attributed to changes in atmospheric and ocean dynamics such as global sea level rise and climate change. These phenomena accentuated hydrodynamic processes to the extreme, such as storm surges with the attendant negative impact on the shoreline which include shoreline erosion, coastal submergence, threat to integrity of coastal infrastructure and installations, etc. Previous works on coastal erosion along Nigerian coastline by several authors have indicated that shoreline erosion is caused by the actions of wave swash and backwash and long-shore current transports on beaches.This trend if allow unabated will result in loss of lives, property and territorial land to the sea. Therefore, the need to isolate and investigate on the fundamental component of the wave processes which is considered as the principal agent of erosion dynamics along the shoreline is imperative [1]. So, the focus of this investigation is to analyse the dynamics and effects of infragravity oscillations in the wave spectrum as one of the remote factor of shoreline erosion/sedimentation and the implications for coastal engineering project on the strand coast of Ibeno beach, East of Qua-Iboe River Estuary, South East Coast of Nigeria.
Study area
The down-drift shoreline adjoining Qua-Iboe River Estuary is located in Ibeno Local Government Area of Akwa Ibom State,South-East coast of Nigeria (Figure 1).
The shoreline is a mesotidal tropical sandy beach exposed to semi-diurnal tides and south-westerly waves with amplitude less than 20 cm, which is associated with south-westerly wind conditions which vary annually from calm (November- February) through transitional (February-April) and storm ( May-October). Modal wave periods close to the shore are 8 s-12 s. Long-shore current velocities along the ocean shoreline ranged 50 cm/s-125 cm/s east with periodic reversals to the west at the downdrift beach contiguous to the estuary mouth due to changes in tidal stage. The shoreline represents an exposed section of the abandoned beach ridges laterally bounded by mangrove swamps of the lower deltaic plain of Holocene age. It is underlain by Sombreiro-Warri deltaic plain sand of late Pleistocene. The beach is texturally homogeneous with predominantly well sorted fined-grained sand.
The study covered a period of seven days from August 24 (neap tide) through 30 (spring tide) in 2015. The monitoring station for this study was at 500 m location which is station 2 away from the estuary mouth and which is also one of my permanent research locations, downdrift of the Qua Iboe River estuary (Figure 1). Wave run-up of wave swash oscillations times were recorded for each successive wave swash as it hits each of a series of range poles strategically fixed at 10 m spacing intervals across the beach foreshore for a 12-hourly tidal cycle. Each recorded time was subtracted from the preceding one to obtain the wave run-up period. The above method is an improvised technique inspired from previous work such as Hollman and Guza. The wave run-up periods were grouped into 10-class intervals and their percentage frequencies determined per hour over a 12 hour tidal cycle. Swash lengths were estimated based on the length of the beach foreshore relative to the range poles as the distance of the wave swash from the breaking point to the wave swash run-up height at every fifteen minutes [2]. Other hydrodynamic parameters such as Wave breaker height and water depth were measured, at each of the four monitoring stations along the ocean shoreline, with the aid of a graduated staff. It was measured by placing the tip of the graduated staff on the water surface as the wave advanced to the beach and read off the height of the crest at breaking. The corresponding water depth was also measured immediately after the wave has broken by placing the tip of the staff to the bottom of the surf zone at the wave breaker point. Long-shore current velocities were also monitored at quarter-hourly intervals as a time taken by a buoyant object to freely drift over a known distance. Daily beach profiles were made at the station over a neap-spring tidal phase, while linear beach profile measurements were converted to beach volumetric change within a one-metre wide transect. At the monitoring station geomorphic beach segments were demarcated into: backshore, upper, mid and lower foreshore.
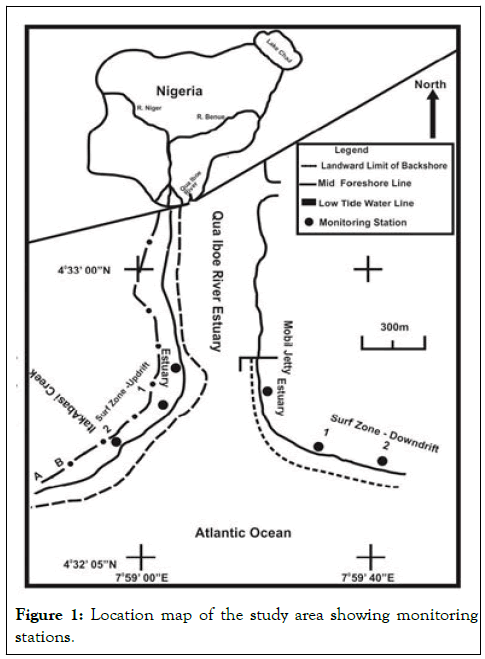
Figure 1: Location map of the study area showing monitoring stations.
Wave parameters
Results of wave run-up periods as shown in Figures 2 and 3, reveal that gravity and infra-gravity waves oscillations periods are inversely related with the percentage frequencies of occurrences. The lower the oscillation period the higher the percentage frequency of occurrence, and the higher the oscillation period the lower the percentage frequency of occurrence. Figures 4 and 5 show that gravity and infra-gravity waves oscillations are independent of tidal stage, flood or ebb. However, Figures 6 and 7 differentiate the wave spectra of the area into gravity wave (0 s-30 s periods) and infra-gravity waves (30 s-150 s) periods with 52.28% and 47.72% frequencies of occurrence respectively. The above finding corroborates the classification of ocean wave spectrum according to wave periods by Munk and is also consistent within the range of classifications of infra-gravity [2]. The infra-gravity wave spectrum (47.72%) is further filtered into group intervals of thirty seconds (30 s) periods range defined as ‘harmonics’ thus: First harmonics(121 s-150 s), second harmonics (91 s-120 s), Third harmonics (61 s-90 s) and fourth harmonics (31 s-60 s), while their corresponding average percentage frequencies of occurrences are 24.68,12.33,7.51 and 3.20 respectively.
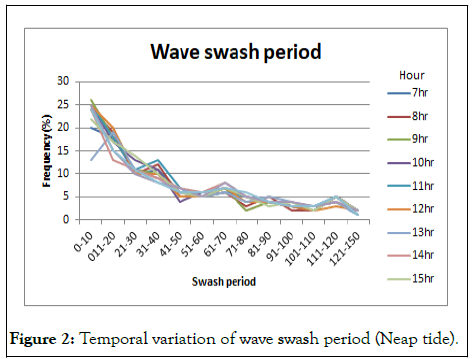
Figure 2: Temporal variation of wave swash period (Neap tide).
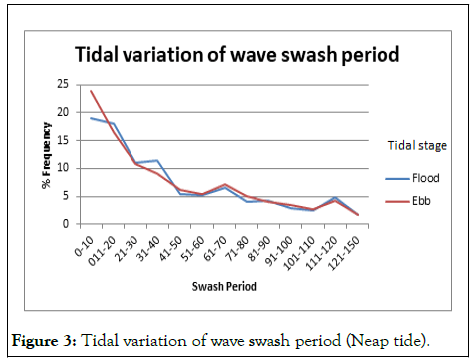
Figure 3: Tidal variation of wave swash period (Neap tide).

Figure 4: Analysis of temporal variation of wave swash period (Neap tide).
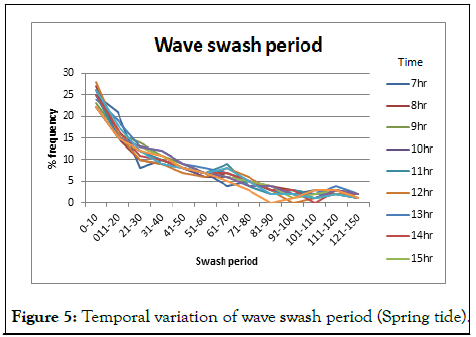
Figure 5: Temporal variation of wave swash period (Spring tide).
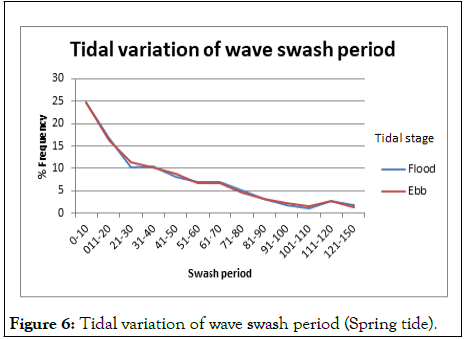
Figure 6: Tidal variation of wave swash period (Spring tide).
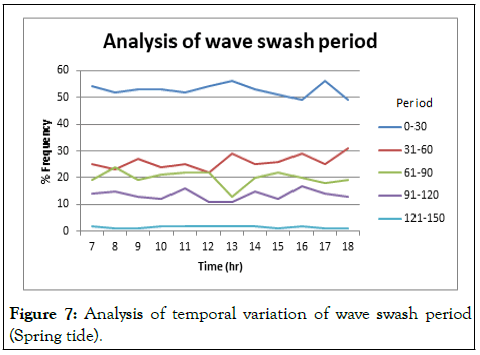
Figure 7: Analysis of temporal variation of wave swash period (Spring tide).
Other wave breaker parameters recorded during the study period reflect the dissipative characteristics of the surf-zone. These include wave-breaker height and water depth which increased from 10 cm at low tide to a maximum value of 80 cm at high water during neap and spring tides (Figures 8-12). Surfscaling parameters of 200 and 350 were noted at neap and spring tide respectively (Figure 10). Wave swash length varies from 5 m-10 m at low tide to 50 m-70 m at high tide and was strongly influenced by increase in wave- breaker height and water depth and the gentle beach slope which ranged 2°-4°.
By implication, infra-gravity waves oscillations energy increases with rising tide from low to high tide and from neap to spring tide [3]. The percentage occurrence of spilling wave breakers was 60% over 40% of plunging breakers. Maximum longshore current velocities recorded were 50 cm/s and 80 cm/s due east with some westward flow reversals at neap and spring tides respectively (Figure 9).
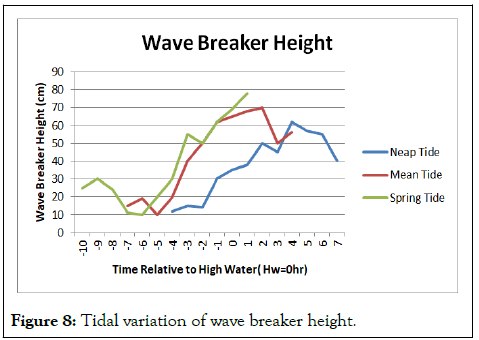
Figure 8: Tidal variation of wave breaker height.
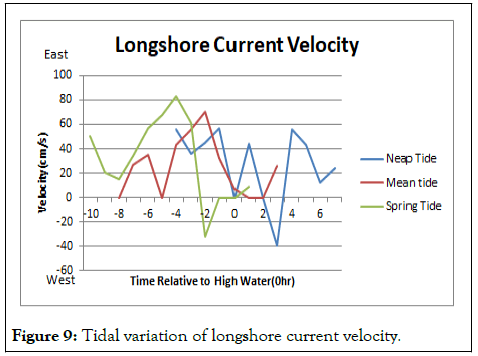
Figure 9: Tidal variation of longshore current velocity.
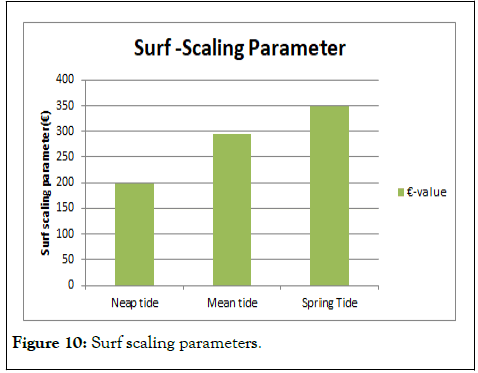
Figure 10: Surf scaling parameters.
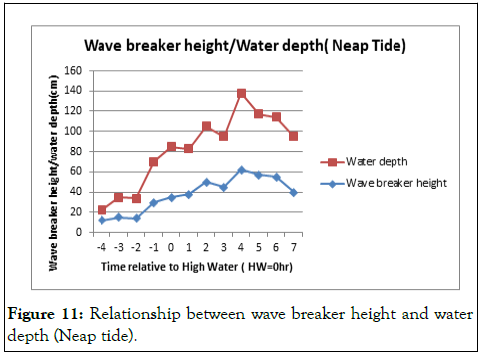
Figure 11: Relationship between wave breaker height and water depth (Neap tide).
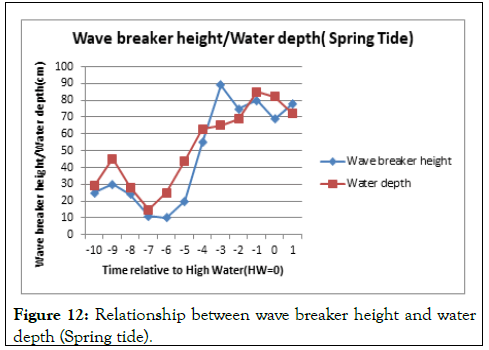
Figure 12: Relationship between wave breaker height and water depth (Spring tide).
A daily beach profile plots which depict concave but gentle gradient (2-50) [4-6]. A computation of beach sediment volumetric change between neap and spring tides during the study period resulted in sediment deficit of -380 m3 as the net which indicate erosion.
Effects of infra-gravity waves oscillations on the shoreline
Effects of infra-gravity wave oscillations on the shoreline are numerous but for the purpose of this investigation, the discussion below is made in respect of wave dynamics, beach erosion and sedimentation.
Infra-gravity wave dynamics
Infra-gravity wave spectrum of the study area is examined based on four groups of harmonic periods, trend of impact on the shoreline and dominant wave processes.
The first harmonic infra-gravity waves group (121 s-150 s): This group of infra-gravity waves is the first to advance, inundate, and impact the beach in every 2.5 to 3 minutes intervals. The has the lowest percentage frequency of occurrence (3.20%) in the wave spectra, characterised by significant wave- breaker height with the corresponding water depth, traverse the longest wave run-up distance and swash length, scour and trigger-off crossshore sediment movement on the beach at the highest degree than other low period harmonics [7]. However, this harmonic tends to propel, with the highest force, energized by significant wave-breaker height and water depth, any floating object in the surf-zone to the shoreline than other low harmonics. Therefore, the terminal effect of energy radiation and transmission by the fourth harmonics infra-gravity wave on the shoreline, through the above processes, could also be a factor for seiches and harbour oscillations which affect anchorage and moorings of marine vessels and other facilities in marinas.
The second harmonic infra-gravity waves group (91 s-120 s): This harmonic is the second group of infra-gravity waves with 7.51% frequency of occurrence to impact the shoreline. It performs an intermediary and a transitory role in effect between the first and the third harmonics infra-gravity waves groups [8]. These include superposition and wedging of backwash from the impact of the first harmonic infra-gravity waves producing periodic erosional perturbation on the beach.
The third harmonics infra-gravity waves group (61 s-90 s): The third harmonic is the third infra-gravity waves group with 12.33% frequency of occurrence to impact on the shoreline. It superposes on wave swashes of the first and second harmonic infra-gravity Waves and disperses sediment in the area [9]. The second harmonic infrgravity Waves transport, distribute and spread sediment on the beach by the processes of long-shore and cross-shore currents.
The fourth harmonics (31 s-60 s): This group of infra-gravity waves with 24.68% frequency of occurrence is the fourth to impact the beach and the lowest periods in the infra-gravity waves spectrum. Similar to the third harmonic, the fourth harmonic infra-gravity waves perform an intermediary and transitory role between the third harmonic infra-gravity waves and gravity waves oscillations (0 s-30 s). The gravity waves, with 52.28% frequency of occurrence in the wave spectra, sort, winnow, compress and compact sediment, generating bed forms by the effect of their oscillatory and turbulent motions on the beach.
The above analysis as was also noted in the field depicts a trend of incident wave group comprises of gravity waves, first, second, third and fourth harmonics of infra-gravity waves oscillations which traverse the beach in sequence as a group at every 2-3 minutes intervals [10]. The wave trend is supposed to be a wavelength.
Beach erosion and sedimentation
In Figure 13, net sedimentation patterns at the monitoring station fluctuated between -1.32 m3 and -3.51 m3 at neap and spring tide respectively indicative of erosion as depicted by the concave beach profile plots. The first harmonic infra-gravity waves erode sediment from the beach, as the net product between wave swash and backwash actions, and transport seaward through backwash when the surf-zone is deficient of sediment supply [11]. But when the surf-zone has surplus of sediment, the net product of wave swash-backwash actions on the beach is accretion through swash action. Depending on the sediment budget in the surf-zone, as the maximum limit of wave swash action at the end of every 24-hour semi-diurnal tidal cycle migrate to the last limit at spring tide towards the land, the erosion/accretion effect of the first harmonics, increases with increase in the gravitational pull on the surface of water. Also, as the maximum limit of wave swash action migrate seaward to the last limit at neap tide, the erosion/ accretion effect of the first harmonics decreases with decrease in gravitational pull on the surface of water [12].
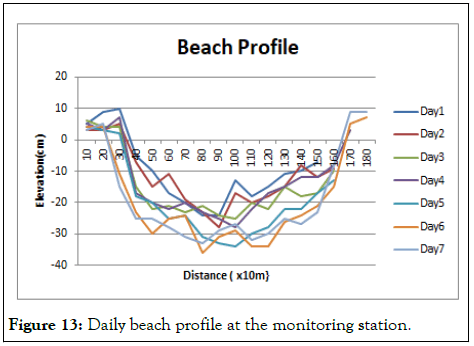
Figure 13: Daily beach profile at the monitoring station.
On the other hand, every successive landward migration of the first harmonic of infra-gravity waves impacts fresh area and subject other areas previously inundated susceptible to erosion as the net product by other lower harmonics [13]. More so, every successive seaward retreat of the first harmonic infra-gravity waves terminates perturbation of sediment on the shoreline area of its previous impact by the last backwash, and the expose beach segment becomes dry, stable and less prone to erosion. However, the above analysis can elucidate why the accelerating phase of neap-spring tide is destructive (erosional) and decelerating phase of spring-neap tide is constructive (depositional).
In summary, the first harmonic infra-gravity Waves oscillations perform the task of the main or primary bulldozer on the shoreline. When surf-zone is deficient of sediment, the first harmonic infra-gravity waves bulldoze and erode the beach, and transport sediment into surf-zone [14]. Similarly, when surf-zone experiences sediment surplus, the first harmonic infra-gravity waves group becomes the principal agent of deposition by discharging off surplus sediment to the beach. In fact, in the surf zone, it is the most destructive during sediment deficit/ constructive during sediment surplus of other harmonic groups in the infra-gravity wave spectrum. The second harmonic is the secondary bulldozer which performs intermediary functions between the first and the third harmonics of infra-gravity waves oscillations [15]. The third harmonic infra-gravity waves group levels the eroded material on the beach foreshore through longshore and cross-shore current transports. The fourth harmonic infra-gravity waves group winnows and sorts sediment for compaction by gravity waves.
First harmonic group of infra-gravity waves oscillations have been identified and delineated in the course of this investigation as the principal agent of erosion/accretion on the beach. These have strong implications on the near-shore sediment transport, beach erosion and sedimentation. Therefore, the understanding of energy radiation and transmission by different infra-gravity wave’s harmonic frequency groups on the shoreline is imperative for sustainable management of shoreline erosion, marinas and sea ports.
This research did not receive any grant from funding agencies in the public, commercial, or not-for-profit sectors. The assistance of the following personnel in the field: Gabriel Udo-Akuaibit and Uduak Akpan, is highly appreciated.
[Crossref] [Google Scholar] [PubMed]
Citation: Bahcevandziev K, Udo-Akuaibit SP (2024) Analysis of Nearshore infra-gravity Waves Oscillation Patterns and its Effects on Shoreline Morphodynamics, East of Qua-Iboe River Estuary, South-East Coast of Nigeria. J Oceanogr Mar Res. 12:303.
Received: 28-Jul-2020, Manuscript No. OCN-24-5677; Editor assigned: 31-Jul-2020, Pre QC No. OCN-24-5677 (PQ); Reviewed: 14-Aug-2020, QC No. OCN-24-5677; Revised: 03-Jun-2024, Manuscript No. OCN-24-5677 (R); Published: 30-Jun-2024 , DOI: 10.35248/2572-3103.24.12.303
Copyright: © 2024 Bahcevandziev K, et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.
Sources of funding : This research did not receive any grant from funding agencies in the public, commercial, or not-for-profit sectors. The assistance of the following personnel in the field: Gabriel Udo-Akuaibit and Uduak Akpan, is highly appreciated.