Journal of Thermodynamics & Catalysis
Open Access
ISSN: 2157-7544
+44 1300 500008
ISSN: 2157-7544
+44 1300 500008
Research Article - (2010) Volume 1, Issue 1
Effects of ?-cyclodextrin, ?CD, on refolding of lysozyme was investigated at pH 12 employing isothermal titration calorimetry (ITC) at 300K in 30mM Tris buffer solution. ?CD was employed as an anti-aggregation agent and the heats obtained for lysozyme+?CD interactions are reported and analyzed in terms of the extended solvation model. It was indicated that there are two sets of identical and non-cooperative sites for ?CD.
Keywords: Lysozyme; Isothermal titration calorimetry; β-cyclodextrin; Binding parameters.
Cyclodextrins (CDs) have been reported to suppress aggregate formation during the refolding of a wide range of proteins. Their potency is often ascribed to their affinity for aromatic amino acids, whose surface exposure would otherwise lead to protein association. However, no detailed structural studies are available. CDs, consisting of six, seven, or eight D-glucopyranose units, which are referred to as α-, β-, and γ-cyclodextrins, respectively. CDs inhibited the chemically induced aggregation and its inhibition was generally in the order of γ-CDs < α-CDs < β-CDs. Hydrophilic CDs reduced the thermally induced unfolding of lysozyme, suggesting that CDs destabilize native lysozyme or stabilize the unfolded state of lysozyme [1-3]. Electrophoresis data indicate that CDs, which promoted lysozyme refolding, arrested aggregation at the stage of smaller soluble aggregates [3].
Lysozyme is a natural enzyme serving as innate immune response antibiotics because it can damage bacterial cell wall. In humans, lysozyme distributes in almost all the secretions and tissues. Lysozyme is regarded as an important defense molecule of the innate immune system, because it can protect higher organisms from the infection of microorganisms. A thermal study between βCD and lysozyme was performed, in order to understand the mechanism of βCD-assisted protein refolding and to identify that βCD could function as good protein folding agents.
The tendency of lysozyme to aggregate is most distinct at pH 12. Thus, exposure to alkaline pH of 12 serves as a convenient approach to initiate the aggregation of lysozyme. In these conditions we can follow the anti-aggregation effect of βCD clearly.
The isothermal titration calorimetric experiments were carried out on a VP-ITC ultra sensitive titration calorimeter (MicroCal, LLC, Northampton, MA). The microcalorimeter consists of a reference cell and a sample cell of 1.8 mL in volume, with both cells insulated by an adiabatic shield. All solutions were thoroughly degassed before use by stirring under vacuum. The sample cell was loaded with lysozyme solution (1.26 mM ) and the reference cell contained buffer solution. The solution in the cell was stirred at 307 rpm by the syringe (equipped with micro propeller) filled with βCD solution (30mM) to ensure rapid mixing. Injections were started after baseline stability had been achieved. The titration of lysozyme with βCD solution involved 30 consecutive injections, the first injection was 5 µL and the remaining ones were 10 µL. In all cases, each injection was done in 6s at 3-min intervals. To correct the thermal effects due to βCD dilution, control experiments were done in which identical aliquots were injected into the buffer solution with the exception of lysozyme. In the ITC experiments, the heat changes associated with processes occurring at a constant temperature are measured. The measurements were performed at a constant temperature of 27.0±0.02 °C and the temperature was controlled using a Poly- Science water bath. The determined heats for lysozyme+βCD interaction were listed in Table 1 and shown graphically in Figure 1. The microcalorimeter was frequently calibrated electrically during the course of the study.
| [βCD]/mM | [lysozyme]/mM | q | qdilut |
| 0.107 | 1.255 | -1.413 | -0.765 |
| 0.319 | 1.246 | -1.086 | -0.733 |
| 0.530 | 1.238 | -0.951 | -0.719 |
| 0.740 | 1.229 | -0.869 | -0.709 |
| 0.948 | 1.220 | -0.795 | -0.686 |
| 1.155 | 1.211 | -0.734 | -0.659 |
| 1.360 | 1.203 | -0.706 | -0.645 |
| 1.563 | 1.194 | -0.717 | -0.674 |
| 1.765 | 1.186 | -0.737 | -0.686 |
| 1.965 | 1.177 | -0.730 | -0.677 |
| 2.164 | 1.169 | -0.686 | -0.657 |
| 2.361 | 1.160 | -0.632 | -0.632 |
| 2.557 | 1.152 | -0.581 | -0.592 |
| 2.751 | 1.144 | -0.541 | -0.561 |
| 2.944 | 1.136 | -0.511 | -0.541 |
| 3.135 | 1.128 | -0.487 | -0.527 |
| 3.325 | 1.120 | -0.468 | -0.511 |
| 3.513 | 1.112 | -0.448 | -0.495 |
| 3.700 | 1.104 | -0.436 | -0.487 |
| 3.885 | 1.096 | -0.426 | -0.475 |
| 4.068 | 1.088 | -0.414 | -0.468 |
| 4.251 | 1.080 | -0.404 | -0.457 |
| 4.431 | 1.073 | -0.392 | -0.446 |
| 4.610 | 1.065 | -0.384 | -0.437 |
| 4.787 | 1.057 | -0.394 | -0.427 |
| 4.963 | 1.050 | -0.384 | -0.420 |
| 5.137 | 1.042 | -0.3586 | -0.413 |
| 5.31 | 1.035 | -0.349 | -0.404 |
Table 1: Heats of lysozyem+βCD interactions, q, in βCD solution with w
We have shown previously [4-8] that the heats of the macromolecules+ligands interactions in the aqueous solvent systems can be reproduced by the extended solvation model as follows:
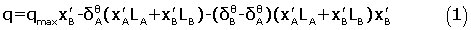
The parameters 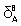 and
and 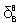 are indicative of lysozyme structural changes as results of its interaction with βCD, in the low and high βCD concentrations respectively. The extended solvation model is analogous to complexation, in which βCD takes the role of the ligands. The positive values for
are indicative of lysozyme structural changes as results of its interaction with βCD, in the low and high βCD concentrations respectively. The extended solvation model is analogous to complexation, in which βCD takes the role of the ligands. The positive values for 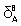 or
or 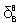 indicate that βCD stabilizes the lysozyme structure and vice versa.
indicate that βCD stabilizes the lysozyme structure and vice versa. 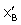 can be expressed as follows:
can be expressed as follows:
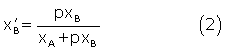
p > 1 or p is the fraction of bound βCD and 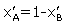 is the fraction of unbound βCD. We can express
is the fraction of unbound βCD. We can express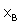 as follows:
as follows:
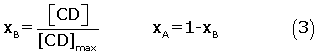
[βCD] is the concentration of βCD after every injection and [CD]max is the maximum concentration of βCD upon saturation of all lysozyme molecule. LA and LB can be calculated from heats of dilution of CD in water, qdilut , as follows:

The heats of lysozyme+βCD interactions were fitted to Eq. 1 over the entire βCD concentrations. In the fitting procedure, the only adjustable parameter (p) was changed until the best agreement between the experimental and calculated data was approached.
There are two distinct sets of binding sites on lysozyme, which are clear in Figure 1. The dissociation equilibrium constant (Kd) and the number of binding sites “g” can be determined by the following equation [4-8]
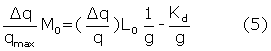
Where Δq = qmax - q and q represents the heat value at a certain βCD (L0 ) and lysozyme (M0) concentrations and qmax represents the heat value upon saturation of all lysozyme molecule. Therefore, the plot of 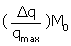 vs
vs 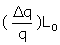 should be a linear plot with slope of “
should be a linear plot with slope of “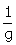 ” and the vertical-intercept of
” and the vertical-intercept of 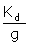 , which “g” and Kd can be obtained (Table 2). If q and qmax are calculated per mole of lysozyme, then the standard molar enthalpy of binding for each binding site, ΔH0 , will be
, which “g” and Kd can be obtained (Table 2). If q and qmax are calculated per mole of lysozyme, then the standard molar enthalpy of binding for each binding site, ΔH0 , will be  . The change in the standard Gibbs free energy, ΔG0 , and change in standard entropy of binding, ΔS0 , could be calculated by using association equilibrium constant, Ka =1 / Kd , and ΔH0 value in equations 6 and 7, respectively.
. The change in the standard Gibbs free energy, ΔG0 , and change in standard entropy of binding, ΔS0 , could be calculated by using association equilibrium constant, Ka =1 / Kd , and ΔH0 value in equations 6 and 7, respectively.
| parameters | First binding sites | Second binding sites |
| p | 1 | 1 |
| gi | 2.00±0.03 | 5.11±0.08 |
| Ka/M | 49967.89±21.15 | 130462.30±13.06 |
| ΔH/ kJ mol-1site-1 | -2.05±0.05 | -0.08±0.01 |
| ΔG/ kJ mol-1 site-1 | -26.80±0.15 | -29.18±0.25 |
| ΔS/ kJ mol-1 site-1 | 0.09±0.01 | 0.10±0.01 |
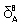 |
-0.36±0.03 | |
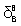 |
0.09±0.02 |
Table 2: Binding parameters for lysozyme+βCD interaction recovered from Eqs.1 and 2 at pH 12. p=1 indicates that the binding is non-cooperative in two sets of binding sites. Enthalpic force in the first binding sites is more important than entropic one, indicating that electrostatic interaction plays an important role in the interaction of lysozyme with βCD. The interaction in the second binding sites is stronger and both enthalpy and entropy driven but hydrophobic interaction has more important than electrostatic force.


The binding parameters recovered from Equations. 1, 5 and 6 were listed in Table 2. These results suggest that the effects of βCD on lysozyme refolding are attributed to its ability to suppress aggregation of lysozyme. βCD reduced the unfolding of lysozyme as evidenced by large values of association equilibrium constants (Ka=49967.89 and 130462 M-1 at the first and second set of binding sites respectively), suggesting that βCD stabilize native or unfolded state of lysozyme. The binding process for inhibition of lysozyme aggregation at the first set of binding sites was both enthalpy and entropy driven (Table 2), but electrostatic interaction plays an important role in the binding processes. The interaction in the second set of binding sites is stronger and both enthalpy and entropy driven but hydrophobic interaction is more important than electrostatic force for the inhibition of lysozyme aggregation (Table 2). βCD has a stronger affinity for lysozyme at the second set of binding sites, as evidenced by larger association equilibrium constant. A negative 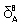 value (
value ( 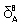 = -0.34) for the interaction is a characteristic of the electrostatic interactions underlying many non-specific ligand protein interactions, indicating that βCD destabilizes lysozyme structure. Destabilization of lysozyme by βCD indicates that βCD binds preferentially to the unfolded lysozyme or to a partially folded intermediate form of lysozyme. Such effects are characteristic of nonspecific interactions, in that the nonspecific ligand binds weakly to many different groups at the protein/water interface. Therefore, the calorimetric results suggest that inhibition of lysozyme aggregation is the result of nonspecific interactions at the first set of binding sites. In the other words, the negative
= -0.34) for the interaction is a characteristic of the electrostatic interactions underlying many non-specific ligand protein interactions, indicating that βCD destabilizes lysozyme structure. Destabilization of lysozyme by βCD indicates that βCD binds preferentially to the unfolded lysozyme or to a partially folded intermediate form of lysozyme. Such effects are characteristic of nonspecific interactions, in that the nonspecific ligand binds weakly to many different groups at the protein/water interface. Therefore, the calorimetric results suggest that inhibition of lysozyme aggregation is the result of nonspecific interactions at the first set of binding sites. In the other words, the negative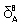 values followed by positive value of
values followed by positive value of 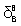 indicates that firstly, the non-specific binding of βCD to exposed side-chains on unfolded lysozyme will destabilize the native folded form of lysozyme. Alternatively, interactions with groups on oligomeric folded proteins can lead to dissociation of these protein aggregates. Finally, cyclodextrin interaction with unfolded proteins may enhance the solubility of partially denatured lysozyme by masking the exposed hydrophobic residues, thereby assisting the refolding of lysozyme molecule. These results suggest that βCD suppress the aggregation of lysozyme refolding, which are in agreement with the previous reports [1-3].
indicates that firstly, the non-specific binding of βCD to exposed side-chains on unfolded lysozyme will destabilize the native folded form of lysozyme. Alternatively, interactions with groups on oligomeric folded proteins can lead to dissociation of these protein aggregates. Finally, cyclodextrin interaction with unfolded proteins may enhance the solubility of partially denatured lysozyme by masking the exposed hydrophobic residues, thereby assisting the refolding of lysozyme molecule. These results suggest that βCD suppress the aggregation of lysozyme refolding, which are in agreement with the previous reports [1-3].