Journal of Physical Chemistry & Biophysics
Open Access
ISSN: 2161-0398
ISSN: 2161-0398
Research Article - (2014) Volume 4, Issue 5
Cisplatin is an important anti-cancer agent widely used in the clinic; however, it has several notable limitations. To develop novel platinum analogues, key characteristics were considered that may result in more effective platinum analogues. Herein results based on ab initio geometry optimizations (gas- and solution-phase) on cisplatin (1), oxaliplatin_1R_2R (2) and BNP3029 (3, a novel substituted cyano ligand-based platinum analogue, PtCl2[N≡C(CH2)3(C6H5)]2) using the recently published potentials and basis sets for platinum are presented. Optimized quantum mechanical derived geometries of the 3 platinum agents were in good agreement with available experimental geometries. The reactivity of BNP3029 was compared to cisplatin by computing the activation free energy barriers for the attack of various nucleophiles on both 1 and 3 and their monoaquated derivatives. Based on the activation energy barriers, it was determined that: (i) the reaction rate may be similar for the attack of water on cisplatin and BNP3029; (ii) the reaction rate for the attack of DNA bases was slower for monoaquated BNP3029 compared to monoaquated cisplatin; and (iii) the reaction rates for a thiol/thiolate attack on monoaquated cisplatin or monoaquated BN3029 were similar. BNP3029 demonstrated potent cytotoxic activity in a variety of human cancer cell lines in comparison to cisplatin and oxaliplatin and also had potent cytotoxic activity in several platinum resistant cell lines.
<Keywords: Cisplatin; Oxaliplatin; BNP3029; Anti-cancer agents
Cisplatin has been an important anticancer agent and has demonstrated a broad spectrum of anti-cancer activity against a variety of tumors including germ cell tumors, ovarian and bladder carcinomas, squamous cell tumors of the head and neck, esophageal cancers, and non-small cell lung tumors either as a single agent or in combination with other chemotherapy drugs (Figure 1) [1-3]. Despite its success as an important anti-cancer agent, cisplatin does have severe limitations in its use such as nephrotoxicity, neurotoxicity, nausea, ototoxicity and resistance to treatment over time [1]. In order to overcome these limitations, newer analogues of platinum have been developed, such as carboplatin and oxaliplatin [4] (Figure 1), but their use is limited compared to cisplatin or is a tradeoff of different serious toxicities (e.g., thrombocytopenia for carboplatin). Nedaplatin (approved only in Japan for esophageal cancer), Heptaplatin (approved in South Korea for gastric cancer) and Lobaplatin (approved in China for bladder cancer) are some of the other platinum analogues currently used in a limited country-specific manner, but these other platinum agents are not approved for use in most countries [3] (Figure 1). Multinuclear platinum analogues, notably BBR3464, have been evaluated in clinical trials but did not, ultimately, gain approval [3]. Since the discovery of cisplatin’s anti-tumor properties, hundreds of platinum analogues have been synthesized and tested for their anti-tumor properties and only very few have been approved for use in patients [3,5]. Newer analogues such as Picoplatin and Satraplatin [6] {a Pt (IV) analogue and also the first oral platinum agent} are currently in clinical trials (Figure 1).
Intracellularly, the postulated mechanism of action of cisplatin involves the initial replacement of one of the two chlorine atoms in cisplatin by water resulting in the formation of a reactive monoaquomonochloro species [3]. This reactive monoaquo species attacks the exposed imidazole N7 of guanine on DNA, initially yielding a monoplatinum- DNA adduct. Once this platinum-DNA adduct is formed, the second chlorine atom on cisplatin may undergo aquation. Then, this species or the native form attacks N7 on an adjacent guanine thereby forming a majority proportion of 1,2-GG intrastrand adducts with DNA. In the majority of cases, cisplatin forms an intrastrand DNA adduct, and has a well documented selectivity for adjacent GG dinucleotide sequences [1]. 1, 2-intrastrand adducts of cisplatin with DNA are also known with adjacent AG dinucleotides but not with GA dinucleotides [1]. A minor category of cisplatin DNA adducts are due to the formation of 1,3-intrastrand (GXG, 10%, X= any nucleotide) and 1,2-interstrand (<2%) crosslinks. All of these platinum-DNA adducts have the potential to be cytotoxic and/or induce apoptosis by the inhibition/modulation of multiple intracellular pathways important for cell growth, cell division and cell survival, including replication and transcription.
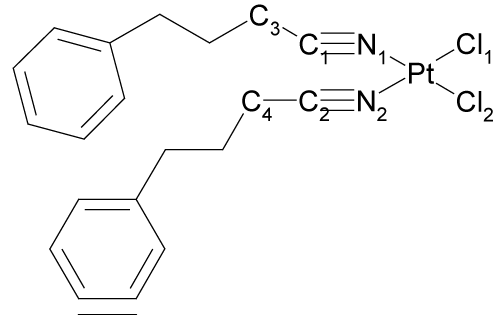
Cisplatin is an important anti-cancer agent; however, some patients develop resistance to cisplatin and the potential nephrotoxic and neurotoxic side effects also have to be carefully managed in the clinical setting [1]. In order to develop novel platinum analogues, key characteristics were considered that may result in more effective platinum analogues, such as: (a) reduced reactivity towards aquation; (b) reduced reactivity towards thiol or thiolate attack; and (c) reduction in excision repair of platinum-DNA lesions by NER proteins by maintaining a normal DNA form (B-DNA). Accordingly, we synthesized a series of platinum analogues with substituted cyano group as the carrier ligand [7] and herein describe computational studies on BNP3029 (Figure 1), a representative substituted cyano ligand-based novel platinum complex. There have been several computational studies on (a) structural properties of cisplatin and analogues [8-10]; (b) aquation of cisplatin and analogues [11-14]; (c) the base preferences of cisplatin and simulation studies on platinum-DNA adducts [15-18]; and (d) the attack of thiol/thiolate on cisplatin [19-21] and where applicable we compare this historical data with our current data.
In this paper, we describe a comprehensive comparative computational study on the structure and reactivity of cisplatin, oxaliplatin, and BNP3029 using the recently Published Date: effective core potentials and basis sets for platinum. Differences in the reactivity of cisplatin and BNP3029, and their monoaquated derivatives, with respect to nucleophilic attack by water, guanine, adenine, thiolate (-SCH3), and thiol (HSCH3) are also evaluated herein by comparing the activation free energy barriers for these reactions.
MCF7/WT cells (human breast cancer), MCF7/ADR (adriamycin resistant MCF7 human breast cancer), A2780 cells (human ovarian cancer), A2780/CP3 cells (cisplatin resistant human ovarian cancer), A2780/C25 cells (oxaliplatin resistant human ovarian cancer) and HCT8/WT cells (human colon cancer) that were gifts from Dr. Y. Rustum of Roswell Park Cancer Institute (RPCI). MCF7-MR cells were a gift from Dr. W. Dalton at Moffit Cancer Center. MCF7/OxR cells (oxaliplatin resistant human breast cancer) and HCT-8/CisR cells (cisplatin resistant colon cancer cells) were developed at BioNumerik Pharmaceuticals, Inc. GLC/WT (human small cell lung cancer) and GLC/ADR cells (Adriamycin resistant human small cell lung cancer) were a gift from Dr. EGE de Vries and Dr. C. Meijer of the University Medical Center Groningen, Netherlands. SW480 cells (human colon cancer), CAMA-1 cells (human breast cancer), NCI-H23 cells (human lung cancer), and NCI-H838 cells (human lung cancer) were purchased from American Type Culture Collection (ATCC). NCI-H23 and NCI-H838 were developed in the laboratory of Dr. Herbert Oie and were used pursuant to a license obtained from the Public Health Service/National Institutes of Health.
Computational Methods
The computational studies described herein were performed in using the Gaussian 03 program [22] with the density functional theory.
Cell Culture and Cytotoxicity Methods
Population doubling time for each of the cell lines used herein varied, but experiments encompassed four to five total cell doublings corresponding to approximately 4 days for HCT-8 and HCT8/CisR; 6 days for SW480; 5 days for MCF7/WT, MCF7/MR, MCF7/ADR, MCF7/OxR, A2780/WT, A2780/CP3, A2780/C25, GCL/WT and GCL/ ADR; 6 days for CAMA-1; and 7 days for NCI-H838, and NCI-H23. Exponentially growing cells were seeded in 96 well microtiter plates (number of cells/well shown parenthetically) as follows: MCR7/WT (600), MCR7/ADR (1500), MCR7/MR (1500), MCF7/OxR (600), A2780/WT (500), A2780/CP3 (1000), A2780/C25 (1000), NCI-H23 (600), NCI-H838 (600), GLC/WT (500), GLC/ADR (500), HCT8 (800), HCT8CisR (1200), and CAMA-1 (800) (100 μL seeding volume). Cells were allowed to attach to microtiter plates in CO2 incubators at 37°C overnight prior to drug treatment. Cells were treated with cisplatin, BNP3029, and oxaliplatin for 1 hour at 37C. Following drug exposure, medium was removed; cells were washed once with medium and incubated in drug-free medium for four to five cell doublings. After a total of four to five cell doublings, cell survival was evaluated using the Sulforhodamine B (SRB) assay [23].
Electronic Structure Calculations
Ab Initio optimizations (geometries and transition states) were performed using Gaussian 03 program [22] with the density functional theory [24]. The exchange-correlation functional mPW1PW (exchange component of Perdew-Wang as modified by Barone and correlation functional by Perdew-Wang) was employed [25]. Dunning’s correlation consistent valence double-zeta cc-pVDZ basis set for the ligands such as NH3, Cl, OH2, N≡C(CH2)3(C6H5), oxalate and 1,2-DACH (1,2-diaminocyclohexane) was used [26]. Newest energy-consistent pseudopotentials and correlation consistent basis set for the 5d element (Pt) from Stoll et al. [27] were employed. Six Cartesian d functions for polarization were used in all of our geometry optimizations and energy evaluations. Transition states were verified using vibrational frequency calculations (one imaginary frequency). Thermal corrections to the free energies were obtained from frequency calculations at 25°C and 1 atm. When comparing the calculated activation energy barriers with experimental data, the calculated energy barriers were corrected for liquid-phase concentrations of 1 mol/L by subtracting 1.9 kcal/mol from the calculated activation energy barriers (correction factor is: RT ln(24.46) = 1.9 kcal/mol where T = 298 K; 24.46 is the volume occupied by 1 mole of gas at 298 K) [28].
Activation Free Energy Barrier Calculation
The following equations (eqsuations 1-5) were used to compute the activation free energy barriers for the attack of various nucleophiles on cisplatin and BNP3029 and their monoaquated derivatives. The activation free energy barrier was the energy difference between the transition state (TS) and the reactants in each equation. Using the gas-phase optimized geometries for each of the reactant and the TS, single point energies were obtained with the inclusion of solvent and employing the same basis sets and potentials used in the gasphase optimizations. The solution energies for each reactant and TS were obtained by adding the Total free energy in solution with all non electrostatic terms and Total non electrostatic terms. To this sum, we added the thermal corrections to the free energy (obtained using the frequency option from the gas-phase calculation). The solvent effects were added using the polarizable continuum model (PCM) within the integral equation formalism (IEF-PCM) [29] using the Pauling radii.
PtL2Cl2 + H2O → PtL2Cl2(H2O) (1)
L= NH3 (1a), ((C6H5)(CH2)3C≡N) (3a)
PtL2Cl(OH2)1+ + guanine → PtL2Cl(guanine)(H2O)1+ (2)
L= NH3 (1b), ((C6H5)(CH2)3C≡N) (3b)
PtL2Cl(OH2)1+ + adenine → PtL2Cl(adenine)(H2O)1+ (3)
L= NH3 (1c), ((C6H5)(CH2)3C≡N) (3c)
PtL2Cl(OH2)1+ + SHCH3 → PtL2Cl(SHCH3)(H2O)1+ (4)
L= NH3 (1d), ((C6H5)(CH2)3C≡N) (3d)
PtL2Cl(OH2)1+ + SCH3- → PtL2Cl(SCH3)(H2O) (5)
L= NH3 (1e), ((C6H5)(CH2)3C≡N) (3e)
Several studies have been reported on the optimized geometries of cisplatin using a variety of methods, basis sets and potentials with recommendations on how to treat cisplatin computationally [10]. As far as we can ascertain, none of these aforementioned studies employed the newest platinum basis set and effective core potential reported recently [27]. Here we employed the newest basis set and potential from Stoll’s group for platinum along with the recommended cc-pVDZ basis set on the ligand atoms within the density functional formalism for the geometry optimizations of cisplatin, oxaliplatin and BNP3029 to assess the performance of the new basis set and potential for platinum. Using the gas-phase optimized geometries, we also performed solution-phase optimizations of cisplatin, oxaliplatin and BNP3029 using the same basis sets and the potentials employed in the gas-phase optimizations and compared the gas-phase and solution-phase geometries to the experiment, where available (see details in sections 7.1 to 7.3).
Optimized geometries of Cisplatin
The gas-phase optimized geometries of cisplatin at the mPW1PW/ cc-pVDZ basis set for Cl, and NH3 and the platinum basis set and potential from Stoll’s group [27] are shown in Table 1 and Figure 2A. The gas-phase optimized geometry indicated that the Pt-Cl bond length (2.292 Å) was shorter than the experimental value (2.330 Å) whereas the Pt-N bond length (2.077 Å) was longer than the experimental value (2.010 Å). Inclusion of solvent corrected these anomalies with both Pt- Cl (2.340 Å) and Pt-N (2.037 Å) bond lengths agreeing very well with the experimental values [30].
| Cisplatin | Pt-Cl | Pt-N | N-Pt-N | N-Pt-Cl | Cl-Pt-Cl |
|---|---|---|---|---|---|
| Calculated | 2.292 2.340 | 2.077 2.037 | 98.4 90.5 | 83.1 88.4 | 95.4 92.7 |
| Expt (averaged)1 | 2.330 | 2.010 | 87.0 | 90.3 | 91.9 |
| Expt (range)1 | 2.333, 2.328 | 1.950, 2.050 | 87.0 ± 1.5 | 92.0 ± 1, 88.5 ± 1 | 91.9 ± 0.3 |
Table 1: Gas-phase and solution-phase (bold) optimized geometries of cisplatin and the comparison of the geometries from experiment. All distances are in Å and angles are in°
Figure 2: Optimized structures of A) Cisplatin. Left: gas-phase optimized geometry and Right: Solvent-phase optimized geometry. B) Oxaliplatin (transl_ 1R_2R, trans-d_1S_2S and cis_1R_2S conformers of oxaliplatin in that order from left to right); C) BNP3029 (all gauche, all trans, trans/gauche forms of cyano ligands on BNP3029 in that order from left to right). All distances are in Angstroms (Å) and angles are in degrees.
Optimized geometries of Oxaliplatin
The three isomeric forms of DACH ligand (1,2-diaminocyclohexane; trans-d_1S_2S; trans-l_1R_2R and cis_1R_2S) yield three isomers of oxaliplatin and we optimized all three of them (Figure 2B) [31]. The trans-l_1R_2R form of oxaliplatin was more stable than the transd_ 1S_2S form of oxaliplatin by 0.28 kcal/mol based upon the solventphase optimized geometries and energies after accounting for thermal corrections. There are only a few reported theoretical studies on the geometries and energies of oxaliplatin [9] and data reported indicate that the trans-d_1S_2S isomer was more stable than the trans-l_1R_2R by 0.30 kcal/mol using gas-phase energies. The optimized geometries of all the three conformers of oxaliplatin are shown in Table 2. As seen in the case of cisplatin, inclusion of solvent (bold numbers in Table 2) led to optimized geometries of oxaliplatin that were in much closer agreement with the experimental values.
 1Oxaliplatin_1R_2R [31]. 1Oxaliplatin_1R_2R [31]. |
||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Pt-N1 | Pt-N2 | Pt-O1 | Pt-O2 | C1-C2 | C1-N1 | C2-N2 | O1-C3 | O2-C4 | C3-O3 | C4-O4 | C3-C4 | N1-Pt-N2 | N1-Pt-O1 | N2-Pt-O2 | O1-Pt-O2 | Pt-O2-C4 | Pt-O1-C3 | O2-C4-C3 | O1-C3-C4 | O2-C4-O4 | O1-C3-O3 | |
| 1R_2R | 2.034 2.067 | 2.034 2.067 | 2.029 1.975 | 2.029 1.975 | 1.521 1.530 | 1.487 1.483 | 1.487 1.482 | 1.299 1.327 | 1.299 1.327 | 1.223 1.203 | 1.223 1.203 | 1.544 1.553 | 82.9 83.0 | 97.6 96.2 | 97.6 96.2 | 82.0 84.6 | 113.5 113.1 | 113.5 113.1 | 115.5 114.6 | 115.5 114.6 | 123.9 123.3 | 123.9 123.3 |
| 1S_2S | 2.034 2.066 | 2.033 2.067 | 2.030 1.975 | 2.029 1.975 | 1.520 1.529 | 1.487 1.482 | 1.487 1.483 | 1.298 1.327 | 1.298 1.327 | 1.223 1.203 | 1.223 1.203 | 1.544 1.553 | 82.9 83.0 | 97.5 96.2 | 97.7 96.2 | 81.9 84.6 | 113.5 113.1 | 113.6 113.1 | 115.5 114.6 | 115.5 114.6 | 123.9 123.4 | 123.8 123.4 |
| 1R_2S | 2.030 2.065 | 2.031 2.064 | 2.029 1.975 | 2.029 1.975 | 1.526 1.536 | 1.489 1.489 | 1.497 1.489 | 1.299 1.327 | 1.299 1.327 | 1.222 1.204 | 1.222 1.204 | 1.543 1.553 | 82.7 82.9 | 97.7 96.2 | 97.7 96.3 | 81.9 84.6 | 113.6 113.1 | 113.6 113.1 | 115.5 114.6 | 115.5 114.6 | 123.9 123.4 | 123/9 123.4 |
| Expt1 | 2.040 | 2.060 | 2.040 | 2.010 | 1.490 | 1.540 | 1.540 | 1.320 | 1.210 | 1.190 | 1.290 | 1.560 | 83.8 | 98.6 | 96.0 | 82.5 | 112.0 | 141.0 | 122.0 | 110.0 | 124.0 | 124.0 |
Table 2: Gas-phase and solution-phase (bold) optimized geometries of oxaliplatin and the comparison of the geometries from experiment. All distances are in Å and angles are in°
Optimized geometries of BNP3029
The structure of BNP3029 is similar to that of cisplatin in that the two carrier ligands (NH3s) are replaced by two substituted cyano groups or ligands [(C6H5)(CH2)3C≡N]. These substituted cyano ligands may adopt all trans, all gauche, or trans/gauche arrangements (Figure 2C). The gas-phase optimized energies indicated that the all gauche conformer of BNP3029 was more stable than trans/gauche conformer by 1.25 kcal/mol, and 1.64 kcal/mol more stable than the all trans conformer after accounting for thermal corrections. When these geometries were reoptimized using solvent, a different stability order was observed with the all trans conformer appearing more stable than the trans/gauche conformer by 1.27 kcal/mol, and the all gauche arrangement by 2.89 kcal/mol.
As seen from gas-phase optimized geometries of cisplatin and oxaliplatin, the gas-phase geometries of BNP3029 also showed shortened Pt-Cl bond lengths compared to the Pt-Cl bond lengths from solution-phase optimized geometries (Table 3). Another notable difference was the deviation from linearity in Pt-N-C and N-C-C bond angles using the gas-phase optimized geometries compared to the ones from solvent-phase optimized geometries of BNP3029 (Table 3). A crystal structure for BNP3029 was not available; therefore the calculated geometries of BNP3029 were compared with the related crystal structure, PtCl2(N≡CCH2CH3)2 [32]. As seen in Table 3, both gas- and solution-phase optimized geometries of BNP3029 are in reasonable agreement with the experimental geometries of PtCl2(N≡CCH2CH3)2.
 1Pt[N=C(C2H5)]2Cl2 [32]. 1Pt[N=C(C2H5)]2Cl2 [32]. |
||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Pt-N1 | Pt-N2 | Pt-Cl1 | Pt-Cl2 | N1-C1 | N2-C2 | C1-C3 | C2-C4 | N1-Pt-N2 | N1-Pt-Cl1 | N2-Pt-Cl2 | Cl1-Pt-Cl2 | Cl2-Pt-N1 | Cl1-Pt-N2 | Pt-N1-C1 | Pt-N2-C2 | N1-C1-C3 | N2-C2-C4 | |||||
| All trans | 1.958 1.963 | 1.958 1.963 | 2.314 2.282 | 2.312 2.281 | 1.153 1.154 | 1.153 1.154 | 1.448 1.453 | 1.448 1.453 | 90.1 94.5 | 89.7 86.9 | 89.4 87.2 | 90.7 91.3 | 179.5 178.3 | 179.8 178.5 | 178.2 172.7 | 179.7 173.5 | 179.3 176.7 | 178.9 177.6 | ||||
| gauche/trans | 1.958 1.964 | 1.958 1.960 | 2.313 2.286 | 2.312 2.280 | 1.154 1.154 | 1.154 1.154 | 1.450 1.455 | 1.448 1.453 | 90.4 94.5 | 89.4 86.9 | 89.4 87.4 | 90.8 91.2 | 179.7 178.1 | 179.8 178.6 | 179.2 171.4 | 179.6 174.0 | 179.1 175.5 | 179.1 177.7 | ||||
| All gauche | 1.958 1.963 | 1.958 1.962 | 2.312 2.286 | 2.313 2.285 | 1.154 1.154 | 1.154 1.154 | 1.450 1.455 | 1.450 1.455 | 90.4 94.9 | 89.4 87.0 | 89.5 87.0 | 90.7 91.2 | 179.8 178.2 | 179.8 178.1 | 179.6 171.5 | 179.8 171.8 | 179.7 175.5 | 179.0 175.8 | ||||
| Expt1 | 1.940 | 1.980 | 2.263 | 2.263 | 1.200 | 1.190 | 1.420 | 1.440 | 89.8 | 89.9 | 89.6 | 90.6 | 179.5 | 177.0 | 177.0 | 175.0 | 176.0 | 177.0 | ||||
Table 3: Gas-phase and solution-phase (bold) optimized geometries of BNP3029 and the comparison of the geometries from experiment1. All distances are in Å and angles are in°.
Activation energy barriers
In order to elucidate how fast/slow BNP3029 reacts with nucleophiles, as compared to cisplatin, the transition state geometries for the attack of various nucleophiles were optimized at the gas-phase using the above mentioned basis sets and effective core potentials. To evaluate the activation energy barriers, solvent contributions to the gas-phase optimized geometries of the transition state and the reactants were included. Table 4 provides the activation energy barriers for the attack of nucleophiles on cisplatin and BNP3029 and their monoaquated derivatives. The barriers indicate that the reaction rate may be similar for the attack of water on cisplatin and BNP3029; the reaction rate for the attack of guanine or adenine on monoaquated BNP3029 is slower compared to an attack by the same nucleophiles on monoaqauted cisplatin; and the reaction rate may be similar for the attack of thiol or thiolate on monoaquated cisplatin and monoaquated BNP3029 (energy barriers and the transition state (TS) geometries are explained in detail below).
Activation energy barriers for the aquation of Cisplatin and BNP3029 (equation 1) and the TS geometries for aquation (1a and 3a)
Several computational studies identify the transition state for the nucleophilic attack of water on cisplatin as trigonal bipyramidal (TBP) geometry with the incoming water and the outgoing chloride ion along with the one of the ammines occupying the equatorial plane (Figure 3A, 1a) [11-14]. A similar TBP transition state geometry was also found for the nucleophilic attack of water on BNP3029 (Figure 3B, 3a). The activation free energies (for details see activation free energy barrier calculation section) are given in Table 4. The computed activation free energy barrier for the aquation of cisplatin was 2-4 kcal/mol higher compared to the experimental barriers. The barriers from Table 4 indicate that the activation free energy barrier is slightly higher for 3a compared to 1a. The magnitude of the difference, which is within the typical margin of the computational error, may indicate that the reaction rate going through this transition state and forming the monoaquated species may be similar for cisplatin and BNP3029. The TBP geometry for the TS attack of water on cisplatin (1a) and BNP3029 (3a) and the geometric parameters are given in Figure 3.
The geometry of 1a (Figure 3C), but not 3a, was optimized using solvent. Optimized geometries of cisplatin (1, gas- and solution- phase, Table 1) indicated that the inclusion of solvent resulted in increased Pt- Cl bond lengths. A similar trend in Pt-Cl bond lengths was obtained with the inclusion of solvent during the optimization of 1a compared to the gas-phase geometries (Pt-Cleq bond length in gas-phase: 2.730 Å and solution-phase: 2.783 Å). Inclusion of solvent also resulted in the increase of the Pt-OH2 bond lengths (gas-phase: 2.396 Å and solutionphase: 2.495 Å) leading to a late TS (identified as having a geometry with long Pt-Cl (leaving) and long Pt-OH2 (incoming) bond lengths).
The equatorial bond lengths of Pt-Cl (2.699 Å) and Pt-OH2 (2.318 Å) in 3a (gas-phase) compared to the equatorial bond lengths of Pt-Cl (2.730 Å) and Pt-OH2 (2.396 Å) in 1a (gas-phase) were indicative of an early transition state for 3a (identified as having a geometry with short Pt-Cl (leaving) and short Pt-OH2 (incoming) bond lengths). The Cyanoeq-Pt-Oeq bond angle (152.5º) was larger for 3a compared to that in 1a (149.8°), leading to a shorter OH2 -H---Cl distance in 3a (2.021 Å) compared to that in 1a (2.063 Å). Since Oeq-Pt-Cleq bond angles were similar in 1a and 3a, the Cyanoeq-Pt-Cleq bond angle in 3a (138.6º) became shorter than the corresponding value in 1a (142.0º).
Activation energy barriers for the attack of DNA bases (guanine, equation2 and adenine, equation 3) on monoaquated cisplatin and monoaquated BNP3029 and the TS geometries (1b, 3b; and 1c, 3c)
Nucleophilic attack by DNA bases (guanine or adenine) on monoaquated cisplatin or monoaquated BNP3029 also resulted in a transition state geometry similar to 1a and 3a. The incoming DNA bases and the outgoing water, along with one of the ammines, occupy the equatorial plane. The computed activation energy barrier for the attack of guanine on monoaquated cisplatin was 2.3 kcal/mol lower compared to the experimental barrier. As seen in Table 4, the barrier for the attack of guanine on monoaquated cisplatin is lower than that for monoaquated BNP3029, indicating a faster reaction of guanine with monoaquated cisplatin (equation 2). The activation free energy barrier for the attack of adenine on monoaquated cisplatin is higher compared to that of the barrier obtained for the attack of guanine on monoaquated cisplatin (Table 4). This observation is in accord with the data from previous studies indicating that guanine was the preferred site of attack by monoaquated cisplatin followed by adenine. As seen with the nucleophilic attack by guanine and adenine on monoaquated cisplatin, the reaction rate for adenine with monoaquated BNP3029 is slower compared to the reaction rate of guanine with monoaquated BNP3029 (Table 4).
| Nucleophile | Cisplatin | BNP3029 |
|---|---|---|
| OH2 | 27.7 23.8b, 24.1c,24.3±1.5d | 28.6 |
| Guanine | 21.1 23.4e | 24.0 |
| Adenine | 30.3 | 34.5 |
| SCH3-1 | 14.9 | 15.7 |
| SHCH3 | 27.8 | 29.3 |
Table 4: Activation free energiesa for the nucleophilic attack of water on cisplatin and BNP3029; or for the nucleophilic attack on monoaquated cisplatin or BNP3029 by guanine, adenine, thiolate and thiol. Bold numbers refer to the experimental activation free energies.
The TS geometry for the attack of guanine on monoaquated cisplatin (1b) resembles a TBP with the leaving OH2 , incoming guanine, and one of the NH3 forming the equatorial plane, and the other NH3 and Cl- occupying the axial positions (Figure 4A). One of the hydrogens from the axial NH3 is in hydrogen-bond contact with the O6 of guanine (1.700 Å). As a consequence, the hydrogen’s distance with ammonia N increases to 1.048 Å compared to other two N-H distances which were 1.021 Å and 1.022 Å. A second TS geometry for 1b (similar 1b geometry was obtained by Costa et al. [18]) is shown in Figure 4B. This TS geometry resulted in a slightly higher activation free energy barrier compared to the TS geometry presented in Figure 4A. In Figure 4B, the outgoing OH2 is in hydrogen-bond contact with the O6 atom of the incoming guanine (1.904 Å). Also, the O6 atom of the incoming guanine is in hydrogen-bond contact with the axial NH3 hydrogen (1.900 Å) as seen in Figure 4A. The equatorial Pt-N7 and Pt-O bond lengths are longer in Figure 4A (2.498 Å and 2.397 Å) than in Figure 4B (2.489 Å and 2.362 Å). As a result, N7eq-Pt-Oeq bond angle (73.1º) in Figure 4B is slightly larger than that in Figure 4A (70.3º).
Figure 4: Optimized transition state geometry for the guanine attack on A) monoaquated cisplatin from gas-phase: Pt(NH3)2Cl(OH2)(guanine) (1b); B) monoaquated cisplatin from gas-phase: Pt(NH3)2Cl(OH2)(guanine) (1b); C) monoaquated BNP3029 from gas-phase: Pt[N≡C(CH2)3(C6H5)]2Cl(OH2) (guanine) (3b). All distances are in Angstroms (Å) and angles are in degrees.
The TS geometry for the attack of guanine on monoaquated BNP3029 (Figure 4C, 3b) exhibited similar TBP geometry with the leaving OH2, incoming guanine, and one of the cyano ligands forming the equatorial plane, and the other cyano ligand and Cl- occupying the axial positions. Since O6 of guanine cannot hydrogen-bond with the cyano ligand, the O6 of guanine forms hydrogen-bonds with the equatorial bound OH2 (1.718 Å) hydrogens. This TS geometry of 3b is similar to the TS geometry obtained for the attack of guanine on monoaquated cisplatin by Costa et al. (Figure 4B) [18]. The Cyanoeq-Pt- Oeq bond angle (144.2º) is smaller in 3b compared to the value observed in 1b (148.1º, Figure 4A). Correspondingly, the N7eq-Pt-Oeq bond angle becomes wider in 3b (75.1º, Figure 4C) versus 1b (70.3º, Figure 4A).
The main features of the transition state geometry for the attack of adenine on monoaquated cisplatin (Figure 5A, 1c) are the NH2-H- --Cl contact between C6-NH2 of Adenine and the axial Cl- (2.403 Å); the OH2-O hydrogen-bond contact with the axial NH3-H (2.873 Å); the OH2-O O---C-H contact with the C8-H (2.615 Å) of adenine; the hydrogen-bond contact between N7(adenine)---H-OH2 (2.497 Å); and the equatorial NH3-H---Cl contact of 2.525 Å. Since both OH2 and equatorial NH3are involved in hydrogen-bond interactions, we noticed an increase of the NH3(eq)-Pt-OH2(eq) bond angle from 148.1º in 1b to 153.0º in 1c. Accordingly, the OH2(eq)-Pt-N7eq bond angle (adenine in the equatorial position) shortens by 2º from the value in 1b.
The transition state geometry for the attack of adenine on monoaquated BNP3029 (Figure 5B, 3c) shows hydrogen-bond contact between OH2-H and the adenine C6-NH2 (1.844 Å), OH2-H---Cl (axial) contact of 2.745 Å, and a concomitant decrease in the Cyano(eq)- Pt-N7(adenine, eq) bond angle from 140.6º in 3b to 138.6º in 3c. Accordingly, OH2(eq)-Pt-N 7(adenine, eq) increases by 2º in 3c compared to the value in 3b.
Activation energy barriers for the attack of thiol (eq. 4) and thiolate (eq. 5) on monoaquated cisplatin and monoaquated BNP3029 and the TS geometries (1d, 3d; and 1e, 3e)
Thiol-containing biomolecules such as glutathione are known to inactivate cisplatin. Since pH is a function of the existing micro environment, nucleophilic attacks on monoaquated cisplatin and monoaquated BNP3029 by thiol (HSCH3) and thiolate (-SCH3) forms of the biological equivalent of cysteine were studied. The nucleophilic attack by thiol or thiolate on monoaquated cisplatin or monoaquated BNP3029 resulted in a TBP transition state geometry. The incoming thiol or thiolate and the outgoing water, along with one of the ammines, occupy the equatorial plane. As seen in Table 4, the barrier for the attack of thiol on monoaquated cisplatin is slightly lower than the barrier seen with monoaquated BNP3029, indicating a faster reaction of thiol with monoaquated cisplatin, whereas the barriers for the thiolate attack on monoaquated cisplatin and monoaquated BNP3029 are similar.
The TS geometries for the attack of thiol on monoaquated cisplatin (1d) and monoaqauted BNP3029 (3d) resemble a TBP arrangement with the leaving OH2, incoming thiol, and one of the ammine (substituted cyano group) forming the equatorial plane, and the other ammine (substituted cyano group) and Cl- occupying the axial positions are shown in Figure 6. In the TS geometry of 3d (Figure 6B), the hydrogen on thiol is in hydrogen-bond contact with the O of OH2 (2.673 Å), and the H on OH2 is in contact with the axial Cl- (2.497 Å). The above two contacts are also noticed in the TS geometry of 1d (Figure 6A, 2.736 Å and 2.618 Å respectively). The Neq-Pt-Oeq bond angle is similar in 1d (151.9º) and 3d (152.8º). Additionally, the Seq-Pt-Oeq bond angle is wider in both 1d (73.6º) and 3d (74.5º) and as a consequence the Neq- Pt-Seq bond angle is shorter in both 1d (133.7º) and 3d (132.2º).
The transition state geometries for the attack of thiolate on monoaquated cisplatin (1e) and monoaquated BNP3029 (3e) also resemble the TBP arrangement with the leaving OH2, incoming thiolate, and one of the ammines (substituted cyano group) forming the equatorial plane, and the other ammine (substituted cyano group) and Cl- occupying the axial positions (Figure 7). In the TS geometry of 1e (Figure 7A), one of the hydrogens on OH2 is in contact with the thiol-S (2.036 Å), and the other hydrogen is in contact with the axial Cl- (2.715 Å). The above two contacts are also noticed in the TS geometry of 3e (Figure 7B, 1.970 Å and 2.652 Å respectively). The Seq-Pt-Oeq bond angle is similar in 1e (67.1º) and 3e (68.8º). Additionally, the Neq-Pt-Oeq bond angle is wider in 3e (161.8º) compared to the one in 1e (157.6º), and as a consequence the Neq-Pt-Seq bond angle is shorter in 3e (130.1º) compared to the value in 1e (135.3º).
Cytotoxicity data for cisplatin, oxaliplatin and BNP3029 in wild-type and resistant cell lines
In order to assess the efficacy of the novel substituted cyano ligandbased platinum analogue, cytotoxicity of BNP3029 in a variety of human cancer cell lines was determined with cisplatin and oxaliplatin included as comparators. For comparison purposes, we also included cisplatin and oxaliplatin in the IC50 evaluations. As seen in Table 5, in the wild-type cell lines, including A2780 (ovarian cancer), MCF7 (breast cancer), NCI-H23 (non-small cell lung cancer), NCI-H838 (non-small cell lung cancer), CAMA-1 (breast cancer), SW480 (colon cancer), and HCT8 (colon cancer), BNP3029 consistently showed superior IC50 activity compared to cisplatin and oxaliplatin (except with respect to the GLC (small cell lung cancer) cell line, where BNP3029 showed comparable activity). Also, BNP3029 showed very promising activity in resistant cell types for A2780 (CP3 and C25), MCF7 (MR, ADR, OxR) and HCT8 (CisR), with better resistance factors (resistance factors were obtained by dividing the IC50 in resistant cell type by the IC50 in wild-type cell line).
| IC50 (µM) | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A2780 | MCF7 | GLC | NCI-H23 | NCI-H838 | CAMA-1 | SW480 | HCT8 | HCT8 | |||||||
| Drug | WT | CP3 | C25 | WT | MR | ADR | OxR | WT | ADR | WT | WT | WT | WT | WT | CisR |
| CDDP | 6.4 | 77.3 | 30.8 | 14.2 | 39.7 | 14.8 | 19.3 | 4.1 | 7.0 | 4.3 | 12.5 | 12.3 | 13.5 | 31.9 | 126.5 |
| Oxaliplatin | 4.0 | 23.4 | 43.7 | 20.4 | 47.7 | 32.1 | 131.5 | 3.7* | 9.6* | 13.9 | >100 | 36.4 | 36.1 | ||
| BNP3029 | 3.8 | 6.5 | 4.8 | 2.96 | 7.08 | 1.92 | 2.51 | 4.3 | 5.7 | 2.4 | 2.2 | 2.0 | 1.9 | 2.73 | 2.65 |
Table 5: IC50 (µM) values for cisplatin, oxaliplatin and BNP3029 using a variety of wild-type (WT) and drug-resistant cell lines. A2780/WT: human wild type ovarian cancer cell line; A270/CP3 is A2780 with cisplatin resistance cell line; A2780/C25 is A2780 with oxaliplatin resistance cell line; MCF7/ WT: human wild type breast cancer cell line; MCF7/MR is MCF7 with mitoxantrone resistance cell line; MCF7/ADR is MCF7 with adriamycin resistance cell line; MCF7/ OxR is MCF7 with oxaliplatin resistance cell line; GLC/WT: human wild type small cell lung cancer; GLC/ADR is humanGLC with adriamycin resistance cell line; NCI-H23/ WT: human wild type non-small cell lung cancer (adenocarcinoma); NCI-H838/WT: human wild type non-small cell lung cancer (adenocarcinoma); CAMA-1/WT: human wild type breast cancer (adenocarcinoma); SW480/WT: human wild type colorectal cancer (adenocarcinoma); HCT8/WT: human wild type colorectal cancer (adenocarcinoma); HCT8/CisR: humanHCT8 with cisplatin resistance cell line.
Since the discovery of cisplatin’s antitumor properties, many platinum analogues have been made and evaluated in clinical trials, yet very few have been approved for use in patients. Several studies have focused on understanding the activation energy barriers for the attack of nucleophiles on cisplatin or the monoaquated cisplatin to understand the platinum analogue’s reactivity.
The optimized geometries of cisplatin, oxaliplatin, and BNP3029 (novel substituted cyano ligand-based platinum analogue) employing the most current basis set and potentials for platinum resulted in good agreement with the experimental data, where available, with the inclusion of solvent providing a closer agreement. Studies described herein compared the reactivity of cisplatin and BNP3029 or their monoaquated species in their ability to react with various nucleophiles. Again, the computed activation free energy barriers were in reasonable agreement with experimental data for cisplatin. BNP3029 showed increased activation energy barriers for some nucleophiles such as guanine and adenine, indicating a slower reactivity with these nucleophiles compared to cisplatin. The reactivity of BNP3029 with other nucleophiles such as water or thiolate/thiol may be similar compared to cisplatin’s reactivity with these nucleophiles (the barriers for BNP3029 were slightly higher compared to the barriers with cisplatin). With no involvement of either NH3s or substituted cyano ligand (axial or equatorial), the transition state geometries (from gasphase) for the aquation of cisplatin or BNP3029 indicate that only the leaving Cl and the incoming OH2 are involved in leading to early (in case of BNP3029, Figure 3C) or slightly late (in case of cisplatin, Figure 3A) TS, thereby leading to similar barriers (albeit slightly higher in the case of BNP3029) for aquation.
The guanine nucleophile participates in stabilizing interactions with the axial NH3 in the case of monoaquated cisplatin (Figure 4A). However, such interactions are not possible with monoaquated BNP3029 due to the lack of hydrogens on substituted cyano ligands (Figure 4C) which may lead to higher barriers for the reaction of guanine with monoaquated BNP3029. In the reaction of adenine with monoaquated cisplatin, stabilizing interactions between OH2 and axial NH3, and between C6-NH2 and axial Cl (Figure 5A), lead to a decreased barrier for adenine reacting with monoaquated cisplatin compared to a similar reaction of adenine with monoaquated BNP3029, due to the lack of hydrogens on the substituted cyano ligands.
Again, with no involvement of either NH3s or substituted cyano ligand (axial or equatorial), the transition state geometries (gasphase) for the reaction of thiol/thiolate with monoaquated cisplatin or monoaquated BNP3029 indicate that only the leaving OH2 and the incoming thiol/thiolate are involved in leading to an early (in the case of BNP3029, Figure 6B and 7B) or slightly late (in the case of cisplatin, Figure 6A and 7A) TS, thereby leading to similar barriers (albeit slightly higher in the case of BNP3029) for thiol/thiolate attack. Our computational calculations indicated novel properties in silico for BNP3029 and importantly, in vitro IC50 evaluations of BNP3029 in comparison to cisplatin and oxaliplatin also indicated superior IC50 data for BNP3029 (Table 5) in a variety of wild-type and resistant human cancer cell lines.
Ab initio geometry optimizations (gas- and solution-phase) using the recently Published Date: energy-consistent pseudo-potentials and correlation consistent basis set for platinum and cc-pVDZ basis set on ligand atoms of cisplatin, oxaliplatin, and BNP3029 (a substituted cyano ligand-based platinum analogue) within the density functional formalism were conducted. The optimized geometries compared very well with the available experimental data for cisplatin and oxaliplatin. Transition state geometries for the nucleophilic attack of water on cisplatin and BNP3029, as well as the TS geometries for the nucleophilic attacks of guanine, adenine, thiol and thiolate on their monoaquated derivatives were also determined. Our computed data indicate that in terms of water attack both cisplatin and BNP3029 have similar activation energy barriers; the barrier for the attack of guanine and adenine was increased for monoaquated BNP3029 compared to that of monoaquated cisplatin. However, the barriers for a thiol/thiolate attack on monoaquated cisplatin and monoaquated BNP3029 were similar.
In vitro, BNP3029 had more potent activity in a variety of human cancer cell lines compared to cisplatin and oxaliplatin. BNP3029 also had potent activity in cell lines traditionally resistant to cisplatin and oxaliplatin.
NCI-H23 and NCI-H838 were developed in the laboratory of Dr. Herbert Oie and were used pursuant to a license obtained from the Public Health Service/ National Institutes of Health. We thank Dr. Yusef Rustum, Dr. W. Dalton, Dr. EGE de Vries and Dr. C. Meijer for gifts of other cell lines described in the materials and methods. We thank Joe Zdanowicz for preliminary work in developing proprietary platinum resistant cell lines.