Journal of Physical Chemistry & Biophysics
Open Access

Derivation of Special Relativity Transformation Formulas without Time Delay Effect and Length Contraction Effect

Sheng Qin^{* *}Correspondence: Sheng Qin, Department of Economics, Southwestern University of Finance and Economics, Chengdu,, China, Email:

Author info »

Introduction

In the past, many physicists have proposed many novel and simple methods to deduce the conclusions of special relativity. For example, Shimeng, [1], proposed a characteristic deduction method of the relationship between mass and velocity, but retained the time and coordinate transformation functions; Yonggang, et al. [2], trying to use space-time algebra to deduce the velocity transformation formula of relativity theory is more of a formal transformation; Youshan, et al. [3], in their article “New derivation of special relativity without assumption of invariant speed of light”, based on the mechanical model a method that does not require the assumption of constant speed of light, but still requires the assumption of time transformation. There are also some scholars who use the relativistic time and length transformation relationship to deduce the Doppler frequency shift effect, such as Junling, et al. [4], Bingkun, et al. [5].

However, the inference methods in the above articles still retain the time transformation and length transformation formulas of relativity. The new method used in this paper does not need to rely on the relativistic time transformation and length transformation, but only relies on the traditional conservation of momentum and energy, and the following transformation formula of the special theory of relativity can be deduced: The relativistic Doppler frequency shift formula, relativistic dynamic mass formula, and relativistic velocity transformation formula.

And more importantly, this paper proves that the relative motion speed of the object in the Doppler relativistic frequency shift formula is actually relative to the speed of the object after the photon is emitted, not the speed before the emission. In the calculations of past experimental studies, this point has been ignored [6], which makes up for the deficiency of the relativistic Doppler frequency shift formula.

This paper finds a new way to derive the special relativity transformation formula, which does not need to rely on the time transformation and length transformation formulas. The author believes that only based on a rigorous experimental basis is a solid foundation for constructing a physical theory.

The biggest difference between this article and the special theory of relativity:

There is no need to assume that length shrinks, time becomes slower, or even the speed of light remains unchanged. This paper is based on the same physical postulates as the laws of physics in the inertial system.

The basis of the theory is based on the mass-energy equation which has been strictly proved by experiments E=mc² [7-11]. Energy Formula of Planck photon E=hω and momentum formula P=hω/c (checked by the Compton scattering experiment [8]).

The relativistic Doppler frequency shift effect is a direct conclusion of Planck’s formula and mass-energy equation, not a new assumption.

In another article, the authors demonstrate that Einstein’s assumption of simultaneity is problematic [12], which we discard in this article.

Literature Review

Proof of the transformation formula of special relativity

Proof of relativistic Doppler frequency shift formula: First, based on the Planck formula E=hω and P=hω/c (E is the energy, P is the momentum, h is Planck’s constant, ω is the photon frequency). We prove two theorems:

Theorem 1: The red-shift or blue-shift Doppler effect of photons emitted from each other between two relatively moving objects A and B, the relative velocity of the two objects A and B in the corresponding photon frequency transformation formula, refers to the object The relative motion speed of A and B after A or B emits a photon.

Prove

As shown in Figure 1, we assume that a black box is stationary in free cosmic space, we emit a photon α with frequency ω from one side of the black box, and it is absorbed on the other side of the black box. We know that the black box as a whole will maintain momentum and energy conservation and will eventually continue to remain at rest.

Figure 1: Photon emission and absorption in a stationary black box.

Then we can reason; due to the conservation of momentum between the black box and the photon, after the black box emits photon α and before the photon α is absorbed by the other side, the black box will acquire a velocity V (relative to the opposite direction of the photon motion), then it means that the black box is no longer in a static state when the photon α is absorbed, but has a relative velocity V.

We assume that the Doppler blue-shift formula is: .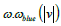

where 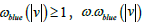is the frequency after the blue-shift of the photon; we assume that the Doppler red-shift formula is:.

where 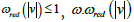is the frequency after photon red-shift.

And: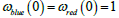

If the photon frequency ω is relative to the static state before the black box is emitted, then when the black box absorbs the photon α, a blue-shift effect will be obtained because the black box has a relative velocity V, and the frequency ω of the photon α will be blue-shifted as: .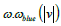

Then, according to Planck’s formula, the black box obtains a momentum relative to the photon when it is emitted: P₁=hω/c; when it absorbs the photon, it obtains another momentum due to the Doppler blue shift effect: 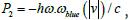obviously P₂>P₁, the momentum of the black box is not conserved before and after the photon α is emitted, which clearly violates the law of conservation of momentum.

Therefore, only assuming that the frequency ω of the photon α is actually the state after the photon is emitted relative to the black box and the speed V is obtained, then for the photon α , when it is absorbed by the other side of the black box, it is equivalent to being absorbed by the object in a relatively static state. At this time, the frequency of the photon α when it is absorbed is still ω due to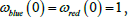 the relative motion speed between the emitting object and the absorbing object is 0. At this time, the black box will maintain momentum before and after the absorption and energy conservation.

Therefore, when we calculate the formula of Doppler red-shift or blue-shift, 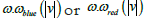the V in actually refers to the relative velocity of A and B after the relative motion objects A or B emits photons, rather than the relative velocity of A and B before the emission of the photon.

Therefore, if we use high-speed moving particles to radiate photons to test the correctness of the Doppler frequency shift formula, then the velocity V used in our calculation should be the speed after the particles radiate photons, not the speed before high-speed particles radiate photons.

Hence proved.

This result is different from that in Einstein’s special theory of relativity [9], which makes up for the deficiency of the Doppler shift formula in the special theory of relativity and answers the premise of the Doppler shift formula more rigorously.

Theorem 2: Two objects move toward or away from each other at the same speed V, and the Doppler blue-shift and red-shift effects are reciprocal. Suppose objects A and B move towards each other at speed V, and emit photons of frequency ω from A to B, and assume that their blue-shifted frequency is 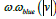; then when A and B move away at speed V, their red-shift frequency will be 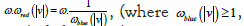is a velocity- dependent Doppler shift function).

Prove

As shown if Figure 2; suppose an object A moves in the direction of the X-axis at a speed V relative to the ground observer, and A first emits photons of frequency ω to the ground P₁ in front of the movement. At this time, because of the blue-shift effect, it is assumed that the frequency of the photons received by the ground observer at P₁ is for . According to the conclusion of the previous “Theorem 1”, we know that v1 at this time refers to the movement speed of A relative to the ground after A emits photons.

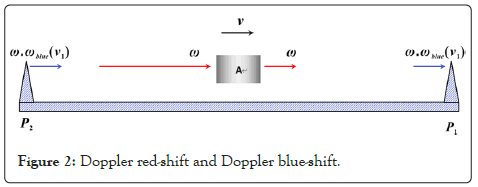
Figure 2: Doppler red-shift and Doppler blue-shift.

After receiving the photon at the ground P₁ in front, the ground observer emits a photon with the same frequency from the rear P₂ to the rear of A, and then absorbed by A. At this time, since object A moves away from the direction of photons emitted by P₂ on the ground behind with v₁, a red-shift effect will occur, and the frequency of photons absorbed by A will become:

Assuming that the ground is large enough, we can ignore the speed change of the ground itself before and after the ground receives and emits photons. Then for a ground observer, after receiving a photon of frequency and transmitting a photon of frequency , the momentum and energy of the ground as a whole will remain unchanged (because the ground observer a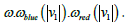bsorbs and emits the same frequency photons in opposite directions).

Then at the same time, before the object A emits photons of frequency ω and absorbs photons of frequency 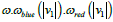, the velocity V of object A relative to the ground and the mass (overall energy) of A will remain unchanged, that is, the momentum of A and the energy will remain the same.

Therefore, from the perspective of A, it is necessary to keep the momentum and energy before and after the emission and absorption of photons unchanged: It must be that a photon of frequency ω is first emitted to the front P₁, and then absorbed from the rear P₂ of the movement. Only in this way can the photon with frequency ω maintain the conservation of its own momentum and energy before and after. We assume that its redshift formula is 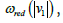hen there must be the following relationship:

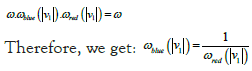
Hence proved.

Theorem 3: The relativistic Doppler red-shift formula is: 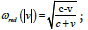The blue- shift formula is: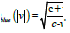

Prove

We continue to do the following thought experiment, as shown in Figure 3; suppose an object A moves along the X-axis with a velocity V relative to the observation system S, and at a certain moment A simultaneously emits two equivalent photons to the front and back of its motion, assuming that both photons have frequencies ω.

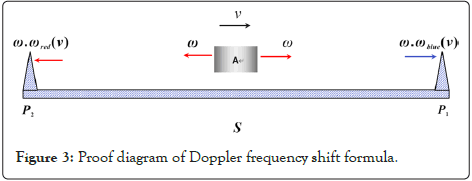
Figure 3: Proof diagram of Doppler frequency shift formula.

The two photons are observed by the observation system S where the ground observer is located. For A, the velocity V relative to the ground before and after the emission is unchanged (the total momentum of the photons emitted before and after is P=0), the only decrease is the overall mass of A (we can assume that these two photons are a pair of symmetric photons produced by the annihilation of a pair of positrons and electrons moving together with A, and the only thing that decreases for A before and after emission is the mass, the velocity V of object A relative to the ground before and after launch remains unchanged). The same seems to be the case for ground observers of the S system, the relative velocity V of A remains unchanged before and after launch.

Then at P1 in front of the movement, the photons observed by the ground observer have a blue-shift. Assuming that the frequency of the detected photon is 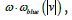then according to the conclusion of our previous “Theorem 2”, the red-shifted light detected at P₂ behind the ground observer the photon frequency is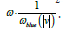

Then from the point of view of the ground observer of the S system, assuming that the mass reduced by the object A is 2Δm, it will be equal to the sum of the energies of the photons observed by the ground observer (note that 2Δm is a moving object from the ground observer’s point of view here), which is derived from Planck’s formula:

The reduced momentum ΔP of A observed by the S system ground observer is:

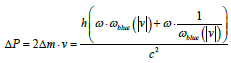………. (1)

At the same time, according to the Planck formula of photon momentum, the momentum is equal to the sum of the momentums of the two front and rear photons observed by the ground observer (because the total momentum obtained by the ground observer is composed of only the sum of the momentums of the two front and rear photons), Since the two photons are in opposite directions, the relationship is as follows:

…………. (2)

There is only one unknown quantity 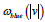in the above equation system formed by equations (1) and (2), we get the following equation:

………….. (3)

We obtain the Doppler blue-shift formula by solving equation (3) as:

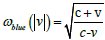
According to the conclusion of the previous “Theorem 2”, we get the formula of Doppler redshift:

Thus, we have the relativistic Doppler shift formula.

Hence proved.

Doppler shift in other directions

As shown in Figure 4, if the two photons with frequency ω are emitted in the direction of the A system and the angle between the X axis is θ, it is assumed that the photon pair is emitted symmetrically, and it is assumed that the angle at which the photon is detected by the S system in front of the motion is θ₁, and the photon frequency is ω₁; The angle at which the photon is detected is θ₂ and the photon frequency is ω₂ (assuming are in the range θ, θ₁, θ₂ are in the range

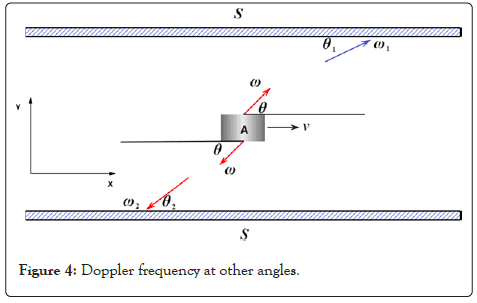
Figure 4: Doppler frequency at other angles.

Since the photons emitted by object A are symmetrical, the velocity V of A before and after the emission is unchanged, and the momentum in the Y-axis direction is also unchanged..

Then photons ω₁ and ω₂ will satisfy the following momentum and energy conservation relations:

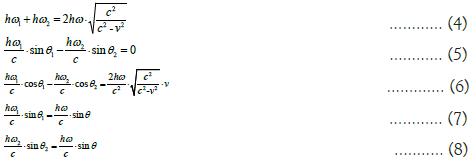
Where, equation (4) refers to the conservation of energy from the perspective of the S system; equation (5) refers to the conservation of momentum in the Y-axis direction from the perspective of the S-system, and the total momentum in the Y-axis direction is 0; equation (6) it refers to the conservation of momentum in the X-axis direction from the perspective of the S system.

Both the aberration formula and the Doppler frequency shift formula in the theory of relativity will be obtained from the solution of the above formula: (4-8). Where, equations (7) and (8) refer to the conclusions of the transformation relationship of the special theory of relativity (This conclusion can be derived based on the following velocity transformation formula, but it will not be repeated in this paper) [10].

Solving equations (4-8) is easy to get:

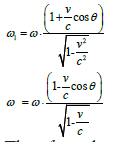
Therefore, the aberration formula is:

The above conclusion is equivalent to the conclusion in Einstein’s theory of relativity, but the expression is different:

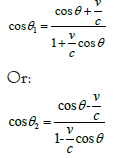
Proof of the dynamic mass formula of relativistic moving objects

Reasoning: From the Doppler frequency shift formula, we can further infer that the dynamic mass formula m(v) of the moving object is: 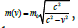where m₀ is the static mass of the object, and V is the moving speed of the object.

Prove

Assume that two positive and anti-matter masses of m0 are moving relative to the ground S observation system in the direction of the X-axis of the S system at a speed V, and the positive and anti-matter annihilate each other to generate two photons of frequency ω, which are respectively directed towards the X-axis of the S system. It is emitted in two directions, where the photon frequency ω is obtained from the mass-energy equation 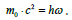Assume that the dynamic mass of matter and antimatter is m₀(v) in the view of the S-system ground observer.

After the annihilation of positive and negative matter, two photons with frequency ω are respectively emitted towards the front and rear directions of the motion. According to the conclusion of “Theorem 3” above, the total photon energy E received by the ground observer is:

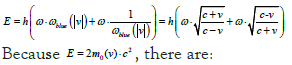
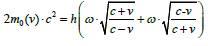………… (9)

Solving equation (9) yields:

This is the dynamic mass formula in the chivalrous theory of relativity.

Hence proved.

Proof of the relativistic velocity transformation formula

The first way of proof: As shown in Figure 5, in the view of the ground observer S system, both objects A and B move along the X-axis direction of the S system, where the speed of B relative to the S system is V, and the speed of A relative to the S system is u (u moves in the negative direction of the X axis, so it has a negative sign).

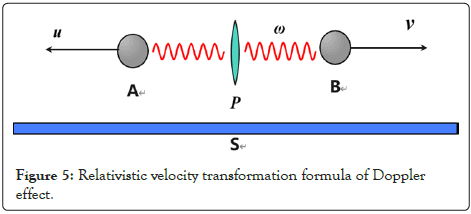
Figure 5: Relativistic velocity transformation formula of Doppler effect.

Now we ask for the relativistic velocity transformation formula: What is the speed of motion of A in the view of the B system?

We assume that B emits a photon of frequency ω to A, which is absorbed by an object P in the S system between A and B, and assume that the photon red-shifted frequency is ω₁ when the photon absorbed by the S system. Then the S-system object P emits a photon of the same frequency ω₁ to A from the other side. We assume that the frequency of the photon observed when A absorbs the photon is ω₂ (we assume that the masses of A and B are relatively high relative to the photon is large, and the difference in motion speed before and after emitting photons and absorbing photons can be ignored).

From the point of view of the S system, the energy of the absorbed and emitted photons of frequency ω₁ is the same and in opposite directions, so both the momentum and the energy of the S system remain unchanged.

At this point, the whole process is equivalent to directly emitting a photon of energy ω from B and absorbed by the A object. According to our previous conclusion of “Theorem 3”, the photon frequency ω₂ received by A is:

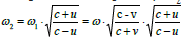………… (10)

(Note: Since the velocity u is moving in the negative direction of the X axis, its sign is negative.)

If we assume that in the view of B, the speed of A’s movement is u' (the direction of movement of u' is the negative direction of the X-axis of B), then the above formula (10) will become:

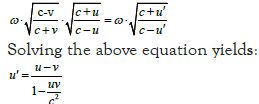
This is the velocity transformation formula in the special theory of relativity. The proofs in other motion situations can refer to the above proofs.

Hence proved.

Of course, we can derive it in another way as follows and obtain more useful conclusions.

The second way of proof

• Let’s do the following thought experiment: Assuming that the static mass of the object A is mA, A moves in the X-axis direction with a velocity vA relative to the S system ground observer (the X-axis direction of the A system is parallel to the ground X-axis). There are two bodies B and C with static mass 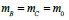. Their velocity relative to the ground S system is unknown, but their velocity relative to object A is V and -V, respectively. Now, what is the speed of the objects B and C in the view of the ground observer S system? (we assume that their velocities are v₁ and v₁ respectively in the view of the S system), as shown in Figure 6, we can also call the ground reference system for the S system, the reference frame of the object A is the S' system or the A system:

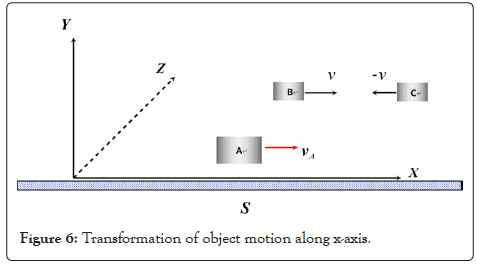
Figure 6: Transformation of object motion along x-axis.

There are two potential hypotheses here, which we will conversely prove correct through the speed-shifting argument:

The static mass of any object is consistent in any inertial reference frame, for example, we observe the mass of electron e in any inertial frame, and it will have the same properties.

Assuming that the objects in the two reference frames do not feel any acceleration, their relative velocity relative to the other frame of reference will remain unchanged (we will not discuss the explanation of the equivalence principle of gravitational fields for now, which will explained in the next article).

We assume that the moving directions of objects B and C are both in the direction of the X-axis. From the perspective of the A system, B and C collide with velocities V and -V respectively and merge together after the collision. Then from the point of view of system A, since B and C have equal masses and opposite speeds, B and C will remain stationary relative to system A after collision. At this time, in the view of the ground observer S, B and C will move along the X-axis with a velocity v_A relative to the ground after the collision.

Then in the view of the ground observer S system, due to the energy conservation before and after the collision of B and C, the total mass before the collision is:

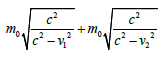………….. (11)

From the point of view of the A system, B and C are stationary relative to the A system after the collision, and the static mass after the B and C collision is the sum of the dynamic masses before the collision seen by the A system:

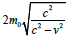
The static mass is also applicable to the ground observer S system, so from the ground observer S system, the total mass of B and C after collision is (dynamic mass):

…….. (12)

The above equations (11) and (12) are equal, so we obtain the first equation:

Equation 1:

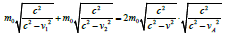
Similarly, we can get a momentum conservation equation:

Equation 2:

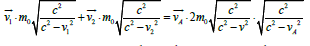
Since we assume that the motion directions v₁, v₂, v, v_A are all along the X-axis direction of the S system and the A system, then we directly substitute the following relativistic velocity transformation formula for verification.

According to the velocity transformation formula of special relativity [3], the X-axis velocity component of B is:

………… (13)

The X-axis velocity direction of C is:

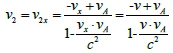………… (14)

It is easy to verify that the two equations (13) and (14) are the solutions of “equation 1 and 2” by substituting (13) and (14) into the above “equations (1) and (2)”.

Conclusion: The velocity transformation formula of special relativity is valid in the direction of X axis.

Discussion of the more general case: As shown in Figure 7, Objects B and C have the same static mass, and B and C collide and combine together. In the view of system A, the velocities of objects B and C are v and v' respectively. The three-direction velocity components of the X, Y, and Z axes of B and C are (v_x, v_y, v_z) and (v_x, -v_y, -v_z), respectively.

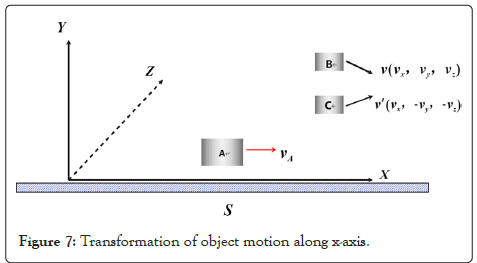
Figure 7: Transformation of object motion along x-axis.

That is to say, from the point of view of the A system, the movement speed of the two objects after the collision will become v_x and move along the X axis. Since vx remains unchanged before and after the collision, obviously for the S system, the velocity component of the X-axis direction before and after the collision of objects B and C is also unchanged.

Assuming that the moving velocities of the two objects B and C are v₁ and v₂ respectively in the S system, we assume that their velocity components in the directions of the three coordinate axes are: (v_1x, v_1y, v_1z) and (v_2x, v_2y, v_2z), then there are:

Assuming that the static mass before the collision between B and C is m₀, and the static mass after the collision is m1, according to the conservation of energy before and after the collision, in the view of the A system, there are:

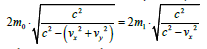
where m₁ is the static mass of objects B and C after collision, so the static mass m1 of objects B and C after collision is obtained as:

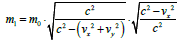………… (15)

According to our previous conclusion on the velocity transformation formula in the X-axis direction, in the S system, there are:

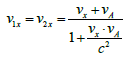………… (16)

Due to the conservation of energy before and after the collision, in the S system, there are:

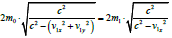………… (17)

where,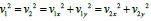

Substituting the above formula (15) into the formula (17), we get:

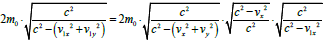
Then substitute equation (16) into the above equation, and solve the equation after expansion to obtain:

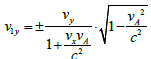
Taking into account the consistency of the directions of v_y and v_1y, the positive sign is taken, which is the component of Einstein’s special relativity velocity transformation formula in the Y-axis and Z-axis directions.

Hence proved.

Therefore, we have proved the relativistic velocity transformation formula through the above thought experiment; at the same time, we have also proved the correctness of the previous two assumptions: The static mass of the object in the inertial frame is unique, otherwise the velocity transformation formula will not hold; at the same time, we also The premise of proving that the relative speed of motion between two objects changes is the force.

Discussion

Explanation of the above derivation

The derivation of the relativistic transformation formula in this paper is based on the assumption of inertial system, mass-energy equation, energy conservation, momentum conservation, Planck’s energy-frequency formula, etc. The core essence of the transformation formula is actually implied by the inertial system. The transformation relationship between the conservation of energy and momentum. We did not use the time transformation relationship and length transformation relationship of relativity, and also deduced the entire relativistic transformation formula.

This proves that the core of the relativistic transformation formula is actually the observance and transformation of momentum conservation and energy conservation.

We cannot infer that there is a time slowing effect and a length contraction effect between the two inertial frames of relative motion because of the speed change; otherwise, we need to draw the same conclusion: Because of the angular effect of relativistic aberration, we consider the object the spatial position of the real changes has taken place. The relativistic effect between inertial frames is only based on the principles of energy conservation and momentum conservation, and a transformation formula that the inertial frame space is relatively independent.

Thus, through another paper and this paper [12], we rigorously demonstrate that Einstein’s definition of simultaneity can be questionable and, in fact, redundant.

Conclusion

In this paper, based on the mass-energy equation and Planck’s energy and momentum formula, without the hypothesis of time slowing down and length contraction, only based on the conservation of momentum and energy, the Doppler red shift and blue shift formula, the kinetic mass equation and the velocity transformation formula of relativity are strictly deduced and proved.

In addition, this paper also supplements a defect of special relativity in demonstrating the Doppler frequency shift formula through rigorous derivation: We have proved that the relative velocities of two objects A and B in the photon frequency conversion formula actually refer to the relative velocity of A and B after object A or B emits photons. In this way, the Doppler frequency shift formula can be measured and calculated more rigorously in the experiment.

This paper also proves that in the special theory of relativity, the two conclusions of time delay effect and length contraction effect are superfluous. Then our conclusion in another article may be correct: Simultaneity is possible in different inertial frames, and the space is relatively independent. In this way, we can make more reasonable explanations for phenomena such as quantum entanglement at a distance and cosmic inflation.

References

1. Shimeng F. The characteristic derivation method of the relationship between mass and velocity in the special theory of relativity. Univ Phys. 2021;40(6):32-35.

   [Crossref] [Google Scholar]

2. Yonggang W, Xuecheng C, Guijun J, Feng G, Hong Z, Wenli Z. Deriving the arbitrary direction acceleration transformation of special relativity using space-time algebra. Univ Phys. 2019;38(4):8-12.

   [Crossref] [Google Scholar]

3. Dai Y, Song X. New derivation of special relativity without the assumption of constant speed of light. J Zhejiang Univ. 2016;43(2):247-251.
4. Junling L, Rongbao W. Simple derivation of Doppler effect formula. Univ Phys. 2005;24(8):25-29.
5. Bingkun G, Fenglin W. Simple derivation of the Doppler effect of relativistic theory. Univ Phys. 2003;22(8):15-16.
6. Mandelberg HI, Witten L. Experimental verification of the relativistic Doppler effect. J Opt Soc Am. 1962;52(5):529-535.

   [Crossref] [Google Scholar]

7. National Institute of Technology, USA. Einstein's special theory of relativity confirmed by precise experiments in the United States. Bull Phys. 2006:64.
8. Compton AH. The spectrum of scattered X-rays. Phys Rev. 1923;22(5):409.

   [Crossref] [Google Scholar]

9. Einstein A. Translated by Yang Zi. Theory of relativity. Beijing: Taihai Publishing House. 2017.
10. Kaihua Z, Ximou C. Electromagnetics. Beijing: Higher Education Press. 2021.
11. Rainville S, Thompson JK, Myers EG, Brown JM, Dewey MS, Kessler EG, et al. A direct test of E=mc2. Nature. 2005;438:1096-1097.

    [Crossref] [Google Scholar]

12. Sheng Q. A possible explanation for the twin paradox and action at a distance. 2022.