Journal of Thermodynamics & Catalysis
Open Access
ISSN: 2157-7544
ISSN: 2157-7544
Editorial - (2013) Volume 4, Issue 2
From thermodynamics point of view, in this era of aiming at energy conservation and sustainability, we need to develop more accurate ways to design thermal power, cooling and heat pump cycles. It has been the general practice in thermodynamic analysis of cycles to use the Carnot efficiency and Carnot coefficient of performance (COP) which are the highest upper bound to efficiency and COP of cycles. In the present report through the application of the 2nd law of thermodynamics for irreversible processes, which results in the general inequality relation for the entropy production, we have introduce new upper- and lower-bounds to the efficiency of thermal power cycles and COP of cooling and heat pump cycles. The resulting upper- and lower-bounds are closer to the actual efficiency and COP of cycles. That allows us a more precise design of cycles and the choice of cycles’ working fluids.
Keywords: COP; Efficiency; Entropy production; Lower- and upperbounds; Rankin cycle; Absorption cycle
In the analysis of thermal power, cooling and heat pump cycles it has been the general practice to use the ideal Carnot efficiency and coefficient of performance (COP). However, due to the ideal nature of Carnot cycle the resulting efficiency and COP relations are the highest upper bound to the real values of efficiency and COP. The marvelously simple and highly cited Carnot cycle and its related efficiency and COP relations [1] were proposed at a time when principles of thermodynamics were at their infancy. The genius Nicolas Léonard Sadi Carnot who proposed his cycle in 1823 recognized the need to develop his theory independent of the knowledge about properties of working fluids, at a time of lack of any accurate thermodynamic property data for such fluids.
Presently, thanks to extensive research and development in thermodynamics of irreversible processes [2,3] and our knowledge about accurate thermodynamic properties of materials (see [4] and many data-books published by IUPAC, JANAF, NIST, TRC, etc.). Since the time of Sadi Carnot, we can now develop upper bounds to efficiency and COP which are much closer to their real values than those of Carnot cycle values. Also the methodology introduced in this report has allowed us to generate lower bounds to efficiency and COP.
In this report we demonstrate, through the application of the 2nd law of thermodynamics for irreversible processes, it is possible to derive both upper- and lower-bounds to efficiency and COP of cycles. The upper bounds derived and reported here are lower upper bounds than the Carnot cycle values. Availability of both, lower and upper bounds to efficiencies and COPs of cycles will allow us to acquire a better understanding about the real performance of thermodynamic cycles.
According to thermodynamics of flow processes for an open system with incoming and outgoing flows the first law of thermodynamics can be written in the following form [2,3,5]:
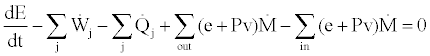 (1)
(1)
In this equation  is the rate of energy accumulation in the system,
is the rate of energy accumulation in the system,  and
and  are the rates of work and heat added to the system, respectively. P is the pressure, v is the specific volume, e is the energy per unit mass and
are the rates of work and heat added to the system, respectively. P is the pressure, v is the specific volume, e is the energy per unit mass and 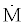 is the mass flow rate of incoming and outgoing flows.
is the mass flow rate of incoming and outgoing flows.
The second law of thermodynamics for an open flow process takes the following form [2,3,5]:
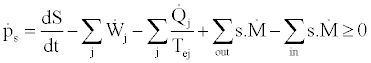 (2)
(2)
In this equation ps is the rate of production of entropy in the system, 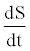 is the entropy accumulation rate in the system, s is the entropy per unit mass of the incoming and outgoing flows to and from the system, and Tej is the temperature of the external heat source or sink
is the entropy accumulation rate in the system, s is the entropy per unit mass of the incoming and outgoing flows to and from the system, and Tej is the temperature of the external heat source or sink  . In what follows we apply Eq.s (1) and (2) to develop the upper and lower bounds to the efficiency of thermal power cycles and COP of cooling / heat pump cycles.
. In what follows we apply Eq.s (1) and (2) to develop the upper and lower bounds to the efficiency of thermal power cycles and COP of cooling / heat pump cycles.
In Figure 1 we demonstrate a basic Rankine thermal power cycle as it is well-known:
For a basic Rankine thermal power cycle, Figure 1, considering it to be in the steady state and steady flow conditions, application of the second law for the boiler produces the following inequality for 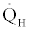
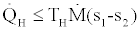 (3)
(3)
Application of the 2nd law for the condenser gives us
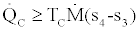 (4)
(4)
In the above two relations equality signs are for the reversible cases and inequality signs are for the irreversible cases. As a result of the above two inequalities we get,
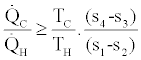 (5)
(5)
Considering that the actual efficiency of the cycle is defined as
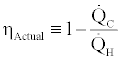 (6)
(6)
We conclude from inequality (5) the following upper bound for efficiency
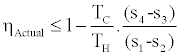 (7)
(7)
The upper bound of efficiency as it is shown by Inequality (7) is lower than the Carnot efficiency, i.e.
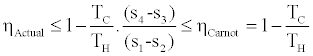 (8)
(8)
This is because (s4-s3) ≥ (s1-s2) as it is shown in Figure 2 and the fact that the Carnot efficiency depends only on the temperatures of the heat source and heat sink and it is independent of the working fluid characteristics.
In order to derive the lower bound to the efficiency, we use the first law for the turbine which gives us
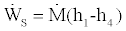 (9)
(9)
From relations (3) and (9) we conclude
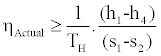 (10)
(10)
Finally we have the following upper and lower bounds to the actual efficiency of the cycle:
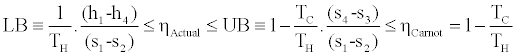 (11)
(11)
The above inequalities can be used to calculate the upper and lower bounds of the efficiency of a cycle. We should add, the efficiency of the cycle according to the first law of thermodynamics is in the following form:
 (12)
(12)
Obviously
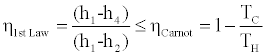 , (13)
, (13)
and we may expect η1st Law to be larger than ηActual, but there is no theoretical indication of the relative values of η1st Law and the upper bound to efficiency, i.e. 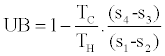
The above inequalities can be used to calculate the upper and lower bounds of the efficiency of a Rankine thermal power cycle. In what follows we show two expels of applications of the above expressions of efficiencies.
Example 1:
As the first example we consider the data of the cycle shown on Figure 2 in which water is the working fluid with TH=500°C=773K, TC=100°C=373K, h1=3460, h2=1320, h3=515, h4=2650 [kJ/kg], and s1=7.35, s2=3, s3=1.35, s4=7.4 [kJ/kg.K]. We calculate the following value for the Carnot, upper bound, lower bound, and first lawefficiencies:
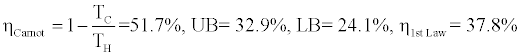
According to the above calculations 24.1% ≤ ηActual ≤ 32.9% while ηCarnot =51.7% which is much higher than 32.9%, the upper bound of efficiency of the Rankine thermal power cycle for the data of Figure 2. The efficiency based on the first law of thermodynamics η1st Law = 37.8% is still much lower than the Carnot efficiency and closer to the actual efficiency of the cycle.It is worth mentioning that the actual efficiency of Rankin thermal power cycles at best possible conditions has not exceeded much above 40%.
Example 2:
We would like to search for the best working fluid which can be used in a Rankine power cycle operating between temperatures of 40°F (4.4°C) and 80°F (26.7°C). A real life example of this cycle is the Ocean Thermal Energy Conversion (OTEC) system in which the hot source is the surface ocean water and cold source is the water about 1000 meters deep in the ocean [6]. We bound our search here to pure working fluids even though mixtures can also be considered as possible candidates for such application. The Carnot efficiency of the cycle is 7.41% which is independent of the nature of working fluid.By considering the vapor coming out of the boiler to be a saturated vapor at 80°F and application of expressions for the upper bound, lower bound and 1st law efficiency of the cycle, the following table is produced for eight different candidate working fluids.
Experimental data needed to produce this table were taken from Perry’s Handbook [7]. Because of the low temperature difference between the heat source and heat sink all the efficiencies are rather small. But it is clear that among all the fluids investigated 1, 3, Butadiene will be a better working fluid from the thermodynamics point of view. It is worth noting that by the mere use of the Carnot cycle efficiency there is no way to compare capabilities of the working fluids (Table 1). Comparison of efficiencies of various working fluids which may be used in an OTEC Rankine power cycle operating between temperatures of 40°F (4.4°C) and 80°F (26.7°C).
| Working Fluid | % Efficiency | |||
|---|---|---|---|---|
| Lower Bound | Upper Bound | First Law | Carnot | |
| Ammonia | 1.41 | 1.67 | 1.42 | 7.41 |
| 1,3, Butadiene | 6.14 | 6.56 | 6.17 | 7.41 |
| Methyl Chloride | 2.32 | 2.63 | 2.33 | 7.41 |
| Propane | 5.27 | 5.64 | 5.28 | 7.41 |
| Refrigerant # 12 | 5.63 | 5.99 | 5.65 | 7.41 |
| Refrigerant # 21 | 4.34 | 4.65 | 4.36 | 7.41 |
| Refrigerant # 504 | 1.76 | 2.00 | 1.76 | 7.41 |
| Water | 1.60 | 1.75 | 1.60 | 7.41 |
While the upper bound shown by Inequality (7) is dependent on the properties of the working fluid in addition to temperatures of heat source and heat sink.
Table 1: Comparison of efficiencies of various working fluids which may be used in an OTEC Rankine power cycle operating between temperatures of 40°F (4.4°C) and 80°F (26.7°C).
In what follows we consider two different cooling and heat pump cycles. One is the Rankine cycle, and the other type is the absorption cooling cycle [8,9].
In Figure 3 we demonstrate a basic Rankine cooling and heat pump cycle as it is well-known:
For the basic Rankine cooling and heat pump cycle, Figure 3, considering that to be in the steady state and steady flow conditions, application of the second law of thermodynamics for the evaporator (refrigerator) and the condenser produces the following inequalities:
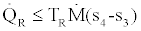 , (13)
, (13)
and
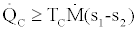 (14)
(14)
In the above two relations equality signs are for the reversible cases and inequality signs are for the irreversible cases. Considering that the coefficient of performance (COP) of the cycle is defined as
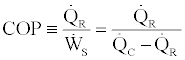 (15)
(15)
and knowing that from Relations (13) and (14)
 , (16)
, (16)
we get the following upper bound for the cycle COP
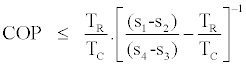 (17)
(17)
The upper bound of COP as shown by the right side of (17) is lower than the Carnot COP, i.e.
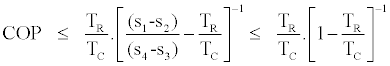 (18)
(18)
This is because (s1-s2)≥ (s4-s3) as it is shown in Figure 4 and the fact that Carnot COP depends only on the temperature of the heat source and heat sink and it is independent of the working fluid characteristics.
An example of stages of ammonia phase transitions going through the basic Rankine cooling cycle of Figure 4. Stage numbers 1, 2, 3, 4 on this figure correspond to the same stages shown in Figure 3.
In order to derive a lower bound to the COP for this cycle we use the first law for the compressor which gives
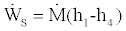 , (19)
, (19)
we also know that
 (20)
(20)
Now by dividing the left side of (20) by right side of (19) we get,
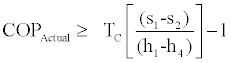 (21)
(21)
The right side of (21) provides us with the lower bound of the COP of the cycle.
Finally we have the following upper and lower bounds (UB, LB) to the actual COP of the cycle:
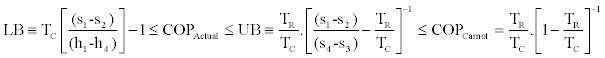 (22)
(22)
The above inequalities can be used to calculate the upper and lower bounds of the COP of a Rankine cooling cycle. However, the COPof the cycle according to the first law of thermodynamics is in the following form:
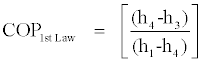 (23)
(23)
Example
Inequalities Eq. (22) can be used to calculate the upper and lower bounds of the COP of a Rankine cooling cycle. As an example for the data of the cycle shown on Figure 4 in which TR=-20°C =253K, TC=60°C =333 K, h1=1775, h2=480, h3=480, h4=1410 [kJ/kg], and s1=5850, s2=1950, s3=2150, s4=5850 [J/kg.K], we calculate the following value for the Carnot, upper bound, lower bound, and first law efficiencies:
LB = 2.558 ≤ COPActual ≤ UB = 2.581≤ COPCarnot = 3.163
and
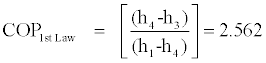
According to the above calculations 2.558 ≤ COPActual ≤ 2.581while COPCarnot=3.163 which is much higher than 0.2.581, the upper bound of actual COP of the Rankine cooling cycle for the example of Figure 4.
In Figure 5 we demonstrate a basic absorption cooling and heat pump cycle as it is well-known:
The coefficient of performance (COP) of the absorption cooling cycle, Figure 5, is defined as the ratio of cooling effect by the evaporator and the heat input to the generator,
 (24)
(24)
According to the first law of thermodynamics the following balance equation holds for the whole cycle,
 (25)
(25)
According to the second law of thermodynamics the following inequality can be written for the cycle,
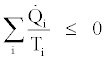 (26)
(26)
or
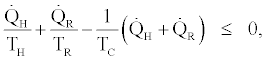 (27)
(27)
Assuming the work input to the liquid pump negligible as compared to the other terms. Now by consideration of definition of COPActual, Eq. (24), the above inequality can be rearranged to the following form
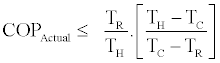 (28)
(28)
This upper bound to COPActual is the Carnot cycle COP. According to the first law of thermodynamics for flow systems the following relations hold between the heat and work transfer rates and the properties of the working fluids in a steady state steady flow condition:
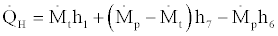 (29)
(29)
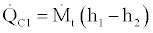 (30)
(30)
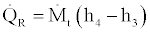 (31)
(31)
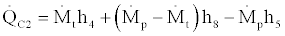 (32)
(32)
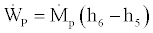 (33)
(33)
In the above equations 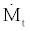 is the mass flow rate of refrigerant passing through the throttling valve (I) and
is the mass flow rate of refrigerant passing through the throttling valve (I) and 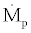 is the mass flow rate of the solution passing through the liquid pump.The following relation exist between
is the mass flow rate of the solution passing through the liquid pump.The following relation exist between 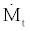 and
and 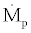
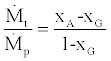 (34)
(34)
where xA is the mass fraction of refrigerant in the strong liquid phase coming out of absorber and xG is for the liquid phase coming out of the generator. In deriving Eq. (34) it is assumed the vapor coming out of the generator is pure refrigerant.
According to the second law of thermodynamics for open systems, Inequality Eq. (2), the following relation also holds the heat transfer rates and properties of working fluids in a steady state steady flow absorption cooling cycle:
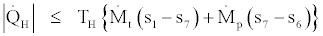 (35)
(35)
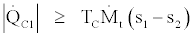 (36)
(36)
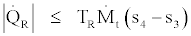 (37)
(37)
 (38)
(38)
In the above four relations equality signs are for the reversible cases and inequality signs are for the irreversible cases. By joining Eq. (35) and (37) we get,
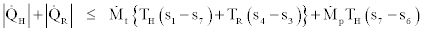 (39)
(39)
Also by joining Eq. (36) and (38) we get,
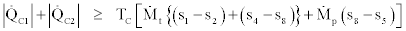 (40)
(40)
Now by assuming 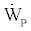 negligible as compared with the other terms in Eq. (25) we can write:
negligible as compared with the other terms in Eq. (25) we can write:
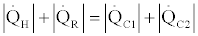 (41)
(41)
Then from relations Eq. (39) - (41) we conclude that
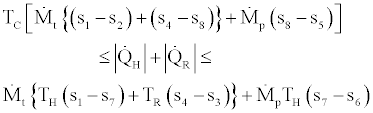 (42)
(42)
By dividing Eq. (42) by 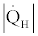 and consideration of Eq. (24) for the Figure 5: Absorption cooling cycle. definition of COP we derive the following relation,
and consideration of Eq. (24) for the Figure 5: Absorption cooling cycle. definition of COP we derive the following relation,
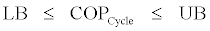 (43)
(43)
Where lower bound (LB) and upper bound (UB) of COP will have the following expressions,
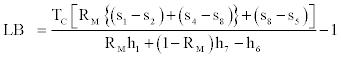 (44)
(44)
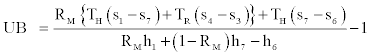 (45)
(45)
Where,
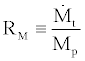 (46)
(46)
Relations Eq. (44)-(46) can be used to calculate the upper and lower bounds of COP of the cycle knowing the working fluid properties.Also with the understanding that the Carnot COP as given by the right side of Eq. (28) is the upper bound of COP regardless of working fluid it is always larger than UL as given by Eq. (45). In conclusion we can write
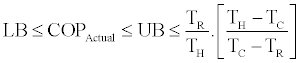 (47)
(47)
These inequalities can be used to calculate the lower bound and upper bound of the actual COP of absorption cooling cycles.
The following relations also exist for the isenthalpic expansion valve (I) and (II) in the cycle:
h2=h3 and h7=h8. (48)
By assuming the power input to the liquid pump  negligible the following relation will also hold
negligible the following relation will also hold
h2=h5 (49)
Based on the above equations the COP of the cycle based on the first law of thermodynamics alone is defined by the following relation:
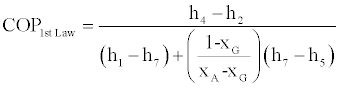 (50)
(50)
These inequalities can be used to calculate the lowerbound and upper bound of the COP of absorption cooling cycles.
Example
Solar energy as the heat source can be utilized through the absorption cooling cycle shown in Figure 5 for cooling (refrigeration and air conditioning) purposes. The major questions in the design of solar absorption cooling cycles are the choice of combined working fluids (refrigerant and absorbent) and thermal energy storage system for the cycle to continue working during evening and cloudy days. The latter subject is out of the scope of this report and the reader is referred to other literature [10,11].
The upper- and lower-bond expressions for the COP absorption cooling cycle as reported by Eq’s (44) and (45) are used in order to make comparative studies of candidate working fluid combinations of the cycle. We have reported the details of the methodsand results of various calculations of the upper- and lower-bounds of COP of absorption cooling cycle in our earlier publications [8,9,12,13]. In general the present approach has allowed us to compare various absorbentrefrigerant combinations which would have been otherwise impossible to do with the use of Carnot cycle COP calculation.
The inequalities reported in this paper can be used to calculate the lower bounds and upper bounds of efficiencies of Rankine thermal power cycles, COPs of Rankine cooling and heat pump cycles and COPs of absorption cooling and heat pump cycle. There are several advantages in using these inequalities over the Carnot upper bound values for efficiency and COP: i. We are now able to calculate, both upper and lower bounds of efficiency and COPs which are quite useful for a more proper design of power and cooling cycles. ii. In the study of specification of better working fluids for alternative power and cooling cycles such upper and lower bounds will help to choose the optimum kind of working fluid. iii. Overall, the inequalities presented in this report are the thermodynamics criteria for the optimum design of thermal power cycles and cooling and heat pump cycles from the point of view of energy conservation and sustainability.
The author would like to thank Prof. A.L. Gomez and Mr. V. Patel for their helpful comments.