Journal of Thermodynamics & Catalysis
Open Access
ISSN: 2157-7544
+44 1300 500008
ISSN: 2157-7544
+44 1300 500008
Research Article - (2017) Volume 8, Issue 1
Nowadays, polyvinyl chloride (PVC) was the most usual plastic. Thereby, to make polyvinyl chloride its monomer must be produced firstly that was called vinyl chloride (VCM). It was severely endothermic reaction that was done in an ethylene dichloride ethylene dichloride thermal cracking reactor within temperature range of 680-758°K and pressure range of 2500000 Pascal, thus this cracking reaction changed into hydrochloric acid and VCM. In production unit, monomeric chloride had the main and principal role as the core of the process of thermal cracking that occurs in the furnace. Increasing of wall temperature cause to boil gas mixture and causing pyrolysis reactions. Regarding to the simulation results showed that number of pyrolysis produced composition have maximum concentration in the length of reactor that illustrated these compositions participated in secondary reactions. Furthermore, by increasing the amount of coil outlet temperatures, the amount of formed coke will be increased. If Carbon tetrachloride considered as the chlorine radicals, it has an important role as the motivator in the cracking procedures, radicals causing an enhancement in VCM production.
Keywords: Carbon tetrachloride; Conversion factor; Coke; Coil outlet temperatures; Thermal cracking
Design and Simulation of thermal cracking reactor because of the chemical reactions in the processes and heat transfer in the furnace are very complex. Therefore, this process from the chemical reactions engineering perspective and from the perspective of transfer phenomena is highly regarded. Computational Fluid Dynamics (CFD) tools help us to investigate the development of detailed models of these reactors and by understanding the basic principles, the optimized design of reactor and by saving the cost and time performance of this equipment in a virtual laboratory can be done. In this research, investigate the proposed model of EDC cracking process in an industrial furnace and by operational data from a petrochemical unit to qualify the model and remove the reductions. To begin, by previous researches that worked on this specific occasion were collected. Then they are studied and investigated. Moreover, the reactor mathematical models were provided and solved by numerical software. Thereby, the operation of the reactor at different operating conditions investigated and sensitivity analysis of effective parameters will be done. As e result, this work could design new reactors or increasing the optimization capacity of existing reactors.
Molecular cracking
Molecular cracking was a process that used in the petrochemical industry and to reduce their hydrocarbons molecular weight by breaking their bonds. This process is the main principal of converting crude oil to useful fuels such as gasoline, diesel, jet fuel and kerosene. Thermal cracking, catalyst cracking, hydrocracking and cracking by water vapour were the most useful cracking processes in the industry. This process was done in the high temperature and pressure and without presence of catalyzer or in the low temperature and pressure with presence of catalyzer.
Geometry of thermal cracking reactor
In EDC thermal cracking process, coils containing process fluid, inside a box called furnace, are positioned horizontally. Coils placement inside the furnace shown in Figure 1 [1]. That positioned on the on both sides of the wall furnace, by burning gases of these furnaces the heat produced, that the heat transferred to the coils by convection or radiation model (Table 1). The number of intended coils to simulate 11 rows of pipes (Inconel 600) with overall length of 95 meters and length of each row tubing is 13.5 meters. Inner diameter of coils is 0.134 meter the distance between centers of the two tubes is 0.32 meter (Figures 2 and 3).
| Reactions | A[(cm3/mole) n-1s-1] | n | E (kJ/mole) |
|---|---|---|---|
| Radical reaction chain initialization | |||
| EDC → R1+R2 | 5.9 × 1015 | 1 | 342 |
| CCl4 → R1+R8 | 2.2 × 1012 | 1 | 230 |
| Radical reaction chain propagation | |||
| EDC+R1 → HCl+R3 | 1.3 × 1013 | 2 | 7 |
| EDC+R5 → VCM+R3 | 1.2 × 1013 | 2 | 34 |
| EDC+R2 → EC+R3 | 1.0 × 1012 | 2 | 42 |
| EDC+R4 → 1,1+R3 | 5.0 × 1011 | 2 | 45 |
| EDC+R6 → 1,1,2+R3 | 2.0 × 1011 | 2 | 48 |
| EDC+R7 → 1,1,1,2+R3 | 1.0 × 1011 | 2 | 56 |
| EDC+R8 → CHCl3+R3 | 1.0 × 1012 | 2 | 63 |
| VCM+R1 → R4 | 9.1 × 1010 | 2 | 0 |
| VCM+R1 → HCl+R5 | 1.2 × 1014 | 2 | 56 |
| VCM+R5 → CP+R1 | 5.0 × 1011 | 2 | 31 |
| VCM+R4 → C4H6H2+R1 | 2.0 × 1010 | 2 | 30 |
| VCM+R2 → EC+R5 | 3.0 × 1011 | 2 | 61 |
| R3 ↔ VCM+R1 | 2.1 × 1014 | 1 | 84 |
| R5 ↔ C2H2+R1 | 5.0 × 1014 | 1 | 90 |
| R6 ↔ Di+R1 | 2.0 × 1013 | 1 | 70 |
| R7 ↔ Tri+R1 | 2.5 × 1013 | 1 | 70 |
| 2C2H2+R5 → C6H6+R1 | 1.0 × 1014 | 2 | 20 |
| EC+R1 → HCl+R2 | 1.7 × 1013 | 1 | 4 |
| 1,1+R1 → HCl+R4 | 1.2 × 1013 | 1 | 6 |
| 1,1,2+R1 → HCl+R6 | 1.7 × 1013 | 1 | 15 |
| 1,1,1,2+R1 → HCl+R7 | 1.7 × 1013 | 2 | 17 |
| CHCl3+R1 → HCl+R8 | 1.6 × 1013 | 2 | 14 |
| CCl4+R5 → Di+R8 | 5.0 × 1011 | 2 | 33 |
| CCl4+R4 → 1,1,2+R8 | 1.0 × 1012 | 2 | 33 |
| CCl4+R5 → 1,1,1,2+R8 | 5.0 × 1011 | 2 | 33 |
| Radical reaction chain termination | |||
| R2+R1 → VCM+HCl | 1.0 × 1013 | 2 | 13 |
| R3+R1 → Di+HCl | 1.0 × 1013 | 2 | 12 |
| R6+R8 → Di+CCl4 | 1.0 × 1013 | 2 | 13 |
| C2H2+2R1 → 2C+2HCl | 1.6 × 1014 | 2 | 70 |
Table 1: Energy of EDC thermal cracking reaction [2].
Mass balance equation in the software proposed in form of equation 1
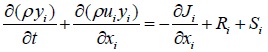 (1)
(1)
ρ=Density
t=time
ui=Potential energy
Ji=diffusion term
Si=heat at point i
Ri=input heat to the reactor
Phrase Ji reflects is the term infiltration, which is not used in this simulation [2,3]. The energy equation in the Fluent software as follows:
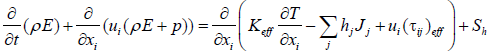 (2)
(2)
ρ=Density
p=pressure
t=time
T=Temperature
ui=input Potential energy
uj=output Potential energy
Tij=torque
Jj=Output diffusion term
si=input thermal of chemical reactions
Sh=thermal of chemical reactions
Keff=the effective conductivity
E=total energy
Kt+K=Keff is the effective conductivity, Kt turbulent thermal conductivity, according to the model used is the perturbation. The first three terms on the right side of the equation as an expression of directed energy transfer, distribution of components and dispersion of viscosity. Sh is thermal of chemical reactions [4-6]. E is expressed as follows:
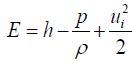 (3)
(3)
ui = input Potential energy
= pressure
ρ = Density
E = total energy
ui = input Potential energy
h = input heat
Mass continuity equation in FLUENT software is in form of eqn 4:
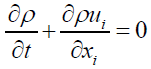 (4)
(4)
Above equation was the general form of the mass balance equation for compressible and non-compressible flows.
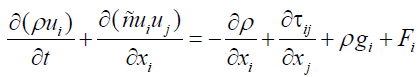 (5)
(5)
In the above equation, P static pressure, τij tensor stress, ρgi and Fi physical forces of gravity and physical external factors are in i direction. Tensor stress is as follows:
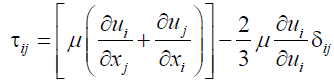 (6)
(6)
μ the molecular viscosity and the second semester is the influence of volumetric expansion [3].
Standard k-ε model
This was a comprehensive model that account as the simplest turbulence model. Two educational turbulent models enables solving the two transfer equations that separately determined turbulent length and time scale. Standard k-ε model is useful for powerful engineering calculations. Powerful of this equation, economical and acceptable accuracy for a wide range of turbulent flow as a result of its popularity in industrial flow and heat transfer simulation. This model is a semiempirical model. Standard k-ε model, was a model based on transfer equations for kinetic turbulent energy and dissipation rate (ε). In achieving the standard k-ε model assumes that the fully turbulent flow and molecular viscosity effects have been ignored. Because of the strengths and weaknesses of the equation to improve its performance, k-ε realizable and k-ε RNG model was introduced. K and ε is obtained by solving simultaneously the following two equations:
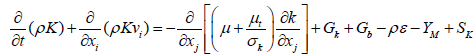 (7)
(7)
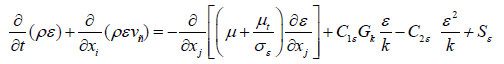
Gk=turbulent kinetic energy generated due to the gradient of speed.
Gb=turbulent kinetic energy generated due to the buoyancy forces.
YM=Share Swinging delay in the compressibility turbulence for a total loss of (j)
C1ɛ=constant parameter of model=1.44
C2ɛ=1.92
σk=turbulent Prantel numbers for k=1
σ ɛ=turbulent Prantel number for ε=1.3
SK=term defined source for k
Sɛ=term defining source for ε
μt=turbulence viscosity that can be obtained from the following equation:
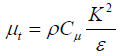 (9)
(9)
RNG k-ε model
This model is based on statistical methods, this model is the same as the standard k-ε model but with the following modifications: 1- An additional term (ε) has been added that significantly increases accuracy for flow to layer velocity. 2- The effect of the rotational movement (vortex motion) included in turbulences and increases accuracy for rotary flow. 3- An analytical formula for turbulent Prantel numbers are provided, as standard k-ε model considers a fixed amount. 4- While the standard k-ε model is the model for high Reynolds numbers, the theory of RNG for analysis of a differential equation for the turbulent viscosity can be provided that it considered the effects of low Reynolds. Effective use of this feature depends on proper behavior of the region near the wall of furnace. K-ε realizable model.
This model have limitations on Reynolds stresses that match with the physics of turbulent flows. This is possibly not in the standard k-ε model and not in the k-ε RNG model. In places where a large curvature in flow lines, vortex and rotation of the screw. K-ε RNG and k-ε realizable models have significant progress towards standard k-ε model. Preliminary studies show that the k-ε realizable model between k-ε models have best performance for confirmation numbers of complex flows and segregated flows. For all of this cases the performance of this model is better than the standard k-ε model. As it was observed that in the standard k-ε model, turbulent viscosity achieved the following equation:
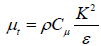 (10)
(10)
That Cμ is constant, but in the k-ε realizable Cμ achieved by the following equation:
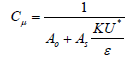 (11)
(11)
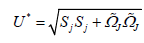 (12)
(12)
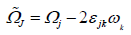 (13)
(13)
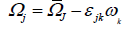 (14)
(14)
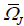 Average rate of tensor with rotational speed is ωk constants are defined below.
Average rate of tensor with rotational speed is ωk constants are defined below.
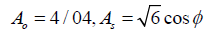
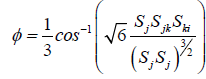 (15)
(15)
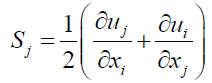 (16)
(16)
As it’s be seen Cμ placed a function of the average strain, rotational speeds, the angle speed of rotation systems, and turbulent fields are (k and ε) (Table 2).
| Temp (K) | Transfer Coefficient (W/m.K) |
|---|---|
| 373 | 15.9 |
| 573 | 19 |
| 753 | 21.2 |
| 973 | 25.7 |
| Density (Kg/m3) | 8470 |
| Heat capacity (J/Kg.k) | 444 |
Table 2: Thermo-physical properties of coils [4].
Wall of furnace
Profile of heat was applied in the walls. A total of eleven wall was located and each row of coil has a heat profile of its own. To enter the thermal flux resulting from furnaces for applying to the coils level use from proposed profiles were provided by Kegard in 2007. The length of each row of coils can be categorized Profile provided by the amount of heat to be entered in the software (Figure 4).
Boundary conditions of cracking reactor
Inlet flow: Input for fluid flow due to the mass flow rate and the percentage of each of the compounds at the entrance of the inlet is used for the mass flow condition that account as boundary condition (Table 3).
| Feed mass flow rate | Kg/s 6.086 |
|---|---|
| Mass percentage of Input EDC | 0.9707 |
| Mass percentage of Input VCM | 0.0184 |
| Mass percentage of HCL input | 0.0109 |
Table 3: Output fluid conditions.
Output flow: The output flow used for pressure boundary condition. The boundary conditions are summarized in Table 4. Investigate the conversion factor and the amount of formed coke due to the different COTs. In this part of the research in different COTs EDC conversion factor and the amount of formed coke has been investigated. The amount of COT according to recent issues in review of EDC cracking processes to the values of 760, 770, 780 and 790 are being selected. How to achieve this profile to be the first calculated 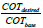 and then by using the proportion a variable factor is described. As a result, the new temperature profile in a particular COT due to the new data was achieved. In this method, input temperature to the coil doesn’t change and base COT temperature multiply in the
and then by using the proportion a variable factor is described. As a result, the new temperature profile in a particular COT due to the new data was achieved. In this method, input temperature to the coil doesn’t change and base COT temperature multiply in the 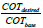 and the other base profile points due to eq.17 and eq.18 multiply in a constant parameter to obtain new Profile points.
and the other base profile points due to eq.17 and eq.18 multiply in a constant parameter to obtain new Profile points.
| Location | Type of boundary condition |
|---|---|
| Walls | Heat flux |
| Input gas | Input mass rate |
| Output flow rate | Output pressure |
Table 4: Applied boundary conditions in the software.
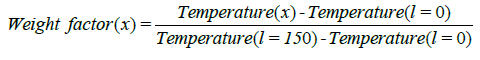 (17)
(17)
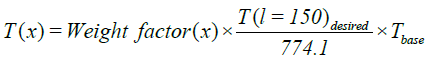 (18)
(18)
The results of these calculations can be seen in Figure 5, COT different temperature profiles have been obtained. Figure 6 indicates the EDC conversion factor in different COTs, as the figure shows at higher conversion factor it will be higher in the COTs. But according to the Figure 7 if the amount of COT is higher, the amount of formed coke also will be higher. So by regarding the simulation results it can be said that for optimum economic performance of furnace, the temperature profile of the fluid is very important.
Investigating of adding carbon tetrachloride as propulsion
By adding this compound to the feed in addition to increase conversion factor, it has other effects on the process. Due to increasing number of chlorine radicals in the process, number of unwanted compounds and Acetylene production and as a result coke production has been increased. Creating unwanted compounds in the next stages, in addition to VCM treating problem, also an important loss costly. As shown in Figure 8 conversion factor increases with increasing carbon tetrachloride, on the other hand in the Figure 9 formed coke, of the increases in carbon tetrachloride. So regarding to the simulation results it can be said that for optimum economic performance of furnace, the temperature profile of the fluid is very important. Therefore, optimize the amount of added carbon tetrachloride to the feed for economic performance of thermal cracking unit is very important. In this amount of propulsion conversion factor increases as an allowable limit and reactor performance due to forming coke and unwanted compounds are economical. It can be seen in the Figure 5.
K-ε realizable model is the most appropriate model for calculation because of these reasons: [7,8]
1- This version contains a new formula for turbulence viscosity.
2- A modified transferring equation for scattering rate, ε, can be considered. Therefore, this model is an exact equation for fluctuations transferring has been made.
In this research, effect of added carbon tetrachloride as a propulsion has been investigated. According to result of this research and other issues, if the amount of this compound does not reach more than 100 ppm, conversion factor will be increased. If this amount will be more than 100 ppm it’s not economically suggested.