Journal of Physical Chemistry & Biophysics
Open Access

First-Principles Study of Pyroxene Structure LiVO3

Mohamed Khedidji^{* *}Correspondence: Mohamed Khedidji, Department of Chemistry, Theoretical Chemistry and Computational Photonics Laboratory, University of Science and Technology, Houari Boumediene, BP 32 El-Alia, Algiers, 16111, Algeria, Email:

Author info »

Abbreviations

LIBs: Lithium-Ion Batteries; EV: Electric Vehicles; HEV: Hybrid Electric Vehicles; DFT: Density Functional Theory; GGA: Generalized Gradient Approximation; ONCV: Optimized Norm- Conserving Vanderbilt pseudopotentials; DFPT: Density-Functional Perturbation Theory

Introduction

The switchable macroscopic polarization characteristic of ABO₃ type ferroelectric materials makes them good candidates for modern devices such as pyroelectric detectors, binary memories and electro-optic modulators for communication systems [1- 4]. The Lithium Vanadium (LiVO₃) is one of these materials, widely used in the manufacturing of modern devices. It has been qualified to be used as solid electrolyte material as well as composite cathode for rechargeable Lithium-Ion Batteries (LIBs) used for Electric Vehicles (EV) and Hybrid Electric Vehicles (HEV) [5,6].

Due to the high mobility of Li⁺ cation, the unidimensionnal character of its structure and its electrochemical characteristics, LiVO₃ appears as an important ferroelectric material which has been studied intensively in the past years [7-11]. LiVO₃ belongs to the class of metavanadate pyroxenes family of the type MVO₃ (M=Li, Na, K, Rb and Cs), in which infinite chains of corner shared VO₄ tetrahedra, interconnected through MO₆ octahedra. The experimental studies showed that LiVO₃ must undergoes a ferroelectric-paraelectric phase transition at about 410°C from the Cc polar phase to the C2/c centrosymmetric phase [12,13].

By mean of high-resolution Neutron Powder Diffraction method, Muller, et al. [13], have associated this kind of phase transition to the reorientation of the anisotropic displacement ellipsoids of the lithium atoms around their average sites. This slight modification in structure introduced a symmetry problem in several experimental studies. Therefore, a detailed knowledge of ferroelectricity and phase transition mechanisms in LiVO₃ is still remains obscure.

In the present work, we investigate the structural, dielectric and dynamical properties of monoclinic LiVO₃, using first-principles methods. We study and discuss the possible occurrence of the C2/c-Cc ferroelectric phase transition. The condensation of the obtained phonon instabilities reveal that the P2_1/c phase, obtained from the condensation of the unstable Y₂⁺ mode into the paraelectric C2/c phase, is the ground state phase. In addition, the condensation of the stable polar mode Γ₂⁻ into the paraelectric C2/c phase leads to the ferroelectric phase of Cc symmetry. This means that the ferroelectric phase transition remains possible for monoclinic LiVO₃. Finely, the spontaneous polarization of such a ferroelectric phase is then calculated using two methods: The Berry phase formalism and from the knowledge of the born effective charge and the dielectric tensor.

Materials and Methods

Computational details

Our calculations were performed in the general framework of the Density Functional Theory (DFT) [14,15]. Using planewave pseudopotentials method as implemented in the ABINIT software package [16,17]. We chose the Generalized Gradient Approximation (GGA) [18], as exchange-correlation functional. Optimized Norm-Conserving Vanderbilt pseudopotentials (ONCV) were used to represent the core region of lithium, vanadium and oxygen atoms [19]. The V (3s 3p 3d), Li (2s) and O (2s 2p) levels, were considered as valence states in the generation of the pseudopotentials. Wave functions were extended in plane waves up to a kinetic energy cutoff of 45 Ha. The Brillouin zone was sampled with a 4 × 5 × 6 Monkhorst- Pack k-point grid converges the results very well [20]. We relaxed the structure until the remaining forces on the atoms are less than 10-5 Hartree/Bohr and the stresses on the unit cell are smaller than 10-7. Phonons frequencies, Born effective charges and dielectric tensors were computed using Density-Functional Perturbation Theory (DFPT) [21,22].

Results and Discussion

Structure

We first determined the structural parameters of the experimentally observed High-temperature paraelectric C2/c phase of LiVO₃ by relaxing simultaneously the unit cell shape and the atomic positions. The C2/c structure has 40 atoms per conventional unit cell-or 20 atoms in the primitive unit cell. The crystal structure, as shown on Figure 1, exhibits the altering chains of VO₄ tetrahedra (gray) and distorted LiO₆ octahedra (blue). This structure consists of bands of V-O corner-shared tetrahedra run parallel to c-axis and is interconnected through edge-shared LiO₆ octahedra which also run parallel to this axis. Our results after full structural optimization are listed on Table 1. Except of the typical error of the GGA method that is known to overestimate the lattice parameters, the results are in good agreement with the experiment.

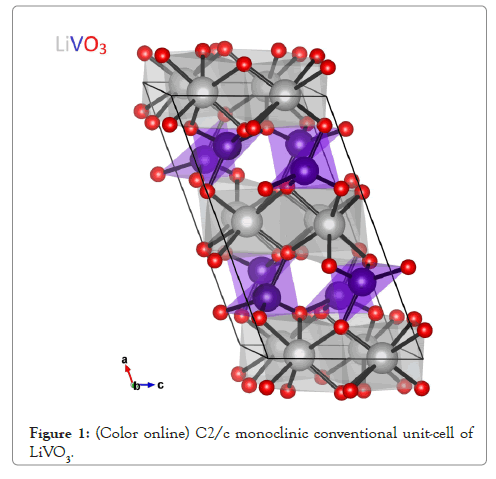
Figure 1: (Color online) C2/c monoclinic conventional unit-cell of LiVO₃.

Table 1: Calculated lattice parameters and atomic positions of LiVO₃ in the C2/c monoclinic phase. In parentheses are the experimental values reported here from [13].

Dielectric properties

The born effective charge (Z*) and the optical dielectric tensor (ε_∞) are important and central for the parameterization of the LO-TO splitting that is considered as the contribution of the long range coulomb interactions to the lattice dynamics. These two quantities have been computed using linear-response techniques [22]. The elements of the calculated ε_∞tensor are reported in Cartesian coordinates with the x and y axes aligned along the a and b directions of C2/c structure, respectively, and the z axis makes an angle of (β-π/2) with the c direction. Within this choice of Cartesian axes, the ε_∞tensor is blocking diagonal in y and xz subspaces;

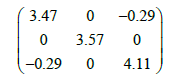
Neither experimental nor theoretical values to compare our results. It is well known that, for the dielectric tensor, the calculated values always overestimate the experimental one.

Table 2 shows the calculated Z* tensors for non-equivalents atoms. The Z* tensors are reported in Cartesian coordinates using the same set of axes as used for the dielectric tensor. The C2/c symmetry of LiVO₃ requires that the Z* tensor should be anisotropic on each atom, so that the charge transfer takes place in different directions. Moreover, all the calculated Z* values are close to their corresponding nominal charges, which, contrast with what is observed for the class of ferroelectric perovskites that exhibit anomalous Z* [23].

Table 2: Born effective charges calculated for monoclinic LiVO₃. The nominal charges of Li, V and O are +1, +5 and -2, respectively.

Dynamical properties

To study the structural instabilities in the monoclinic C2/c configuration of LiVO₃, we performed zone-center phonon calculations in the conventional cell of 40 atoms (Figure 1). In this configuration the Brillouin zone is folding, and so gives access to phonons at Γ (0, 0, 0) and Y=(0, 1, 0) points. We obtained three unstable modes. The strongest unstable mode is at 61i cm^-1 and corresponding to the irreductible representation . Γ₂⁺ The two remaining unstable modes are at 57i cm^-1 and 24i cm^-1 and corresponding to the irreductible representation Y₂⁺ and Y₂⁻ , respectively (Table 3).

Table 3: Calculated eigendisplacements (in a.u.) of unstable TO modes. Atomic displacements corresponding to polar mode Γ₂^- are also reported. The values in brackets present the frequencies of different modes in cm^-1.

In order to study the symmetry-breaking behavior of C2/c monoclinic LiVO₃, we first individually condensed the three unstable modes into the C2/c parent phase and then we fully optimized the aroused distorted phases. We ported on Table 4 the low-symmetry phases with their corresponding cell parameters obtained after full relaxation and their energy differences (ΔE_m) calculated with respect to the C2/c parent structure. The condensation of mode Γ₂⁺ leads to a triclinic structure of space group. While, the condensation of the nonpolar modes Y₂⁺ and Y₂⁻ lead to a monoclinic structure of space group P2_1/c. Also, the calculated ΔE_m show that the P2_1/c phase arising from the condensation of Y₂⁺ mode is the most stable phase because it exhibits the lowest ΔE_m, with an important energy gain of about 150 meV/f.u. Significantly, as shown on Table 4, the condensation of the stable polar mode Γ₂⁻ into the C2/c paraelectric phase leads to a ferroelectric phase of Cc symmetry. Despite numerous experimental efforts, this phase transition is still ambiguously stated. This is probably due to the very small structural changes between Cc and C2/c phases [13].

Table 4: Cell parameters (lengths in Å and angles in C°), energy differences ΔE_m (in meV/f.u) and space groups of different phases obtained after condensation of unstable modes.

Spontaneous polarization

According to the modern theory of polarization [24], the spontaneous polarization of bulk LiVO₃ is well attributed to a formal transformation from a paraelectric C2/c structure to a polar Cc structure. The Cc symmetry of LiVO₃ allows a development of a spontaneous polarization along the x and z axes. To calculate this fundamental quantity we used both the Berry Phase approach (BP) [24], and from the knowledge of the born effective charges and atomic displacements as;

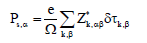
where Z*_κ,αβ is the born effective charge tensor of atom k and δ_τκ,β presents the displacement of such atom along the direction β during the phase transition from the paraelectric C2/c phase to the ferroelectric Cc phase. This estimation gives 3.19 μC/cm² along the x direction and 7.28 μC/cm² along the z direction. From BF formalism, the computed spontaneous polarization is of 5.80 μC/cm² and 7.56 μC/cm² along the x and z directions, respectively. Finely, we observe that the P_s(Z*) underestimates P_s(BP) along the a direction, while along the c direction P_s(Z*) is in closer agreement with P_s(BF).

Conclusion

We have performed a systematical study of structural, dielectric and dynamical properties of LiVO₃ in its high-temperature paraelectric structure of C2/c symmetry using density functional theory and density functional perturbation theory. We characterized the C2/c high-temperature structure. Dielectric properties and phonon frequencies were calculated. Unlike typical perovskites, the computed Z* are not anomalous and are close to their nominal values. A phonons calculation reveals three structural instabilities that lead after their condensation to more stable phases of monoclinic and triclinic symmetries. The ground state phase is monoclinic of P2_1/c symmetry. Finely, the condensation of the polar mode at 14 cm^-1 leads to the polar phase of Cc symmetry, which confirms the possible occurrence of the ferroelectric-paraelectric phase transition.

References

1. Lines ME, Glass AM. Principles and applications of ferroelectrics and related materials. Oxford Classic Texts in the Physical Sciences, Clarendon Press, Oxford. 1977.

   [Crossref] [Google Scholar]

2. Nishihara, Haruna M, Suhara T. Optical integrated circuits, edited by RE Fischer and WJ Smith, optical and electro-optical engineering series. Mcgraw-Hill, New York. 1985.
3. Wooten EL, Kissa KM, Yi-Yan A, Murphy EJ, Lafaw DA, Hallemeier PF, et al. A review of lithium niobate modulators for fiber-optic communications systems. IEEE J Sel Top Quantum Electron. 2000;6(1):69-82.

   [Crossref] [Google Scholar]

4. Buse K, Adibi A, Psalti D. Non-volatile holographic storage in doubly doped lithium niobate crystals. Nature. 1998;393(6686)665-668.

   [Crossref] [Google Scholar]

5. Huang Z, Cao L, Kuang Y, Zhou H, Fu C, Chen Z. Preparation, characterization, and lithium intercalation behavior of LiVO3 cathode material for lithium-ion batteries.  J Phys Chem C. 2016;120(6):3242-3429.

   [Crossref] [Google Scholar]

6. Lee JB, Moon J, Chae OB, Lee JG, Ryu JH, Cho M, et al. Unusual conversion-type lithium in LiVO3 electrode for lithium-ion batteries. Chem Mater. 2016;28(15):5314-5320.

   [Crossref] [Google Scholar]

7. Jian XM, Tu JP, Qiao YQ, Lu Y, Wang XL, Gu CD. Synthesis and electrochemical performance of LiVO3 cathode materials for lithium ion batteries. J Power Sources. 2013;236:33-38.

   [Crossref] [Google Scholar]

8. Jian JB , Wenren HQ, Huang S, Shi SJ, Wang XL, Gu CD, et al. Oxalic acid-assisted combustion synthesized LiVO3 cathode material for lithium ion batteries. J Power Sources. 2014;246:417-422.

   [Crossref] [Google Scholar] [PubMed]

9. Li P, Ren X, Guo GC, He L. The origin of hyperferroelectricity in LiBO3 (B= V, Nb, Ta, Os). Sci Rep. 2016;6:34085.

   [Crossref] [Google Scholar] [PubMed]

10. Li S, Zhang Y, Tang Y, Tan X, Liang S, Zhou J. Facile synthesis of LiVO3 and its electrochemical behavior in rechargeable lithium batteries.  J Electroanal Chem. 2019;853:113505.

    [Crossref] [Google Scholar]

11. Khedidji M, Amoroso D, Djani H. Microscopic mechanisms behind hyperferroelectricity. Phys Rev B. 2021;103(1):014116.

    [Crossref] [Google Scholar]

12. Kashid AP, Patil VV, Chavan SH. Pyroelectric properties of Gd-doped KVO3 and LiVO3. Bull Mater Sci. 1989;12(1):57-62.

    [Crossref] [Google Scholar]

13. Muller C, Valmalette JC, Soubeyroux JL, Bouree F, Gavarri JR. Structural disorder and ionic conductivity in LiVO3: A neutron powder diffraction study from 340 to 890 K. J Solid State Chem. 2001;156(2):379-389.

    [Crossref] [Google Scholar]

14. Hohenberg P, Kohn W. Inhomogeneous electric gas. Phys Rev B. 1964;136(3B):B864.

    [Crossref] [Google Scholar]

15. Kohn W, Sham LJ. Self-consistent equations including exchange and correlation effects. Phys Rev B. 1965;140(4A):A1133.

    [Crossref] [Google Scholar]

16. Gonze X, Amadon B, Anglade PM, Beuken JM, Bottin F, Boulanger P, et al. ABINIT: First-principles approach to material and nanosystem properties. Comput Phys Commun. 2009;180(12):2582-2615.

    [Crossref] [Google Scholar]

17. Gonze X, Beuken JM, Caracas R, Detraux F, Fuchs M, Rignanese GM, et al. First-principles computation of material properties: The ABINIT software project. Comput Mater Sci. 2002;25(3):478-492.

    [Crossref] [Google Scholar]

18. Perdew JP, Burke K, Ernzerhof M. Generalized gradient approximation made simple. Phys Rev Lett. 1996;77:3865-3868.

    [Crossref] [Google Scholar] [PubMed]

19. Hamann DR. Optimized norm-conserving vanderbilt pseudopotentials. Phys Rev B. 2013;88(8):085117.

    [Crossref] [Google Scholar]

20. Monkhorst HJ, Pack JD. Special points for brillouin-zone integrations. Phys Rev B. 1976;13(12):5188.

    [Crossref] [Google Scholar]

21. Baroni S, De Gironcoli S, Dal Corso A, Giannozzi P. Phonons and related crystal properties from density-functional perturbation theory. Rev Mod Phys. 2001;73:515.

    [Crossref] [Google Scholar]

22. Gonze X, Lee C. Dynamical matrices, born effective charges, dielectric permittivity tensor, and interatomic force constants from density-functional perturbation theory. Phys Rev B. 1997;55(16):10355.

    [Crossref] [Google Scholar]

23. Ghosez P, Michenaud JP, Gonze X. Dynamical atomic charges: The case of ABO3 compounds. Phys Rev B. 1998;58(10):6224.

    [Crossref] [Google Scholar]

24. Resta R. Macroscopic polarization in crystalline dielectrics: The geometric phase approach. Rev Mod Phys. 1994;66(3):899.

    [Crossref] [Google Scholar]