The aim of this paper is to discuss the meaning of a generalization of extensive structures [1,2] based on Matsushita’s [3,4] approach in the context of measurement theory. Extensive structures can be useful in measuring attributes that possess an invariant unit. However, extensive structures may be not adequate in intertemporal choice because it is often observed that the value of money (a unit) decreases with the passage of time. This observation is caused by a behavioral tendency for many people to prefer to advance the timing of satisfaction, called impatience [5]. To reflect this behavioral tendency, extensive structures were generalized such that the concatenation operation can be nonassociative and non-commutative [3]. Consequently, a weighted additive function (having a constant weight) was obtained, and it is suitable for intertemporal choice problems as long as the assumption that discount is constant (i.e., constant impatience) is acceptable. Unfortunately, the assumption is often violated in actual intertemporal choice problems [6]. Recently, it was reported that we could deal with the problem of non-constant impatience by introducing the concept of subjective time perception [7,8]. Therefore, right action by “subjective” durations should be devised on the generalized extensive structure. Herein, right multiplication (or right division) by subjective durations denotes the advanced (or postponed) receipt of outcomes [4]. By the introduction of right action, the weighted additive function was transformed into an extended form such that the weight is a function of time, which can reflect non-constant impatience.

An extensive structure is characterized by a set of properties underlying the concept that an object’s attribute can be measured by counting the number of unit copies included in the said attribute [1,2]. Precisely, the extensive structure is specified as a weakly ordered set that is equipped with a monotone concatenation operation for which the associativity, restricted solvability, positivity and Archimedean conditions are satisfied. Attention should be focused on ensuring that the above conditions imply the commutativity of the concatenation operation (see ref [1,2] for the details of each condition). The definitions of commutativity, associativity, and the Archimedean condition are given in the following example because these conditions are the subject of this paper.

We should bear in mind that extensive structures would be beneficial to measuring attributes that possess a unit invariant with respect to time and space, which can be chosen arbitrarily. This can be illustrated by length measurement. Consider a set of rods. Assume that the set is equipped with two relations, one of which is an ordering relation 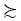to compare the length of rods and the other of which is an operation ° that concatenates rods by laying them end to end in a straight line. Let a and b be arbitrary two rods. If a is longer than or equal to b, then we write a 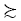b. In particular, the case where a 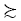b and b 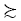a is written as a ~ b, which means that a is equivalent to b in length. For any positive integer n, the n-copy of a is defined by 1a=a and na=(n-1)a ° a. Hereafter, let A be the set of all the rods and all the finite concatenations of rods. Under this preparation, the Archimedean condition is specified as follows: for any a,b ∈ A, there exists a positive integer n such that na 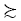b. Now take a as a unit. The Archimedean condition guarantees the existence of an integer k>0 such that:

(k+1)a > b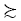 ka (where > denotes the greater than relation)

Let u be a function that assigns a real number of length to each rod and define u(a)=1. The above inequality states that u(b) lies between k and k+1. To make this measurement precise, a similar procedure may be applied to the m-copy of b. Again from the Archimedean condition, we obtain:

(l+1) a > mb 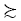la for some l > 0.

If u is to be determined such that u(mb)=mu(b), then u(b) falls in the interval between l/m and (l+1)/m. By making m greater and greater and continuing this procedure, u(b) will approach the true value. In this procedure, it is clear that the concatenation operation ° is associative and commutative up to equivalence, i.e., a ° b ~ b ° a and (a ° b) ° c ~ a ° (b ° c) for all a, b, c ∈ A. It is clear that the Archimedean condition plays an important role in assigning a proper real number to u(b).

The system (A, 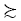, °) in the example is an extensive structure. This example suggests that for any extensive structure, there exists a function u having the following properties:

Order preservation: a b 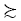⇔ u(a) ≥ u(b),

Additivity: u(a ° b)=u(a)+u(b).

The former and latter properties show that the function u sends the ordering and concatenation relations among rods into the respective relations among real numbers. This order-preserving and additive function u is called an additive representation of an extensive structure. Note that u is a ratio scale; that is, u′is the other additive representation of (A, 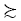, °) if and only if u′ =au for some real number α>0.

In general, in intertemporal choice problems, a preference for advancing the timing of satisfaction (impatience [5]) has been observed. For instance, receiving $10,000 this year and $5000 next year will probably be preferred to receiving $5000 this year and $10,000 next year. This preference suggests that a unit of the attribute (in this case, the value of money) changes with the passage of time. Hence, it will be improper to directly explain this kind of preference in the frame of extensive structures.

To address the problem, Matsushita [3] generalized extensive structures so that the concatenation operation need not always be commutative or associative, although the Archimedean condition is still satisfied (for accomplishing measurement in the real numbers). We examine the meaning of non-commutativity and non-associativity from the viewpoint of expressions of temporal sequences. Henceforth, the order relation 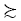should be interpreted as a preference relation. Non-commutativity of the concatenation operation arises to explain the above-mentioned preference. Let a and b be two outcomes. Let ab denote the concatenation of a and b, which means their two-period outcome sequence. Set a=$10,000 and b=$5000. Then the abovementioned preference is expressed by ab > ba . If a unit was invariant between the first and second periods, ab would be indifferent to ba, i.e., ab ~ ba. Non-associativity clarifies the difference between the left-branching fashion (i.e., concatenation on the right) and the rightbranching fashion (i.e., concatenation on the left) in expressions of temporal sequences. The following two rules are laid down. First, we express a temporal sequence based on the left-branching fashion. Second, we count the receipt period number of each component in a temporal sequence going back to the past, after setting the receipt period of the last component at the latest period. Hence, we always write (…((a₁a₂)a₃)…a_n-1)an to denote the outcome of receiving a₁ in period 1, a₂ in period 2, …, an in period n. On the other hand, the expression based on the right-branching fashion has an entirely different meaning: a₁(a₂…(a_n-2(a_n-1a_n))…) denotes an outcome of receiving a₁, a₂, …, a_n-1 in period n-1 and an in period n. A brief case helps us understand the reason. By the first rule, a(bc) (a,b,c ∈ A) is interpreted as the twoperiod temporal sequence of a single outcome a and a compound outcome bc. Clearly, a is received in period 1 and by the second rule, the last component c of bc is received in period 2, so that b must be received in period 1, as required. The right-branch fashion has an advantage in that we can express a simultaneous receipt of outcomes in the same period (i.e., the period just before the latest period). This notation can give a special meaning to the following condition:

a(bc) ~ b(ac). (1)

Indeed, this condition implies that given the same outcome c in the latest period, the preference is invariant regarding the order of receiving a and b in the preceding period. Hence, Condition (1) will suggest that a concatenation that is defined to mean a simultaneous receipt of outcomes should be commutative.

The extensive structure generalized such that the concatenation operation is non-commutative and non-associative can be easily transformed into an extensive structure. Let A be the set of all temporal sequences of outcomes. A null outcome e, which is a left identity element (i.e., ea ~ a for all a ϵ A), plays an important role in this transformation. The right multiplication of a by e, denoted ae, implies advancing the receipt of a by one period; the right division of a by e, denoted a/e, implies postponing the receipt of a by one period. These two operations are the inverse operations of each other:

(ae)/e ~ (a/e)e ~ a. (2)

By definition, (a/e)b can be interpreted as receiving both a and b in the same period. If an operation is defined by

a ° b=(a/e)b, (3)

then it would be rational to consider the operation ° as commutative and associative up to equivalence. Mathematically, the commutativity and associativity of ° is deduced from Condition (1). Further, it can be shown that the generalized extensive structure becomes an extensive structure with respect to °, in which e is an identity element (i.e., e ° a ~ a ° e ~ a). Hence, there exists an additive representation u with respect to °. Since ab ~ ((ae)/e)b=ae ° b by (2), (3) and monotonicity of the concatenation operation, it follows from the order-preserving and additivity properties of u that,

u(ab)=u(ae)+u(b)

Assume here that right multiplication by e (which means the advancement operator) satisfies the following conditions:

(ab)e ~ (ae)(be), (4)

a 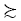b ⇔ ae 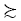be, (5)

ae 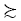a. (6)

Condition (4) provides consistency in the meaning of right multiplication by e, because it will be rational to consider advancing the receipt of the temporal sequence ab by one period equivalent to the temporal sequence (ae) (be) in which each receipt of a and b is advanced by one period. Condition (5) states that the preference of outcomes is invariant under their advancement by one period. Condition (6) states that any advanced outcome by one period is never less preferred than the original outcome, which means impatience in a wider sense. Omitting the mathematical proof, it is seen from (4) and (5) that the set of temporal sequences of type ae (a ∈ A) is also an extensive structure with respect to ° and °, in which e is an identity element. In view of u being a ratio scale and (6), it follows that u(ae)=αu(a) for some real number α ≥ 1. Substituting this equality into the additive representation displayed at the end of the previous paragraph, we obtain a weighted additive representation:

u(ab)=u(ae)+u(b) where α ≥ 1. (7)

Recall that u is a ratio scale.

It is notable that by (7) we can evaluate preferences between temporal sequences having any different numbers of periods because (7) is a representation with respect to the concatenation operation (not a representation of a product set).

Example 1: Assume that the receiving period of the last component in each of the two temporal sequences for comparison is defined as period 3.

(i) The comparison between sequences of outcomes of the last three and last two periods is given as:

(a₁a₂)a₃ vs. b₂b₃.

The weighted additive representation of (7) assesses a preference as follows:

(a₁a₂)a₃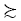 b₂b₃⇔ α₂u(a₁)+αu(a₂)+u(a₃) ≥ αu(b₂)+u(b₃)

(ii) The comparison between sequences of outcomes of the future three and future two periods is given as:

(a₁a₂)a₃ vs. (b₂b₃)e.

Similarly, the representation of (7) assesses a preference as follows:

(a₁a₂)a₃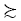(b₂b₃)e ⇔ α2u(a₁)+αu(a₂)+u(a₃) ≥ α₂u(b₂)+αu(b₃)

This notation makes it possible to express the situation where the receipt of outcomes is advanced or postponed by multiple periods. Indeed, the repeated use of right multiplication or right division by e yields an n-period advancement or n-period postponement, respectively:

Advancing case: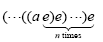

Postponing case: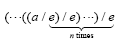

Moreover, by (2) and (5), we have:

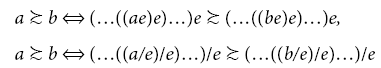
as long as the number of e in each side to multiply or divide is the same. These relations say that a preference between outcomes is invariant under advancement and postponement by the same number of periods. This property is called stationarity [5]. Applying the weighted additive representation of (7) to the n-period advancement and postponement, we obtain:

Advancing case: u((…(ae)…)e)=αⁿu(a), (8)

Postponing case: u((…(a/e)…)/e)=(1/α)ⁿu(a). (9)

Here α and 1⁄α are interpreted as a markup and a discount rate, respectively.

It is well known that the state of stationarity (i.e., constant impatience) is often violated [6]. For instance, assume that a person is faced with the following choice problems.

A: receiving $1000 now vs. B: receiving $1100 after one year.

A′: receiving $1000 after two years vs. B’: receiving $1100 after three years.

Option A would probably be preferred to Option B. Conversely, Option A′ may be less preferred than Option B′ although the time lag of a pair of options is the same as the previous choice. This is because impatience decreases with the passage of time (decreasing impatience). The representation of (7) cannot explain these preferences because it has a constant discount rate, 1/a. Indeed, from Eq. (9) it is seen that if u($1000)>(1/α)u($1100), then (1/α²)u($1000)>(1/α³)u($1100). An exponential discount function, exp(-rT) where T is an objective time duration of delay and r is a discount rate, also cannot explain the preferences from the same reason (i.e., having a constant discount rate), but a generalized hyperbolic discount function [6], 1/(1+bT)ra with arbitrary constants a, b can.

Takahashi et al. [7] provided interesting knowledge about this problem. That is, the exponential discount functions with logarithmic time perception, a subjective time duration S of delay with the logarithmic unit, is transformed into the generalized hyperbolic discount function with the objective time duration T:

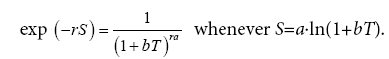
In other words, perceiving time according to a logarithmic scale and constantly discounting in terms of this perceived time yields decreasing impatience, because the exponential discount function reflects constant impatience and the hyperbolic one captures decreasing impatience [8].

This knowledge gives us the following idea. If a one-period postponement occurs in a period closer to the present, then a person is sensitive to the postponement, and if the person is more impatient, then he/she may feel as if the time increment is longer than the actual increment. To allow for the effect of the time duration varying according to a period in which a postponement occurs, a one-period postponement is expressed by dividing outcomes by an increment in a “subjective” duration (not e) corresponding to the period on the right. To be more precise, let si be an increment in a subjective duration when advancing (or postponing) the receipt of a, from period i-1 to period i in the previous (or future) direction. Then, the n-period advancement and postponement can be rewritten by right multiplication and right division, respectively:

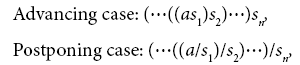
Figure 1 illustrates that the advanced or postponed receipt of a that is defined by right multiplication or right division by subjective durations when setting the present at a time point of reference. Right multiplication and right division are also the inverse operations of each other:

Figure 1: Advanced or postponed receipt of an outcome that is defined by right multiplication or right division by increments of a subjective duration when setting the present at a time point of reference.

(as)/s ~ (a/s)s ~ a. (10)

Here as (or a/s) denotes the one-period advancement (or postponement) of receiving a at an arbitrary period, which is possible by setting the arbitrary period at a time point of reference.

To introduce right action based on right multiplication by subjective durations in the generalized extensive structure, right multiplication is assumed to inherit the conditions corresponding to (4), (5) and (6) [4]:

(ab)s ~ (as)(bs), (11)

Similarly, from (10) and (12) we obtain:

These relations are a “generalization of stationarity” in the sense that the concept of subjective time durations is introduced.

It is notable that the delayed preferences can be divided into three cases by using the right division (the advanced preferences can be also divided similarly by using the right multiplication).

Case 1. 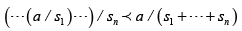. This case implies that the n-step delay reduces the value of a more than the one-step delay, although the sum of delay durations is the same.

Case 2. 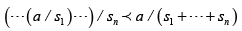. This case implies that the n-step delay does not reduce the value of a more than the one-step delay, although the sum of delay durations is the same.

Case 3. 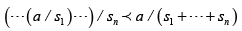. This case implies that the step-by-step delay has no effect.

As a representation of the generalized extensive structure equipped with right action, we obtain an order-preserving function having the following properties:

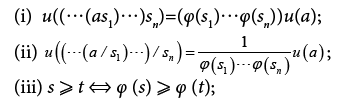
where, u is the weighted additive representation of (7), and φ is a weight function, which is an absolute scale. The properties (i) and (ii) are generalizations of (8) and (9), respectively. Hence, the representation can reflect non-constant impatience because it has a markup (or discount) rate varying according to time, and it is called a generalized weighted additive representation.

It is worthwhile to show the relation between this representation and the typical discount functions (the exponential and the generalized hyperbolic discount function). We will now restrict our concern to the third case (i.e., the step-by-step delay having no effect). Let 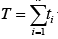with an increment ti in the objective duration. In the third case, it can be seen [4] that the reciprocal of φ becomes the exponential function:

1/φ(T)=exp(-rT) for some r>0.

Therefore, as was mentioned in the second paragraph of this section,

if the sum of subjective time durations is calculated in a logarithmic scale [7], then the reciprocal of φ becomes the generalized hyperbolic discount function:

Example 2. The generalized weighted additive representation can explain the preferences of the example shown in the top of this section. Assume that a cumulative subjective duration until year k is calculated by S_k=a.ln(1+bk). Then, by the property (ii) and Eq. (14),

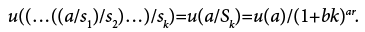
For example, let u($1000)=1.0 and u($1100)=1.1, and set a=3.46, b=0.5, r=0.1. Then u($1000)>u($1100/s₁) and u(($1000/s₁)/s₂)1)/ s₂)/s₃), implying the above-mentioned preferences.

Before closing the section, we shall discuss the possibility of applying the theory of the generalized extensive structure with right action to problems in the field of psychology and psychotherapy. The relationship [9-12] between psychiatric problems (e.g. depression, addiction, obesity and suicide) and temporal discounting has already been investigated. More precisely, by using a particular discount function (which can be transformed to the generalized hyperbolic discount function), it was reported by Takahashi et al. [9] that depressive patients were more impatient and inconsistent in temporal choice (which means timedependency of impatience) than healthy control subjects. Additionally, Takahashi [10-12] emphasized the significance of observing the variation of two parameters in the discount function regarding both impatience and inconsistency for patients with the above-mentioned psychiatric diseases, because neurobiological substrates (e.g. serotonin, dopamine and steroid hormones) are not only related to the above diseases but also somewhat correlated with temporal discounting and time perception. To actually begin such study, it is presupposed that we examine what type of discount function explains temporal discounting of each subject most suitably, and in general, for this purpose, curve fitting for each discount function will be conducted by using some optimization algorithm. It is to be noted that our theory might provide an axiomatic measurement approach (not a curve fitting approach) in selecting the most appropriate discount function. To state this concretely, assume that all conditions for the generalized weighted additive function are satisfied. Moreover, assume that the equivalence of Case 3 also holds, herein this assumption seems to be valid for a narrow interval of time horizon. Our theory asserts that an appropriate discount function becomes the exponential or the generalized hyperbolic type according as the usual or a logarithmic scale is adopted to accumulate subjective time durations. However, a further study is needed to develop an axiomatic measurement determination method for selecting an appropriate discount function. The development may be achieved by deriving the condition for an additive representation of closed extensive structures [1] to be a logarithmic form (for example, refer to [13]) because the usual (arithmetic) addition has already been extended [4] to a binary operation on the structures that concatenates subjective durations. This is an urgent future research. Another future work is to develop an axiom system to construct a discount function for loss in the framework of a “negativity” version of the generalized extensive structure in which positivity is replaced with negativity, because it has been reported [9,10,12] that the difference in temporal discounting between gain and loss could be useful in dealing with psychiatric problems.

This paper, focusing on a problem involved in extensive structures, has considered the solutions in the context of intertemporal choice. Extensive structures can certainly be useful in measuring attributes when there is a unit invariant with respect to time, whereas they will not be adequate when a unit varies according to time. To solve the problem, extensive structures have been generalized such that the concatenation operation can be non-commutative and non-associative. Herein, non-commutativity is needed to express different preferences for two-period temporal sequences arising from changing the order of receipt of each outcome; non-associativity plays an important role in transforming the generalized extensive structure into an extensive structure with respect to a newly defined operation. A weighted additive function (the representation of the generalized extensive structure with non-associative and non-commutative operation) can explain the preferences when the degree of consumers’ impatience is constant regardless of receipt time, but it cannot when constant impatience is violated. To address the problem, with the help of the concept of subjective time perception, right action based on right multiplication by subjective durations was introduced into the generalized extensive structure. Finally, a generalized weighted additive function was obtained as a representation of the generalized extensive structure equipped with right action. The generalized extensive structure equipped with right action seems to be useful for intertemporal choice also regarding health consequences because it has an advantage that the set of outcomes does not always need to be a set of real numbers. A topic for future research is to derive a condition for the additive representation of closed extensive structures to be a logarithmic form. This is an essential work when selecting an appropriate discount function by collating subjects’ behaviors with the condition.

This work was supported by JSPS KAKENHI Grant Numbers 25330330 and 17K00392.