International Journal of Advancements in Technology
Open Access
ISSN: 0976-4860
ISSN: 0976-4860
Research Article - (2016) Volume 7, Issue 2
We analyze the spectrum of entanglement fluctuations and local entanglement that holds true for the two-mode photon system. We also present a definition for the degree of local entanglement. In order to carry out our analysis, we consider a quantum system with a Gaussian variable with zero mean. It is found that 50% maximum degree of entanglement as well as 75% maximum degree of squeezing occurs at steadystate and threshold in the given frequency interval.
<Keywords: Entanglement; Fluctuation; Local; Spectrum
In recent years, the topic of continuous-variable entanglement has received a significant amount of attention as it plays an important role in all branches of quantum information processing [1]. The efficiency of quantum information schemes highly depends on the degree of entanglement. A non-degenerate parametric amplifier at and above threshold has been theoretically predicted to be a source of light in an entangled state [2,3]. Recently, the experimental realization of the entanglement in non-degenerate parametric amplifier has been demonstrated by Zhang et al. [4]. In a non-degenerate parametric amplifier, a pump photon of frequency ωc is down converted into highly correlated signal and idler photons with frequencies ωa and ωb such that ωc= ωa + ωb [5]. A detailed analysis of the quadrature squeezing and photon statistics of the light produced by a non-degenerate parametric amplification has been made by a number of authors [1,6-8]. It has been shown theoretically [9-12] and subsequently confirmed experimentally [13,14] that parametric amplification produces a light that has a maximum of 50 % squeezing below the coherent state level.
On the other hand, Xiong et al. [15] have recently proposed a scheme for an entanglement based on a non-degenerate three-level laser when the three level atoms are injected at the lower level and the top and bottom levels are coupled by a strong coherent light. They have found that a non-degenerate three-level laser can generate light in entangled state employing the entanglement criteria for bipartite continuous-variable states [15].
Moreover, Tan et al. [16] have extended the work of Xiong et al. and examined the generation and evolution of the entangled light in the Wigner representation using the sufficient and necessary in separability criteria for a two-mode Gaussian state proposed by Dual et al. [15] and Simon [17]. Tesfa [18] has considered a similar system when the atomic coherence is induced by superposi-tion of atomic states and analyzed the entanglement at steady-state. Furthermore, Ooi [19] has studied the steady-state entanglement in a two-mode laser. More recently, Eyob [20] has studied continuous-variable entanglement in a non-degenerate three-level laser with a parametric amplifier.
Even though Einstein, along with Podolsky and Rosen, was first to recognize the criterion for analyzing global entanglement condition for a two-mode light beams [21-24], a significant number of works have not been devoted on spectrum of entanglement fluctuations and local entanglement condition for two-mode cavity light.
In this paper, we present new definitions of power spectrum, spectrum of intensity fluctuations, spectrum of quadrature fluctuations, and spectrum of entanglement fluctuations. Moreover, we also analyze the local quadrature squeezing and the local photon statistics for the two-mode cavity light.
In this section, we first obtain c-number Langevin equations with the aid of the master equation (Figure 1).
We then determine the solutions of the resulting differential equations. With the pump mode represented by a real and constant c-number, the process of non-degenerate parametric amplification can be described by the Hamiltonian.
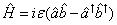 (1)
(1)
Where 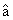 and
and 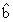 are respectively the annihilation operators for the signal and idler modes and ε = λμ, with λ being the coupling constant. Applying Equation 1 and taking into account the interaction of the signal-idler modes with a two-mode vacuum reservoir via a single-port mirror, the master equation for the cavity modes can be written as
are respectively the annihilation operators for the signal and idler modes and ε = λμ, with λ being the coupling constant. Applying Equation 1 and taking into account the interaction of the signal-idler modes with a two-mode vacuum reservoir via a single-port mirror, the master equation for the cavity modes can be written as
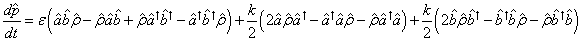 (2)
(2)
in which the cavity damping constant k is assumed to be the same for both the signal and idler modes. Now employing the commutation relations
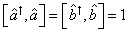 (3)
(3)
and
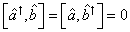 (4)
(4)
together with Equation 2, we readily obtain
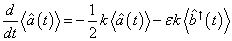 (5)
(5)
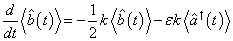 (6)
(6)
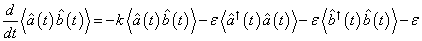 (7)
(7)
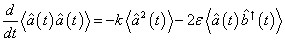 (8)
(8)
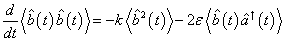 (9)
(9)
We note that the c-number equations corresponding to Equations 5, 6, 7, 8 and 9 are
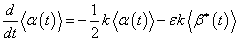 (10)
(10)
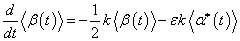 (11)
(11)
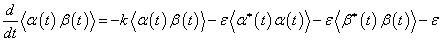 (12)
(12)
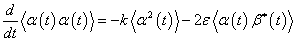 (13)
(13)
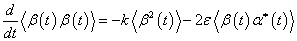 (14)
(14)
On the basis of Equations 10 and 11, one can write
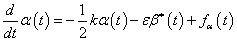 (15)
(15)
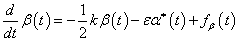 (16)
(16)
where fα(t) and fβ(t) are noise forces corresponding to the twomodes . Moreover, one can readily check that
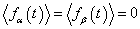 (17)
(17)
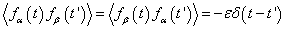 (18)
(18)
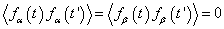 (19)
(19)
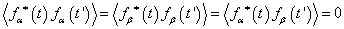 (20)
(20)
Applying Equation 15 along with the complex conjugate of Equation 16, we readily obtain
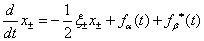 (21)
(21)
in which
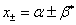 (22)
(22)
And
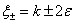 (23)
(23)
According to Equations 21 and 22, the equation of evolution of α- does not have a well behaved solution for k < 2ε. We then identify k = 2ε as a threshold condition. For 2ε < k, the solution of Equation 21 can be put in the form
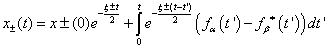 (24)
(24)
It then follows that
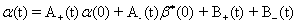 (25)
(25)
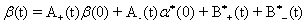 (26)
(26)
Where
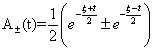 (27)
(27)
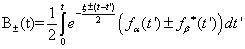 (28)
(28)
Power Spectrum
In nearly of two-mode light is is some variation about the central frequencerr We wish here to obtain the spectrum of the mean photon number, usually know as the power spectrum of a light modes represented by the operators c and c ? . We would like to mention that c and c ? can be cavity mode operators. We define the power spectrum of two-mode light with central frequency Ω0 by
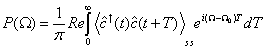 (29)
(29)
in which
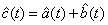 (30)
(30)
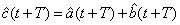 (31)
(31)
and Ω0= (ωa + ωb), with ωa and ωb being the central frequencies of the signal and idler modes and the power spectrum is Lorentzian centered at Ω = Ω0 as well as Ω′=-λ and Ω0=+λ are the lower and upper frequency limits with the band width of 2λ. Then the power spectrum is found to be
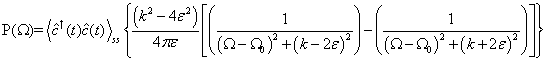 (32)
(32)
where
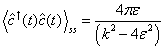 (33)
(33)
being the steady-state mean photon number of the signal-idler modes. Upon integrating both sides of Equation 32 over , we readily get
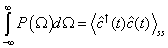 (34)
(34)
On the basis of Equation 34, we observe that P(Ω)dΩ represents the steady-state mean photon number for the signal-idler modes in the interval between Ω and Ω+dΩ. We thus realize that the steady-state local mean photon number in the interval between Ω′= -λ and Ω′= λ can be written as
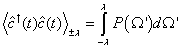 (35)
(35)
where Ω′ = Ω- Ω0. Therefore, using Equation 32 and the fact that
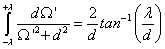 (36)
(36)
we readily obtain
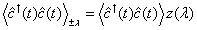 (37)
(37)
Where
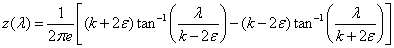 (38)
(38)
One can easily get from Figure 2 that z(0.5) = 0.9019, z(1) = 0.9496,
z(2) = 0.9713, and z(3) = 0.9815. Then combination of this results with Equation 37 yields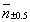 = 0.9019 n,
= 0.9019 n, 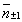 = 0.9496 n,
= 0.9496 n, 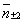 = 0.9713n and
= 0.9713n and 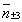 = 0.9815 n. We immediately see that a large part of the total mean photon number is confined in a relatively small frequency interval.
= 0.9815 n. We immediately see that a large part of the total mean photon number is confined in a relatively small frequency interval.
We seek to determine the local variance of the photon number in a given frequency interval employing the spectrum of intensity fluctuations. The spectrum of intensity fluctuations for a two-mode cavity light with central frequency Ω0 is expressible as
 (39)
(39)
Where
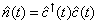 (40)
(40)
And
 (41)
(41)
Then which follows
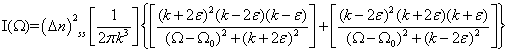 (42)
(42)
Where
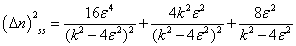 (43)
(43)
is the global photon-number variance of the signal-idler modes. Upon integrating both sides of Equation 42 over Ω, one easily obtains
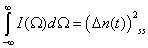 (44)
(44)
Moreover, on the basis of Equation 44, we observe that I(Ω)dΩ represents the steady-state variance of the photon number for the twomode cavity light in the interval between Ω and Ω+dΩ. We thus realize that the photon number variance for the cavity light in the interval between Ω′= -λ and Ω′= λ can be written as (Figure 3)
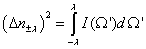 (45)
(45)
where Ω′ = Ω-Ω0. Therefore, employing Equations 36 and 42, we readily obtain
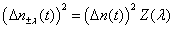 (46)
(46)
where
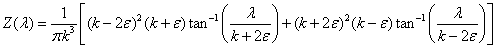 (47)
(47)
From the plot in Figure 3, we easily find z(0.5)=0.856, z(1)=0.932, z(2)=0.960, z(3)=0.974. Then combination of this results with Equation 46 yields (Δn±0.5)2=0.856 (Δn)2, (Δn±1)2=0.932 (Δn)2, (Δn±2)2=0.960 (Δn)2, (Δn±3)2=0.974(Δn)2. We immediately see that a large part of the photon number variance is confined in a relatively small frequency interval.
Here we seek to obtain the local quadrature squeezing of the signalidler modes employing the spectrum of quadrature fluctuations. We first define the spectrum of quadrature fluctuations for a given twomode cavity light with central frequency Ω0 by
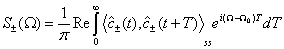 (48)
(48)
in which
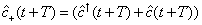 (49)
(49)
and
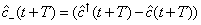 (50)
(50)
Then, the spectrum of the quadrature fluctuations for the signalidler modes is found to be
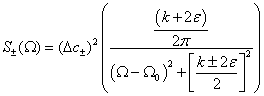 (51)
(51)
where
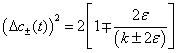
is the quadrature variance of the signal-idler modes at steady-state. We observe that the signalidler modes are in a squeezed state and the squeezing occurs in the plus quadrature. Upon integrating both sides of Equation 51 over, we get
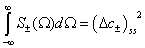 (53)
(53)
On the basis of Equation 53, we observe that S ± (Ω) dΩ is the quadrature variance of the two-mode cavity light in the interval between Ω and Ω + dΩ. Now the local quadrature variance in the interval Ω′= -λ and Ω′= λ can then be written as
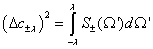 (54)
(54)
in which Ω′ = Ω-Ω0.
Furthermore, upon integrating Equation 51 in the interval between ω′= -λ and ω′= λ, using the relation described by Equation 36, we readily get
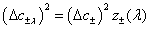 (55)
(55)
in which
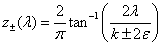 (56)
(56)
We easily obtain from Figure 4 that z(+5)=0.906, z(+15)=0.968, z(+25)=0.981, and z(+50)=0.990. Then combination of this results with Equation 55 yields (Δc±5)2=0.906 (Δc+)2, (Δc±5)2=0.968 (Δc+)2, (Δc±25)2=0.981 (Δc+)2, and (Δc±50)2=0.990 (Δc+)2. We immediately see that a large part of the quadrature variance of the signal-idler modes is confined in a relatively small frequency interval.
Moreover, in view of Equation 52 and 56, Equation 55 can be rewritten as
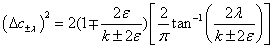 (57)
(57)
We note that the quadrature variance of the two-mode vacuum state in the interval between ω′= -λ and ω′= λ can be obtained by setting ε = 0 in Equation 57. We then get Figures 4 and 5.
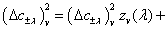 (58)
(58)
where
 (59)
(59)
and
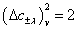 (60)
(60)
The plot in Figure 5 shows as λ increases, zv(λ) approaches to 1.
We next calculate the local quadrature squeezing of the signal-idler modes relative to the local quadrature variance of vacuum state. We then define the local quadrature squeezing of the twomode cavity light in the interval between Ω′= -λ and Ω′= λ by
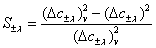 (61)
(61)
Then combination of Equations 57, 58 and 61 leads to
 (62)
(62)
We immediately see that the quadrature squeezing of the two-mode cavity light in a given frequency interval is not equal to that of the cavity light in the entire frequency interval. We see from the plot in Figure 6 that the maximum local quadrature squeezing is 75% and occurs in the ±0:01 frequency interval. In addition, we note that the local quadrature squeezing approaches to the global quadrature squeezing as λ increases. We also realize that as the quadrature squeezing increases, the mean photon number decreases.
In this section we seek to study the local entanglement fluctuations for a two-mode cavity light employing the entanglement spectrum. We first define the spectrum of entanglement fluctuations of the two EPRlike operators û and 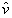 for a given two-mode cavity light with central frequency Ω0 by
for a given two-mode cavity light with central frequency Ω0 by
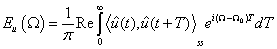 (63)
(63)
in which
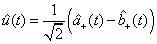 (64)
(64)
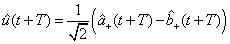 (65)
(65)
And
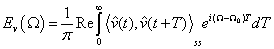 (66)
(66)
in which
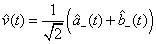 (67)
(67)
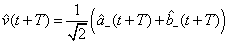 (68)
(68)
with 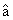 and
and 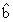 are the plus and minus quadrature operators of the signal and idler modes, respectively. Then we can readily find
are the plus and minus quadrature operators of the signal and idler modes, respectively. Then we can readily find
where
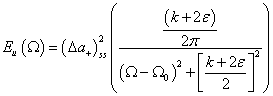 (69)
(69)
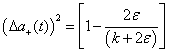 (70)
(70)
is the quadrature variance of the signal mode at steady-state. And
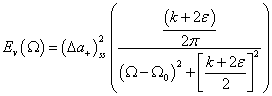 (71)
(71)
Where
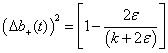 (72)
(72)
is the quadrature variance of the idler mode at steady-state. Therefore, the spectrum of entanglement fluctuations for the signalidler modes can be written as (Figure 7).
 (73)
(73)
Where(  we observe that the spectrum of entanglement fluctuation is a Lorentzian centered at Ω-Ω0 and with a half width of k. Upon integrating both sides of Equation 73 over Ω, we get
we observe that the spectrum of entanglement fluctuation is a Lorentzian centered at Ω-Ω0 and with a half width of k. Upon integrating both sides of Equation 73 over Ω, we get
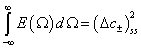 (74)
(74)
Thus we realize that the local entanglement fluctuations in the interval Ω′= -λ and Ω′= λ can then be written as
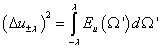 (75)
(75)
And
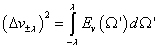 (76)
(76)
in which Ω′ = Ω-Ω0
Furthermore, upon integrating Equations 69 and 71 in the interval between Ω′= -λ and Ω′= λ we readily find
 (77)
(77)
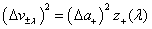 (78)
(78)
in which
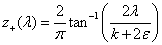 (79)
(79)
Hence on the basis of the criteria stated in [5], a two-mode cavity light is said to be locally entangled if the sum of the local variance of the two EPR-like operators û and  satisfies the inequality (Figure 8).
satisfies the inequality (Figure 8).
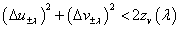 (80)
(80)
For instance, the sum of the local variance of the two EPR-like operators for the system under consideration to be
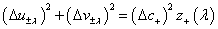 (81)
(81)
Moreover, the sum of the local variance of the two EPR-like operators, at steady-state and threshold, is found to be
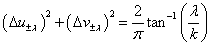 (82)
(82)
With the aid of Equation 80, we clearly see that a signal-idler
modes are locally entangled when the light operating at steady-state and threshold.
On the other hand, we can define the degree of local entanglement as in the form
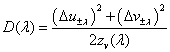 (83)
(83)
then it leads to
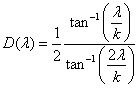 (84)
(84)
The plot in Figure 8 shows that 50% maximum degree of local entanglement is turned out be observed in the squeezed photons for the given frequency interval.
We have analyzed a slightly modified definitions for the power spectrum, the spectrum of intensity fluctuations, the spectrum of quadrature fluctuations, and the spectrum of entanglement fluctuations that holds true for a two-mode photon system. We have also presented a new definition for local entanglement fluctuations. In order to carry out our analysis, we considered a quantum system with a Gaussian variables with zero mean. It is found that the local mean photon number of the signal-idler light beams to be the sum of the local mean photon numbers of the constituent light beam. And the local photon number variance of the signal-idler light beams happens to be the sum of the local photon number variances of the separate light beam.
Furthermore, applying a slightly modified definition of the local quadrature variance, we have obtained that the local quadrature variance of the signal-idler modes to be the sum of the local quadrature variances of the individual light beams and the signal-idler light beams are in a squeezed state and the squeezing occurs in the plus quadrature. Moreover, the global quadrature squeezing turned out to be the average of the quadrature squeezing of the component light beams. Besides, our analysis shows that at steady state and at threshold, the signalidler modes have a maximum squeezing of 75% below the two-mode vacuum-states level in the given frequency interval. We have also clearly shown that two-mode light beams are locally entangled at steady-state and the entanglement turned out to be observed in the highly correlated squeezed photons with 50% degree of local entanglement.
To this end, we would like to mention that the predictions made in this paper concerning the local entanglement and local quadrature squeezing to be experimentally verified.