Journal of Physical Chemistry & Biophysics
Open Access
ISSN: 2161-0398
ISSN: 2161-0398
Research Article - (2016) Volume 6, Issue 4
Densities, viscosities, speed of sound and IR spectroscopy of binary mixtures of tert-butyl acetate (tBA) with isopropylbenzene, isobutylbenzene, methoxybenzene have been measured over the entire range of composition, at (298.15 and 308.15) K and at atmospheric pressure. From the experimental values of density, viscosity, speed of sound and IR spectroscopy the excess molar volumes (VE), deviations in viscosity (Δη) and deviation in isentropic compressibility (Δks) and stretching frequency () have been calculated. The excess molar volumes and deviations in isentropic compressibility with tBA are positive for the mixtures of isobutylbenzene and methoxybenzene with tBA and, negative for isopropylbenzene with tBA while deviations in viscosities are negative for the binary mixtures of isobutylbenzene, methoxybenzene and positive for isopropylbenzene. The excess molar volumes, deviations in viscosity and, deviations in isentropic compressibility have been fitted to the Redlich-Kister polynomial equation.
<Keywords: Benzene; Deviations in viscosity; Deviations in isentropic compressibility; Excess molar volumes; IR; Tert-butyl acetate
Studies on thermodynamic and transport properties of binary liquid mixtures provide information on the nature of interactions in the constituent binaries. Literature provides extensive data on the density and viscosity of liquid mixtures but a combined study of density, viscosity, speed of sound and IR study is quite scarce. In continuation of our earliest studies [1-5] with binary mixtures of esters with normal and branched alcohols, we now report the density, viscosity, speed of sound and spectroscopic studies of tert-butyl acetate (tBA) with isopropylbenzene, isobutylbenzene and methoxybenzene at 298.15 and 308.15K. The effect of chain length and chain branching of alkyl on the solute- solvent interactions were reported earlier [6]. The excess molar volumes were explained on the basis of strong dipole-induced dipole interaction between π- electron cloud of aromatic ring and carbonyl group of tBA. The excess molar volume depends with temperature. The interaction between esters and hydrocarbons were studied [7] for binary mixtures of butyl acetate with aromatic hydrocarbons. The electron withdrawing groups increases IR absorption frequency while electron donating groups lowers IR absorption frequency. In present paper we have studied the physico-chemical properties of the mixtures indicated above, in order to explain the strength and nature of the interactions between the components by deriving various thermodynamic parameters from volumetric, viscometric, acoustic properties of binary mixtures and spectroscopic study.
Experimental Procedure
Isopropylbenzene (Cumene) (Fluka, purity >99%), isobutylbenzene (SD Fine Chem., purity >99%), methoxybenzene (Anisole) (Sisco Research Lab Pvt Ltd., purity >99.5%), and tert-butyl acetate (tBA) (Spectrochem Pvt. Ltd, purity >99%) were used after single distillation. The purity of the solvents, after purification, was ascertained by comparing their densities, viscosities and speed of sound with the corresponding literature values at 298.15 K and 308.15 K (Table 1). Binary mixtures were prepared by mass in air tight stoppered glass bottles. The masses were recorded on an Adairdutt balance to an accuracy of ± 1 × 10-4 g. Care was taken to avoid evaporation and contamination during mixing. The estimated uncertainty in mole fraction was <1 × 10-4.
| Liquid | Tempt | r × 10-3 (kg.m-3) | η (mPa.s) | u (m.s-1) | |||
|---|---|---|---|---|---|---|---|
| K | Expt | Lit | Expt. | Lit | Expt. | Lit. | |
| tert-butyl acetate | 298.15 | 0.8611 | 0.86057 a | 0.683 | - | 1092 | 1092.76 b |
| 308.15 | 0.8494 | 0.84938 a | 0.596 | - | 1055 | 1049.71b | |
| isopropylbenzene | 298.15 | 0.8570 | 0.85752 c 0.8571 d |
0.735 | 0.739 d 0.731 c |
1330 | 1338 d |
| 308.15 | 0.8485 | 0.84924 c 0.84915 f |
0.656 | 0.636 c | 1280 | 1266.5 e 1264 k |
|
| isobutylbenzene | 298.15 | 0.8488 | 0.84891f | 0.979 | - | 1296 | 1296.7 e |
| 308.15 | 0.8404 | 0.84082 f | 0.844 | 0.848 k | 1260 | 1257.5 e 1252 k |
|
| methoxybenzene | 298.15 | 0.9892 | 0.9893 l 0.9889 g 0.98932 h 0.98915 j |
1.017 | 1.0023 g 1.017 j 0.991i |
1404 | 1410 g 1408.02 j |
| 308.15 | 0.9791 | 0.9788 I 0.9796 h |
0.890 | 0.8896 g | 1376 | - | |
aRef. [6]; bRef. [19]; cRef. [20]; dRef. [21]; eRef. [22]; fRef. [7]; gRef. [23]; hRef. [24]; iRef. [25]; jRef. [26]; kRef. [27]; lRef. [28].
Table 1: Comparison of experimental density, viscosity and speed of sound pure liquids with literature values at 298.15 and 308.15K.
Densities were determined by using a 15 cm3 bicapillary pycnometer as described earlier [8-10]. The pycnometer was calibrated using conductivity water with 0.99705 g.cm-3 as its density [11] at 298.15 K. The pycnometer filled with air bubble free experimental liquids was kept in a transparent walled water bath (maintained constant to ± 0.01 K) for (10 to 15) min to attain thermal equilibrium. The positions of the liquid levels in the two arms were recorded with the help of a traveling microscope, which could read to 0.01 mm. The estimated uncertainty of density measurements of solvent and binary mixtures was 0.0001 g.cm-3. At least three to four measurements were made which had an average deviation of ± 0.0001 g.cm-3.
The dynamic viscosities were measured using an Ubbelohde suspended level viscometer [9], calibrated with conductivity water. An electronic digital stop watch with readability of ± 0.01 s was used for the flow time measurements. At least three repetitions of each data reproducible to ± 0.05 s were obtained, and the results were averaged. The uncertainties in dynamic viscosities are of the order s 0.003 mPa.s. The speed of sound (u) were measured at a frequency of 2 MHz in these solutions through interferometric method (using Mittal’s F-81 model) at (298.15 and 308.15) K (± 0.05 K). The uncertainty in speed measurements is estimated to be ± 0.1%. The other experimental details are the same as reported earlier [4,12]. FTIR spectra of the above were recorded on FTIR spectrometer model Shimadzu 8400S PC.
Experimental values of densities ρ, viscosities η and speed of sound u of mixtures at (298.15 and 308.15) K are listed as a function of mole fraction in Table 2. The density values have been used to calculate excess molar volumes VE using the following equation
| x1 | ρ × 10-3 (kg. m-3) |
VE × 106 (m3.mol-1) |
η (mPa.s) |
?η (mPa.s) |
u (m.s-1) |
ks (TPa-1) |
?ks (TPa-1) |
|---|---|---|---|---|---|---|---|
| tBA (1)+isopropylbenzene (2) at 298.15K | |||||||
| 0.0000 | 0.8570 | 0.000 | 0.735 | 0.000 | 1330 | 660 | 0 |
| 0.0991 | 0.8574 | -0.001 | 0.732 | 0.002 | 1302 | 688 | -3 |
| 0.1972 | 0.8578 | -0.003 | 0.729 | 0.004 | 1276 | 716 | -6 |
| 0.2970 | 0.8583 | -0.019 | 0.724 | 0.004 | 1250 | 746 | -7 |
| 0.3962 | 0.8587 | -0.018 | 0.719 | 0.005 | 1225 | 776 | -8 |
| 0.4965 | 0.8591 | -0.017 | 0.713 | 0.004 | 1201 | 807 | -9 |
| 0.5963 | 0.8596 | -0.031 | 0.707 | 0.003 | 1177 | 840 | -7 |
| 0.6973 | 0.8600 | -0.028 | 0.701 | 0.002 | 1154 | 873 | -6 |
| 0.7976 | 0.8604 | -0.025 | 0.695 | 0.001 | 1132 | 907 | -3 |
| 0.8992 | 0.8608 | -0.020 | 0.689 | 0.001 | 1111 | 941 | -1 |
| 1.0000 | 0.8611 | 0.000 | 0.683 | 0.000 | 1092 | 974 | 0 |
| tBA (1)+isopropylbenzene (2) at 308.15K | |||||||
| 0.0000 | 0.8485 | 0.000 | 0.656 | 0.000 | 1280 | 719 | 0 |
| 0.0991 | 0.8486 | -0.002 | 0.652 | 0.002 | 1254 | 749 | -4 |
| 0.1972 | 0.8487 | -0.005 | 0.647 | 0.003 | 1230 | 779 | -7 |
| 0.2970 | 0.8488 | -0.006 | 0.642 | 0.004 | 1205 | 811 | -9 |
| 0.3962 | 0.8489 | -0.008 | 0.636 | 0.004 | 1181 | 845 | -8 |
| 0.4965 | 0.8491 | -0.026 | 0.629 | 0.003 | 1157 | 880 | -7 |
| 0.5963 | 0.8492 | -0.028 | 0.623 | 0.003 | 1135 | 914 | -7 |
| 0.6973 | 0.8493 | -0.029 | 0.616 | 0.002 | 1114 | 949 | -6 |
| 0.7976 | 0.8493 | -0.014 | 0.609 | 0.001 | 1093 | 986 | -3 |
| 0.8992 | 0.8494 | -0.015 | 0.602 | 0.000 | 1074 | 1021 | -3 |
| 1.0000 | 0.8494 | 0.000 | 0.596 | 0.000 | 1055 | 1058 | 0 |
| x1 | ρ × 10-3 (kg. m-3) |
VE × 106 (m3.mol-1) |
η (mPa.s) |
?η (mPa.s) |
u (m.s-1) |
ks (TPa-1) |
?ks (TPa-1) |
|---|---|---|---|---|---|---|---|
| tBA (1)+isobutylbenzene (2) at 298.15K | |||||||
| 0.0000 | 0.8488 | 0.000 | 0.979 | 0.000 | 1296 | 701 | 0 |
| 0.0980 | 0.8496 | 0.045 | 0.948 | -0.002 | 1273 | 726 | -2 |
| 0.1980 | 0.8501 | 0.152 | 0.916 | -0.004 | 1250 | 753 | -2 |
| 0.2973 | 0.8506 | 0.260 | 0.883 | -0.008 | 1228 | 780 | -2 |
| 0.3981 | 0.8511 | 0.374 | 0.850 | -0.011 | 1207 | 807 | -3 |
| 0.4980 | 0.8517 | 0.471 | 0.818 | -0.014 | 1186 | 835 | -2 |
| 0.5972 | 0.8526 | 0.519 | 0.787 | -0.015 | 1167 | 861 | -3 |
| 0.6974 | 0.8539 | 0.507 | 0.758 | -0.015 | 1148 | 889 | -2 |
| 0.7983 | 0.8556 | 0.439 | 0.730 | -0.013 | 1129 | 917 | -2 |
| 0.8980 | 0.8579 | 0.281 | 0.705 | -0.008 | 1110 | 946 | 0 |
| 1.0000 | 0.8611 | 0.000 | 0.683 | 0.000 | 1092 | 974 | 0 |
| tBA (1)+isobutylbenzene (2) at 308.15K | |||||||
| 0.0000 | 0.8404 | 0.000 | 0.844 | 0.000 | 1260 | 750 | 0 |
| 0.0980 | 0.8410 | 0.031 | 0.819 | -0.001 | 1237 | 777 | -3 |
| 0.1980 | 0.8414 | 0.105 | 0.792 | -0.003 | 1214 | 806 | -5 |
| 0.2973 | 0.8417 | 0.199 | 0.765 | -0.005 | 1192 | 836 | -6 |
| 0.3981 | 0.8421 | 0.278 | 0.737 | -0.008 | 1171 | 866 | -7 |
| 0.4980 | 0.8425 | 0.358 | 0.710 | -0.010 | 1151 | 896 | -7 |
| 0.5972 | 0.8431 | 0.404 | 0.684 | -0.012 | 1131 | 927 | -7 |
| 0.6974 | 0.8440 | 0.404 | 0.600 | -0.071 | 1111 | 960 | -5 |
| 0.7983 | 0.8453 | 0.343 | 0.636 | -0.010 | 1092 | 992 | -4 |
| 0.8980 | 0.8470 | 0.221 | 0.615 | -0.006 | 1074 | 1024 | -3 |
| 1.0000 | 0.8494 | 0.000 | 0.596 | 0.000 | 1055 | 1058 | 0 |
| x1 | ρ × 10-3 (kg. m-3) |
VE × 106 (m3.mol-1) |
η (mPa.s) |
?η (mPa.s) |
u (m.s-1) |
ks (TPa-1) |
?ks (TPa-1) |
|---|---|---|---|---|---|---|---|
| tBA (1)+methoxybenzene (2) at 298.15K | |||||||
| 0.0000 | 0.9892 | 0.000 | 1.017 | 0.000 | 1404 | 513 | 0 |
| 0.0974 | 0.9736 | 0.063 | 0.966 | -0.018 | 1339 | 573 | 15 |
| 0.1983 | 0.9581 | 0.137 | 0.921 | -0.030 | 1284 | 633 | 29 |
| 0.2988 | 0.9433 | 0.218 | 0.883 | -0.034 | 1240 | 689 | 38 |
| 0.3987 | 0.9293 | 0.290 | 0.850 | -0.034 | 1203 | 744 | 47 |
| 0.4982 | 0.9161 | 0.342 | 0.820 | -0.031 | 1173 | 793 | 50 |
| 0.5990 | 0.9034 | 0.380 | 0.792 | -0.025 | 1149 | 838 | 49 |
| 0.6992 | 0.8916 | 0.373 | 0.766 | -0.017 | 1128 | 881 | 46 |
| 0.7984 | 0.8807 | 0.318 | 0.739 | -0.011 | 1113 | 917 | 36 |
| 0.8975 | 0.8707 | 0.190 | 0.713 | -0.004 | 1100 | 949 | 22 |
| 1.0000 | 0.8611 | 0.000 | 0.683 | 0.000 | 1092 | 974 | 0 |
| tBA (1)+methoxybenzene (2) at 308.15K | |||||||
| 0.0000 | 0.9791 | 0.000 | 0.890 | 0.000 | 1376 | 539 | 0 |
| 0.0974 | 0.9635 | 0.037 | 0.847 | -0.014 | 1309 | 606 | 16 |
| 0.1983 | 0.9479 | 0.096 | 0.810 | -0.022 | 1255 | 670 | 28 |
| 0.2988 | 0.9331 | 0.153 | 0.776 | -0.026 | 1211 | 731 | 37 |
| 0.3987 | 0.9191 | 0.201 | 0.747 | -0.026 | 1174 | 789 | 43 |
| 0.4982 | 0.9058 | 0.243 | 0.720 | -0.024 | 1144 | 844 | 46 |
| 0.5990 | 0.8930 | 0.271 | 0.695 | -0.019 | 1119 | 894 | 44 |
| 0.6992 | 0.8811 | 0.255 | 0.671 | -0.013 | 1098 | 941 | 39 |
| 0.7984 | 0.8699 | 0.222 | 0.647 | -0.008 | 1081 | 984 | 31 |
| 0.8975 | 0.8594 | 0.149 | 0.623 | -0.003 | 1067 | 1022 | 17 |
| 1.0000 | 0.8494 | 0.000 | 0.596 | 0.000 | 1055 | 1058 | 0 |
Table 2: Density (ρ), viscosity (η), excess molar volume (VE), deviation in viscosity (Δη), speed of Sound (u), isentropic compressibility (κs) and deviation in isentropic compressibility (Δκs).
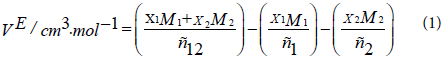
where ρ12 is the density of the mixture and x1, M1, ρ1, and x2, M2, ρ2 are the mole fraction, the molecular weight, and the density of pure components 1 and 2, respectively.
The viscosity deviations Δη were calculated using
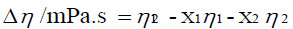 (2)
(2)
where η12 is the viscosity of the mixture and x1, x2 and η1, η2 are the mole fraction and the viscosity of pure components 1 and 2, respectively.
The speed of sound u was used to calculate the isentropic compressibility κs by the equation
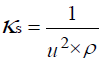 (3)
(3)
The deviation from isentropic compressibility, (Δκs), was obtained using the relation,
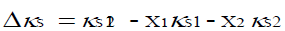 (4)
(4)
where κs12 is the experimental isentropic compressibility of the mixture, x1, x2 and κs1, κs2 are the mole fraction and isentropic compressibility of pure components.
The excess molar volumes and deviations in viscosity and isentropic compressibility were fitted to Redlich Kister [13] equation of the type
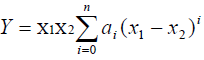 (5)
(5)
Where Y is either VE, or Δη, or Δκs, and n is the degree of polynomial. Coefficients ai were obtained by fitting eq 5 to experimental results using a least-squares regression method. In each case, the optimum number of coefficients is ascertained from an examination of the variation in standard deviation σ.
σ was calculated using the relation
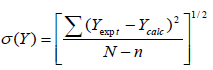 (6)
(6)
where N is the number of data points and n is the number of coefficients. The calculated values of the coefficients ai along with the standard deviations σ are given in Table 3. The variation of VE with the mole fraction x1 of tBA for isopropylbenzene, isobutylbenzene and methoxybenzene is represented in Figure 1 at 298.15K. The values of VE are positive for the binary mixtures of tBA with isobutylbenzene and methoxybenzene while these are negative for the binary mixtures of tBA with isopropylbenzene. If the interaction between molecules of two mixed components is weaker than in the pure component, the excess volume will be positive. This usually occurs when one component has polar groups and the other a non-polar or weakly polar behaviour. tBA is weakly polar and aromatic hydrocarbons are nearly non-polar. When the pure compounds are mixed, the non-polar hydrocarbon molecules intersperse among the tBA molecules resulting in a decreased interaction among the dipoles of the acetate and the destructions of dispersive interactions among benzenic rings. The new interaction among unalike molecules is less strong and produces expansion [7].
| Standard deviations | T/K | a0 | a1 | a2 | a3 | a4 | σ | ||
|---|---|---|---|---|---|---|---|---|---|
| tBA (1)+isopropylbenzene (2) | |||||||||
| VE/(cm3.mol-1) | 298.15 | -0.0931 | -0.1154 | -0.0287 | 0.0042 | ||||
| 308.15 | -0.0804 | -0.0866 | -0.0028 | 0.005 | |||||
| ?η /(mPa.s) | 298.15 | 0.0162 | -0.0197 | -0.0082 | 0.0187 | 0.0145 | 0.0004 | ||
| 308.15 | 0.0141 | -0.0127 | -0.0043 | 0.0003 | |||||
| ?ks /(Tpa-1) | 298.15 | -33.5391 | 13.6785 | 17.0287 | 0 | 0 | 0.4448 | ||
| 308.15 | -30.4397 | 13.0323 | -11.732 | 0 | 0 | 0.707 | |||
| tBA (1)+isobutylbenzene (2) | |||||||||
| VE/(cm3.mol-1) | 298.15 | 1.8709 | 1.3876 | -0.1099 | 0.3165 | 0.0052 | |||
| 308.15 | 1.4375 | 1.2575 | -0.0811 | 0.0039 | |||||
| ?η /(mPa.s) | 298.15 | -0.0547 | -0.0423 | 0.0005 | 0.0003 | ||||
| 308.15 | -0.089 | -0.0818 | 0.0652 | 0.0186 | |||||
| ?ks /(Tpa-1) | 298.15 | -10.59 | -8.0089 | -1.5468 | 33.0352 | 0.5755 | |||
| 308.15 | -28.7762 | 7.8474 | 16.3418 | -10.7753 | -37.0481 | 0.3528 | |||
| tBA (1)+methoxybenzene (2) | |||||||||
| VE/(cm3.mol-1) | 298.15 | 1.3823 | 0.9712 | 0.2266 | -0.1930 | -0.3255 | 0.0032 | ||
| 308.15 | 0.9717 | 0.5746 | 0.0767 | 0.2622 | 0.0053 | ||||
| ?η /(mPa.s) | 298.15 | -0.123 | 0.1006 | -0.0035 | 0.0005 | ||||
| 308.15 | -0.0935 | 0.0772 | -0.0024 | 0.0004 | |||||
| ?ks /(Tpa-1) | 298.15 | 199.596 | 40.0968 | 8.6513 | 0 | 0 | 0.6392 | ||
| 308.15 | 181.8703 | 6.8637 | 3.1048 | 0 | 0 | 0.5049 | |||
Table 3: Parameters and standard deviation σ of equations (5) and (6) for tBA + isopropylbenzene, + isobutylbenzene, + methoxybenzene at temp. 298.15 and 308.15 K.
Figure 2 depicts the variation of Δη with the mole fraction x1 of tBA. The Δη values are positive for isopropylbenzene and negative for isobutylbenzene and methoxybenzene systems. The methoxybenzene is more negative than isobutylbenzene.
The viscosities of binary mixtures are fitted to Grunberg and Nissan [14] viscosity model.
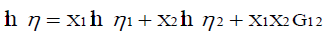 (7)
(7)
where G12 is an interaction parameter which is a function of the components 1 and 2 as well as temperature. Table 4 includes the different parameters for Grunberg - Nissan, Hind et al., Heric - Brewer and percentage standard deviations.
| System | T/K | G12 | σ | H12 | σ | Heric- Brewer α12α21 |
σ | |
|---|---|---|---|---|---|---|---|---|
| tBA (1) + isopropylbenzene (2) |
298.15 | 0.025 | 0.17 | 0.717 | 0.17 | 0.026 | -0.017 | 0.08 |
| 308.15 | 0.024 | 0.10 | 0.631 | 0.08 | 0.024 | -0.020 | 0.05 | |
| tBA (1) + isobutylbenzene (2) |
298.15 | -0.003 | 0.44 | 0.804 | 0.41 | 0.021 | -0.044 | 0.07 |
| 308.15 | -0.056 | 2.33 | 0.681 | 2.25 | -0.028 | -0.120 | 2.06 | |
| tBA (1) + methoxybenzene (2) |
298.15 | -0.066 | 0.85 | 0.788 | 1.01 | -0.032 | 0.107 | 0.08 |
| 308.15 | -0.045 | 0.48 | 0.695 | 0.67 | -0.014 | 0.094 | 0.06 | |
Table 4: Interaction parameters of Grunberg-Nissan, Hind et al., Heric-Brewer model and standard deviations σ at temp. 298.15 and 308.15 K.
Hind et al. [15] suggested an equation for the viscosity of binary liquid mixtures as
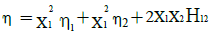 (8)
(8)
where H12 is an interaction parameter and is attribute to unlike pair interactions.
The two parameter Heric and Brewer [16] equation is of the form
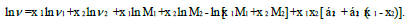 (9)
(9)
where M1 and M2 are molecular weights of components 1 and 2 and α12, α21 are interaction parameters.
The correlating ability of equations (7) to (9) was tested by calculating the percentage standard deviation (σ %) between the experimental and calculated viscosity as
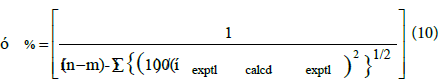
where n represents the number of experimental points and m represents the number of coefficients. From Table 4, it is seen that the values of G12 are positive for the binary mixtures of tBA with isopropylbenzene and negative for methoxybenzene. Also for isobutylbenzene G12 negative at 298.15K and positive at 308.15K. The values of H12 are positive for all the binary mixtures while α12 is positive for isopropylbenzene and isobutylbenzene and for methoxybenzene it is negative at 298.15K and positive at 308.15K. Also α21 is negative for isopropylbenzene and isobutylbenzene and positive for methoxybenzene.
| x1 | nC=O | nC-H | nC=C | nC-O |
|---|---|---|---|---|
| tBA(x1)+isopropylbenzene (1-x1) | ||||
| 0.0 | - | 2955(s) and many peaks within 2886 to 3023 | 1466 (m) | - |
| 0.4 | 1736 (s) | 2963 (s) 3053 (shoulder) |
1452 (m) 1373 (m) |
1262 (s) 1169 (s) |
| 0.5 | 1734 (s) | 2957 (s) | 1468 (m) 1381 (m) |
1263 (s) 1167 (s) |
| 0.6 | 1734 (s) | 2963 (m) | 1464 (m) 1373 (m) |
1262 (s) 1169 (s) |
| 0.7 | 1732 (s) | 2961 (m) | 1470 (m) 1377 (m) |
1263 (s) 1165 (s) |
| 0.8 | 1736 (s) | 2974 (m) and many peaks within 2882 to 3065 | 1458 (m) 1368 (m) |
1262 (s) 1171 (s) |
| 1.0 | 1732 (s) | 2982 (m) | - | 1262 (s) 1169 (s) |
| tBA(x1) + isobutylbenzene (1-x1) | ||||
| 0.0 | - | 2957 (s) and many Shoulders 2878 to 3071 |
1458 (m) 1377 (m) |
- |
| 0.4 | 1732 (s) | 2947 (s) 3038 (shoulder) |
1464 (m) 1373 (m) |
1260 (s) 1169 (s) |
| 0.5 | 1732 (s) | 2947 (s) 3042 (shoulder) |
1462 (m) 1375 (m) |
1260 (s) 1165 (s) |
| 0.6 | 1736 (s) | 2967 (m) and many Shoulders 2876 to 3065 |
1460 (m) 1370 (s) |
1260 (s) 1169 (s) |
| 0.7 | 1732 (s) | 2955 (s) 2920(shoulder) 2874(shoulder) |
1460 (m) 1377 (m) |
1263 (s) 1171 (s) |
| 1.0 | 1732 (s) | 2982 (m) | - | 1262 (s) 1169 (s) |
| x1 | nC=O | nC-H | nC=C | nC-O |
|---|---|---|---|---|
| tBA(x1)+methoxybenzene (1-x1) | ||||
| 0.0 | - | 2945 (m) 2837 (m) 3052 (m) |
1483 (s) 1593 (s) |
- |
| 0.4 | 1728 (s) | 2967 (m) | 1478 (s) 1591 (m) |
1263 (s) 1169 (m) 1040 (m) |
| 0.5 | 1728 (s) | 2969 (m) | 1476 (m) 1593 (m) |
1262 (s) 1169 (m) 1038 (m) |
| 0.6 | 1728 (s) | 2971 (m) | 1478 (m) 1594 (m) |
1262 (s) 1169 (m) 1038 (m) |
| 0.7 | 1730 (s) | 2980 (m) 2859 (shoulder) 3050 (shoulder) |
1481 (m) 1595 (m) |
1260 (s) 1169 (m) 1032 (m) |
| 0.8 | 1732 (s) | 2980 (m) | 1478 (m) 1601 (m) |
1260 (s) 1169 (m) 1030 (m) |
| 1.0 | 1732 (s) | 2982 (m) | - | 1262 (s) 1169 (s) 1028 (m) |
Table 5: FTIR stretching frequency (cm-1) of tBA(x1) and isopropylbenzene, isobutylbenzene, methoxybenzene (1-x1).
The variation of Δκs with mole fraction of tBA, x1, is represented in Figure 3. The values of Δκs for mixtures of tBA with isobutylbenzene and methoxybenzene are positive and negative for isopropylbenzene. The trend is observation is methoxybenzene more positive than isobutylbenzene.
Kiyohara and Benson [17] have suggested that ΔKs is the resultant of several opposing effects. The positive values may be attributed to the size of these molecules that allow relative molecular interactions between acetate molecules [18]. The trend signify decreasing dipoledipole interactions due to decreasing proton donating abilities with increasing chain length of aromatic hydrocarbons. From Table 5, the νC=O of tBA+isopropylbenzene decreased slightly from x1=0.4 onwards. Irregular trend in νC=C and alkyl νC-H is observed. There is no drastic change in νC-O stretching frequencies. From observation of νC=C of system it is concluded that the magnitude of interaction are more for tBA+isopropylbenzene.
The νC=O of TBA+Isobutylbenzene remains same within x1=0.4- 0.7 except at x1=0.6. Trend of νC-H was irregular with remarkable changes. νC=C increased from x1=0.0 to 0.4 and decreased x1=0.5 onwards. There are equal intense bands for νC-O in x1=0.4-0.6. Presence of extra CH2 group in isobutylbenzene as compared to isopropyl benzene affects νC=C and νC-H. More changes in νC=C are observed in isobutylbenzene. The νC=O almost remain same for tBA+methoxybenzene from x1=0.4 to 0.6 and then slightly increases. νC-H sharply increased from x1=0.4 onwards. An irregular trend in νC=C is observed for this system. The aromatic νC-O decreased with increase in x1. This suggests dipole-induced dipole interaction between carbonyl of tBA and aryl C-O.
The excess molar volumes and deviations in isentropic compressibility with tBA are positive for the mixtures of isobutylbenzene and methoxybenzene with tBA and, negative for isopropylbenzene with tBA while deviations in viscosities are negative for the binary mixtures of isobutylbenzene, methoxybenzene and positive for isopropylbenzene. The excess molar volumes, deviations in viscosity and, deviations in isentropic compressibility have been fitted to the Redlich-Kister polynomial equation. In FTIR spectra, presence of extra CH2 group in isobutylbenzene as compare to isopropylbenzene affects νC = C and νC-H. More changes in νC=C are observed in isobutylbenzene. The aromatic νC-O decreased with increase in x1. This suggests dipole-induced dipole interaction between carbonyl of tBA and aryl C-O.
Authors thanks to Mehadi Hasan, Former Head, PG Department of Chemistry, MSG Arts, Science and Commerce College, Malegaon Campus, Maharashtra, India for his kind co-operation.